Geometric Properties of an Isosceles Right Triangle
Aim: To check the accuracy of the geometric properties calculation for a rod cross-section in the form of an isosceles right triangle.
Name of a file with the initial data: Triangle90.cns
Formulation: Check the accuracy of the torsional geometric properties calculation for a rod cross-section in the form of an isosceles right triangle.
References: Galerkin B.G., Torsion of a Triangular Prism, Izv. Akad. Nauk. VI series, 13:1 (1919), 111–118
Polya G., Szego G., Isoperimetric Inequalities in Mathematical Physics, — M., Fizmatgiz, 1962.
Initial data:
| a = 40 cm | - leg length of the triangle. |
Design model: The design model is formed by triangulation (the number of triangles ≈ 3000) on the basis of a model of the external contour. The external contour is an isosceles right triangle. The number of vertices of the contour in a model is 3.
Results Obtained in Consul
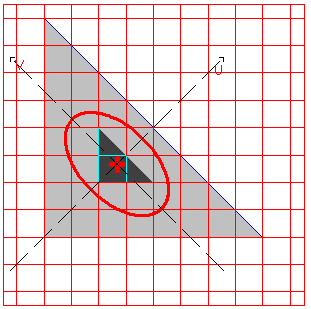
Design model, coordinate and principal axes, center of mass, ellipse of inertia, core of the section
Comparison of results:
|
Parameter |
Theory |
CONSUL |
Deviation, % |
|---|---|---|---|
|
Torsional moment of inertia, It cm4 |
66816 |
66251,348 |
0,845 |
Notes: Geometric properties can be determined analytically by the following formulas:
\[ I_{t} =a^{4}\left[ {\frac{1}{12}-\frac{16}{\pi^{5}}\sum\limits_{n=1}^\infty {\frac{1}{(2n-1)^{5}}\coth \left( {\frac{\pi (2n-1)}{2}} \right)} } \right]\approx 0.0261\cdot a^{4}. \]