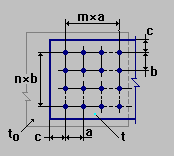
Analysis of an Overlapping Bolted Connection of Steel Sheets with Ordinary Bolts

Objective: Check the mode for calculating bolted connections

Task: Check an overlapping connection of 500х12 mm sheets with ordinary bolts from steel grade С245 for a shear force.

Source: Steel Structures: Student Handbook / [Kudishin U.I., Belenya E.I., Ignatieva V.S and others] - 13-th ed. rev. - M.: Publishing Center "Academy", 2011. p. 165.

Compliance with the codes: SNiP II-23-81*, SP 16.13330, DBN B.2.6-163:2010.

Initial data file:

2.1 SNiP.sav;	2.1 SP2011.sav;
report — Kristall 2.1 SNiP.doc	report — Kristall 2.1 SP2011.doc

 

Initial data from the source:

N = 1000 kN	Shear force;
Rbp = 450 MPa	Steel grade C245; Thickness of plates: two external – 8 mm, internal – 12 mm;
Rbs = 200 MPa	Bolts of 5.8 strength class and C accuracy class; Diameter of bolts 20 mm, diameter of holes 23 mm;
γc = 1	Service factor  of the structure;
γb = 0,9	Service factor  of the bolted connection.

 

KRISTALL initial data:
Steel: C245

Group of structures according to Annex C of SP 16.13330.2011 2

Importance factor	1
Service factor	1
Service factor  of members to be joined	1
Design shear strength of bolts Rbs	20897,044 T/m2
Design bearing strength of bolt elements Rbp	49541,284 T/m2

 

Type:	Bolts:	Parameters:
	Diameter of bolts 20 mm Diameter of holes 23 mm Class of bolts 5.8 Accuracy class B or C	m = 5 n = 1 a = 60 mm b = 60 mm c = 50 mm t = 8 mm t0 = 12 mm

Internal forces and moments:

N = 101,937 T
M_y = 0 T*m
Q_z = 0 T

 

Manual calculation (SP 16.13330.2011):

1. Design shear resistance of the bolts was calculated as follows
R_bs = 0.41R_bun = 0.41 × 500 = 205  MPa (see table 5).

2. Design bearing resistance of the bolts was calculated as follows R_bp = 1.35R_u = 1.35 × 360 = 486 MPa (see table 5).

3. Shear strength of the bolts was calculated according to the following formula:

\[ N_{bs} =R_{bs} A_{b} n_{s} \gamma_{b} \gamma_{c} =205\times 10^{3}\times 3,14\times 10^{-4}\times 2\times 1,0\times 0,9=115,866 \quad kN. \]

4. Bearing strength of the bolts was calculated according to the following formula:

\[ N_{bp} =R_{bp} D\left( {\sum\limits_i {t_{i} } } \right)_{\min } \gamma_{b} \gamma_{c} =486\times 10^{3}\times 20\times 12\times 10^{-6}\times 1,0\times 0.9=104.976 \quad kN. \]

 

Manual calculation (SNiP II-23-81*):

1. Design shear resistance of the bolts was calculated as follows R_bs = 0.4R_bun = 0.4 × 500 = 200 MPa (see table 5*).

2. Design bearing resistance of the bolts was taken as (see table 5*):

\[ R_{bp} =\left( {0,6+340\frac{R_{un} }{E}} \right)R_{un} =\left( {0,6+340\cdot \frac{370}{2,06\cdot 10^{5}}} \right)\cdot 370=447,95 \quad MPa. \]

3. Shear strength of the bolts was calculated according to the following formula:

\[ N_{bs} =R_{bs} A_{b} n_{s} \gamma_{b} \gamma_{c} =200\times 10^{3}\times 3,14\times 10^{-4}\times 2\times 1,0\times 0,9=113,04 \quad kN. \]

4. Bearing strength of the bolts was calculated according to the following formula:

\[ N_{bp} =R_{bp} D\left( {\sum\limits_i {t_{i} } } \right)_{\min } \gamma_{b} \gamma_{c} =447,95\times 10^{3}\times 20\times 12\times 10^{-6}\times 1,0\times 0,9=96,7575 \quad kN. \]

Comparison of solutions:

Factor	Codes	Shear strength	Bearing strength
Manual calculation	SP 16.13330.2011	1000/(12*115,866) = 0,719	1000/(12*104,976) = 0,794
KRISTALL	SP 16.13330.2011	0,719	0,794
Deviation from the manual calculation, %		0,0	0,0
Manual calculation	SNiP II-23-81*	1000/(12*113,04) = 0,737	1000/(12*96,7575) = 0,861
KRISTALL	SNiP II-23-81*	0,737	0,865
Deviation from the manual calculation, %		0,0	0,5
Source	SNiP II-23-81*	0,737	0,857