Plane Truss Subjected to a Concentrated Force

Objective: Determination of the stress-strain state of a plane truss subjected to a concentrated force.

Initial data file: SSLL09_v11.3.spr

Problem formulation: The plane truss consists of two inclined downward bars of the same length and rigidity of the cross-section arranged symmetrically with respect to the vertical axis, connected by hinges in the common node (point C) and simply supported at the opposite nodes (points A and B). A vertical concentrated force F is applied in the common node of the truss bars. Determine the vertical displacement of the common node of the truss bars Z and longitudinal forces in the truss bars N.

References: S. Timoshenko, Resistance des materiaux, t.1, Bruxelles, Edition Polytechnique Beranger, 1963, p. 10.

Initial data:

E = 2.1·1011  Pa	- elastic modulus of truss bars;
l = 4.5 m	- length of truss bars;
θ = 30º	- inclination angle of the bars to the horizon;
A = 3.0·10-4 m2	- cross-sectional area of the bars;
F = 2.1·104 N	- value of the vertical concentrated force.

 

Finite element model: Design model – plane hinged bar system, 2 bar elements of type 10. Boundary conditions are provided by imposing constraints in the directions of the degrees of freedom X, Z for pinned support nodes. Number of nodes in the design model – 3.

 

Results in SCAD

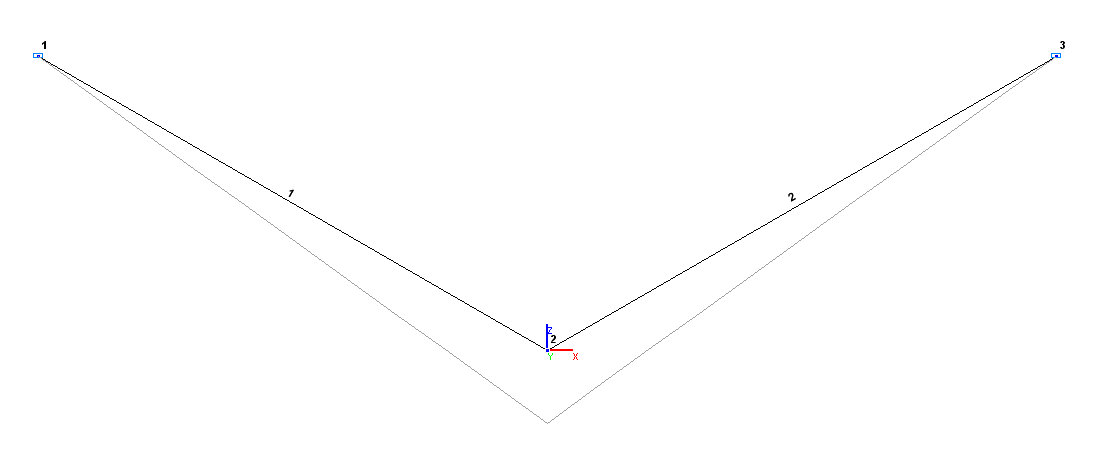
Design and deformed models

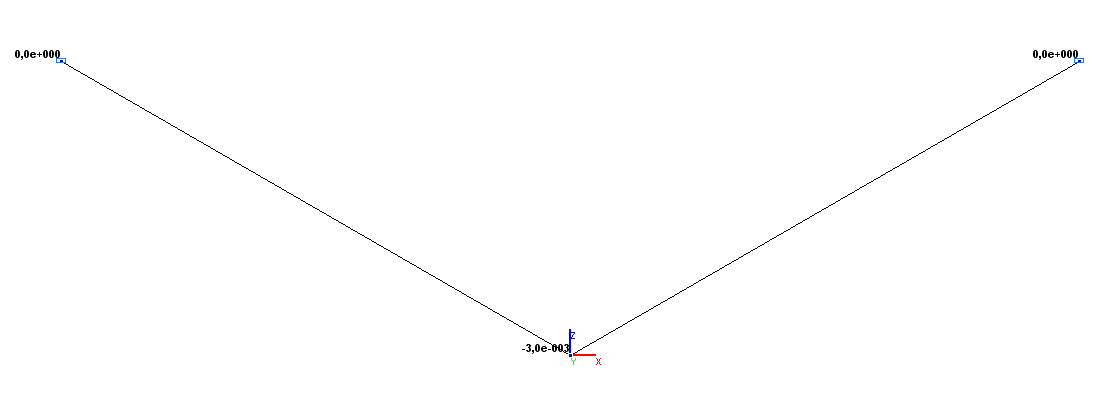
Values of vertical displacements Z (m)

Values of longitudinal forces N (N)

Comparison of solutions:

Parameter	Theory	SCAD	Deviations, %
Vertical displacement Z (point C), m	-3.0000·10-3	-3.0000·10-3	0.00
Longitudinal force N (bar AC), N	21000.0	21000.0	0.00
Longitudinal force N (bar BC), N	21000.0	21000.0	0.00

Notes: In the analytical solution, the vertical displacement of the common node of the truss bars Z and longitudinal forces in the truss bars N are determined according to the following formulas:

\[ Z=\frac{F\cdot L}{2\cdot E\cdot A\cdot \sin^{2}\left( \theta \right)}; \]

\[ N=\frac{F}{2\cdot \sin \left( \theta \right)}. \]