Stability of a Rectangular Simply Supported Plate with Longitudinal Stiffeners Uniformly Compressed in the Longitudinal Direction (Model 2)
Objective: Determination of the critical value of the compressive forces uniformly distributed along two opposite transverse sides of a rectangular simply supported plate reinforced by longitudinal stiffeners corresponding to the moment of its buckling.
Initial data files:
|
File name |
Description |
|---|---|
|
Design model with the ratios of the sides of the plate a/b = 1.0 |
|
|
Design model with the ratios of the sides of the plate a/b = 4.0 |
Problem formulation: The rectangular simply supported plate reinforced by longitudinal stiffeners is subjected to the action of compressive forces σ, uniformly distributed along two opposite transverse sides. Determine the critical value of the compressive forces σcr, corresponding to the moment of buckling of the rectangular reinforced plate taking into account the following assumptions made when deriving the analytical solution:
- The stiffeners are symmetric with respect to the midplane of the reinforced plate;
- Torsional stiffness of the stiffeners is not taken into account
- The stiffeners and the plate are subjected to the uniform compression
References: S. P. Timoshenko, Stability of Bars, Plates and Shells, Moscow, Nauka 1971, p. 507.
A.S. Volmir. Stability of Deformable Systems, Moscow, Nauka, 1967, p. 377.
Initial data:
| a = 0.6; 2.4 m | - side of the rectangular plate free from forces (along the X axis of the global coordinate system); |
| b = 0.6 m | - side of the rectangular plate subjected to the compressive forces (along the Y axis of the global coordinate system); |
| h = 0.01 m | - thickness of the rectangular plate; |
| F = 0.01∙0.03 = 3∙10-4 m2 | - cross-sectional area of the stiffeners; |
| I = 0.01∙0.033/12 = 2.25∙10-8 m4 | - cross-sectional moment of inertia of the stiffeners; |
| s = 3 | - number of stiffeners arranged uniformly along the width of the plate; |
| E = 2.0·108 кН/м2 | - elastic modulus of the material of the plate and stiffeners; |
| ν = 0.3 | - Poisson’s ratio; |
| σ = 1.0·105 кН/м2 | - initial value of the compressive forces. |
Finite element model: Design model – general type system. Two design models with the ratios of the sides of the plate a/b = 1.0; 4.0 are considered. The plate is modeled by eight-node shell elements of type 50. The spacing of the finite element mesh along the sides of the plate (along the X and Y axes of the global coordinate system) is 0.075 m. Number of plate elements in the models – 64; 256. The stiffeners are modeled by eight-node shell elements of type 50. The spacing of the finite element mesh along the longitudinal axes of the stiffeners (along the X1 axes of the local coordinate systems) is 0.075 m, and along the height of the stiffeners (along the Y1 axes of the local coordinate systems) is 0.015 m. Number of stiffener elements in the models – 48; 192. Boundary conditions are provided by imposing constraints on the nodes of the support contour of the plate in the direction of the degree of freedom Z. The load uniformly distributed along the line on the plate and on the stiffeners with the initial value p = σ∙h = 1000 kN/m is specified on one of the two opposite transverse sides of the plate subjected to the compressive forces, and constraints in the respective direction on the plate (along the X axis of the global coordinate system) are imposed on the nodes of the other one, and the load uniformly distributed along the line on the stiffeners with the initial value p = σ∙h = 1000 kN/m is specified on it. The dimensional stability of the design model is provided by imposing constraints in the normal direction (along the Y axis of the global coordinate system) on the nodes of one of the two opposite longitudinal sides of the plate free from forces, and by imposing a constraint in the UZ direction of the global coordinate system on the node of one of the corners of the plate. Number of nodes in the models – 381; 1437.
Results in SCAD
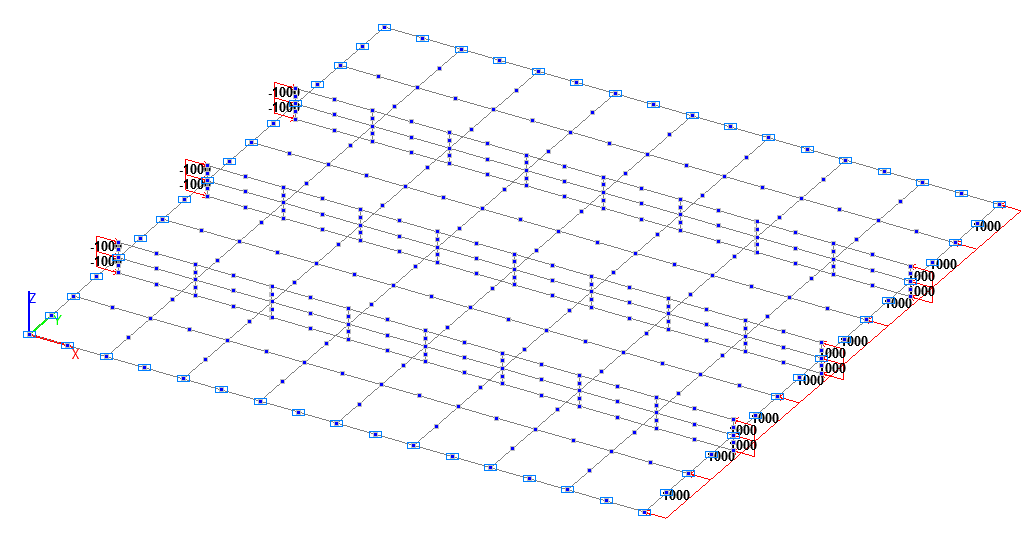
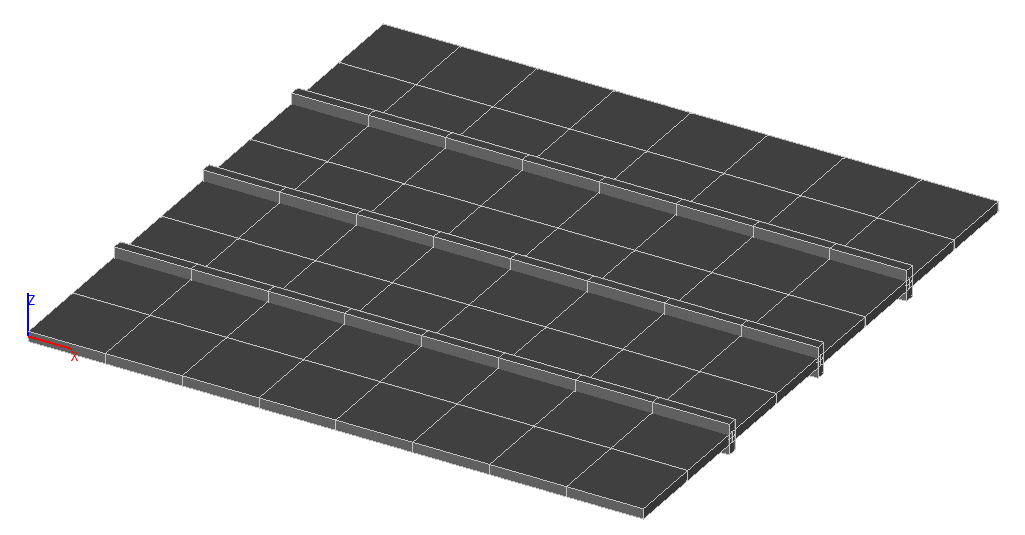
Design model with the ratio of the sides of the plate a/b = 1.0


Design model with the ratio of the sides of the plate a/b = 4.0
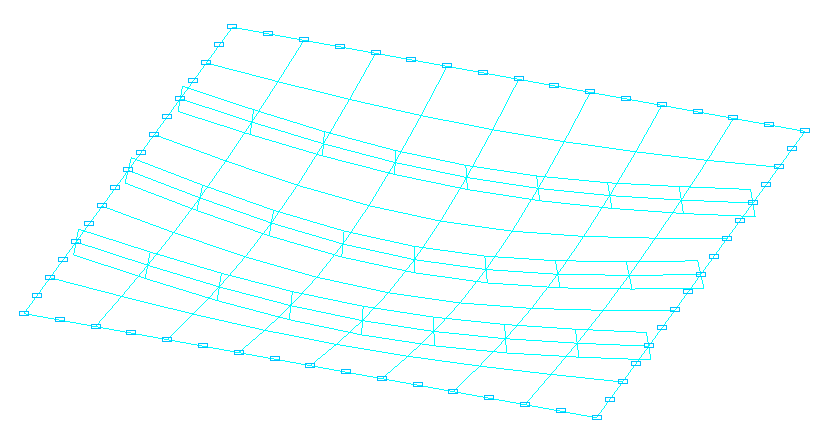
1-st buckling mode for the design model with the ratio of the sides of the plate a/b = 1.0
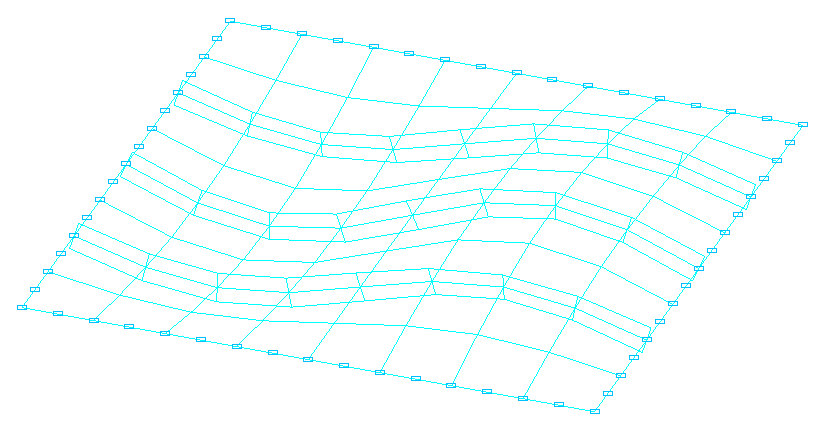
2-nd buckling mode for the design model with the ratio of the sides of the plate a/b = 1.0
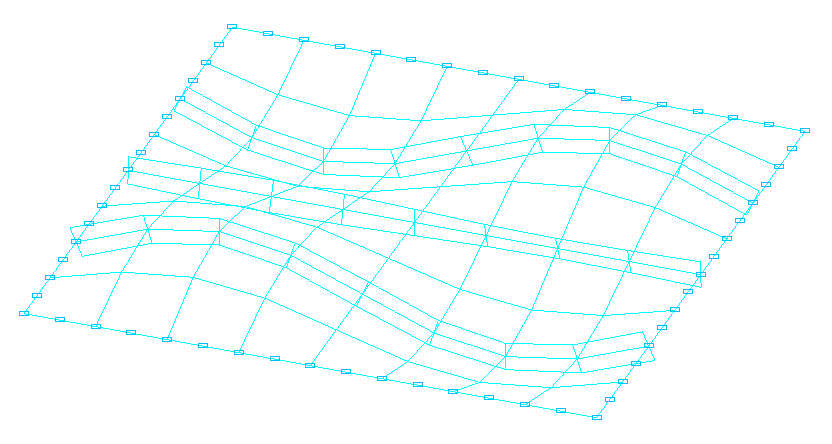
3-rd buckling mode for the design model with the ratio of the sides of the plate a/b = 1.0
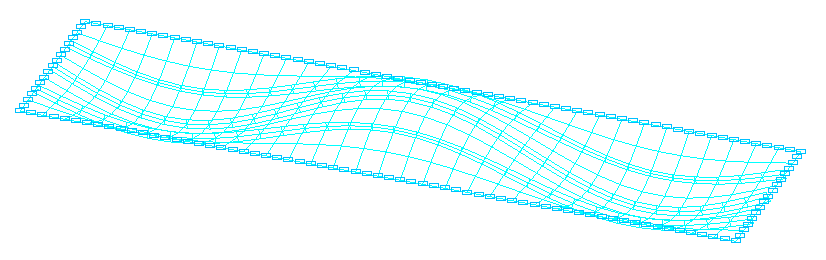
1-st buckling mode for the design model with the ratio of the sides of the plate a/b = 4.0
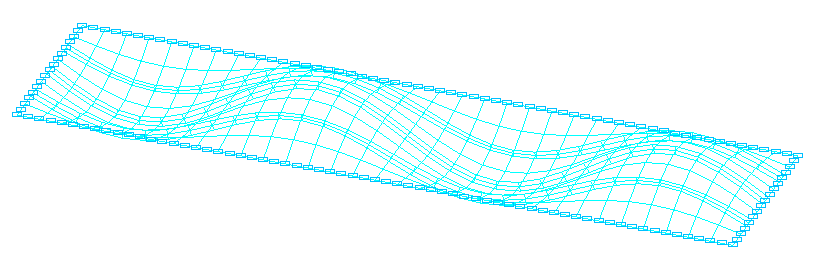
2-nd buckling mode for the design model with the ratio of the sides of the plate a/b = 4.0
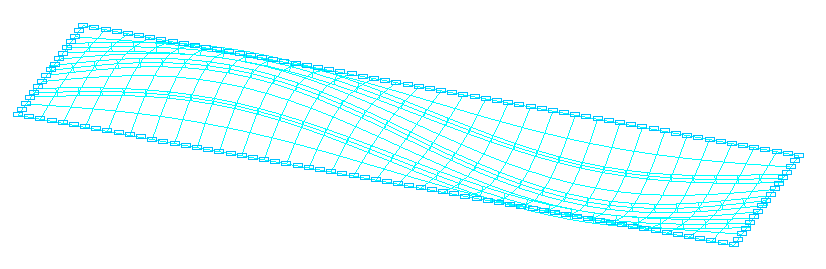
3-rd buckling mode for the design model with the ratio of the sides of the plate a/b = 4.0
Comparison of solutions:
Critical value of the compressive forces σcr, kN/m2
|
Plate sides ratio |
Buckling mode |
Number of half waves in the transverse n and in the longitudinal m directions |
Theory |
SCAD |
Deviation, % |
|---|---|---|---|---|---|
|
a/b = 1.0 |
1 |
1; 1 |
235900 (235911) |
2.410318∙1000/0.01 = = 241032 |
2.18 |
|
2 |
1; 2 |
533934 (535675) |
5.369516∙1000/0.01 = = 536952 |
0.57 |
|
|
3 |
2; 2 |
942681 (943645) |
9.604025∙1000/0.01 = = 960403 |
1.88 |
|
|
a/b = 4.0 |
1 |
1; 3 |
220165 (220164) |
2.257856∙1000/0.01 = = 225786 |
2.55 |
|
2 |
1; 4 |
235900 (235911) |
2.414278∙1000/0.01 = = 241428 |
2.34 |
|
|
3 |
1; 2 |
278652 (278654) |
2.842984∙1000/0.01 = = 284298 |
2.03 |
Theoretical values calculated in the fourth approximation are given without brackets;
Theoretical values calculated in the first approximation are given in brackets
Notes: In the analytical solution the critical value of the compressive forces σcr1 in the first approximation corresponding to the moment of buckling of the rectangular reinforced plate can be determined according to the following formula:
\[ \sigma_{cr1} =\frac{\pi^{2}\cdot D\cdot m^{2}}{b^{2}\cdot h\cdot \lambda ^{2}}\cdot \frac{\left[ {1+\left( {\frac{n\cdot \lambda }{m}} \right)^{2}} \right]^{2}+2\cdot \gamma \cdot \sum\limits_{i=1}^s {\sin^{2}\left( {\frac{\pi \cdot i}{s+1}} \right)} }{1+2\cdot \delta \cdot \sum\limits_{i=1}^s {\sin^{2}\left( {\frac{\pi \cdot i}{s+1}} \right)} }, \] \[ at \quad s = \quad 3 \sigma_{cr1} =\frac{\pi^{2}\cdot D\cdot m^{2}}{b^{2}\cdot h\cdot \lambda^{2}}\cdot \frac{\left[ {1+\left( {\frac{n\cdot \lambda }{m}} \right)^{2}} \right]^{2}+4\cdot \gamma }{1+4\cdot \delta }, \quad where: \] \[ D=\frac{E\cdot h^{3}}{12\cdot \left( {1-\nu^{2}} \right)}, \quad \lambda =\frac{a}{b}, \quad \gamma =\frac{E\cdot I}{b\cdot D}, \quad \delta =\frac{F}{b\cdot h}, \]
n, m = 1, 2, 3 … – number of half waves of the buckling mode in the transverse and longitudinal directions with respect to the compression of the plate.
In the analytical solution the critical value of the compressive forces σcr1 in the fourth approximation corresponding to the moment of buckling of the rectangular reinforced plate is determined on the basis of the condition of equality to zero of the determinant of the system of governing equations:
\[ \left| {{\begin{array}{*{20}c} {\begin{array}{l} \frac{\pi^{2}\cdot D\cdot m^{2}}{b^{2}\cdot h\cdot \lambda^{2}}\cdot \left[ {\left[ {1+\left( {\frac{n\cdot \lambda }{m}} \right)^{2}} \right]^{2}+4\cdot \gamma } \right] \\ -\sigma_{cr4} \cdot \left( {1+4\cdot \delta } \right) \\ \end{array}} & {-\frac{\pi^{2}\cdot D\cdot m^{2}}{b^{2}\cdot h\cdot \lambda^{2}}\cdot 4\cdot \gamma +\sigma_{cr4} \cdot 4\cdot \delta } & {\frac{\pi^{2}\cdot D\cdot m^{2}}{b^{2}\cdot h\cdot \lambda^{2}}\cdot 4\cdot \gamma -\sigma_{cr4} \cdot 4\cdot \delta } & {-\frac{\pi ^{2}\cdot D\cdot m^{2}}{b^{2}\cdot h\cdot \lambda^{2}}\cdot 4\cdot \gamma +\sigma_{cr4} \cdot 4\cdot \delta } \\ {-\frac{\pi^{2}\cdot D\cdot m^{2}}{b^{2}\cdot h\cdot \lambda^{2}}\cdot 4\cdot \gamma +\sigma_{cr4} \cdot 4\cdot \delta } & {\begin{array}{l} \frac{\pi^{2}\cdot D\cdot m^{2}}{b^{2}\cdot h\cdot \lambda^{2}}\cdot \left[ {\left[ {1+49\cdot \left( {\frac{n\cdot \lambda }{m}} \right)^{2}} \right]^{2}+4\cdot \gamma } \right] \\ -\sigma_{cr4} \cdot \left( {1+4\cdot \delta } \right) \\ \end{array}} & {-\frac{\pi^{2}\cdot D\cdot m^{2}}{b^{2}\cdot h\cdot \lambda^{2}}\cdot 4\cdot \gamma +\sigma_{cr4} \cdot 4\cdot \delta } & {\frac{\pi^{2}\cdot D\cdot m^{2}}{b^{2}\cdot h\cdot \lambda^{2}}\cdot 4\cdot \gamma -\sigma_{cr4} \cdot 4\cdot \delta } \\ {\frac{\pi^{2}\cdot D\cdot m^{2}}{b^{2}\cdot h\cdot \lambda^{2}}\cdot 4\cdot \gamma -\sigma_{cr4} \cdot 4\cdot \delta } & {-\frac{\pi ^{2}\cdot D\cdot m^{2}}{b^{2}\cdot h\cdot \lambda^{2}}\cdot 4\cdot \gamma +\sigma_{cr4} \cdot 4\cdot \delta } & {\begin{array}{l} \frac{\pi^{2}\cdot D\cdot m^{2}}{b^{2}\cdot h\cdot \lambda^{2}}\cdot \left[ {\left[ {1+81\cdot \left( {\frac{n\cdot \lambda }{m}} \right)^{2}} \right]^{2}+4\cdot \gamma } \right] \\ -\sigma_{cr4} \cdot \left( {1+4\cdot \delta } \right) \\ \end{array}} & {-\frac{\pi^{2}\cdot D\cdot m^{2}}{b^{2}\cdot h\cdot \lambda^{2}}\cdot 4\cdot \gamma +\sigma_{cr4} \cdot 4\cdot \delta } \\ {-\frac{\pi^{2}\cdot D\cdot m^{2}}{b^{2}\cdot h\cdot \lambda^{2}}\cdot 4\cdot \gamma +\sigma_{cr4} \cdot 4\cdot \delta } & {\frac{\pi ^{2}\cdot D\cdot m^{2}}{b^{2}\cdot h\cdot \lambda^{2}}\cdot 4\cdot \gamma -\sigma_{cr4} \cdot 4\cdot \delta } & {-\frac{\pi^{2}\cdot D\cdot m^{2}}{b^{2}\cdot h\cdot \lambda^{2}}\cdot 4\cdot \gamma +\sigma_{cr4} \cdot 4\cdot \delta } & {\begin{array}{l} \frac{\pi^{2}\cdot D\cdot m^{2}}{b^{2}\cdot h\cdot \lambda^{2}}\cdot \left[ {\left[ {1+225\cdot \left( {\frac{n\cdot \lambda }{m}} \right)^{2}} \right]^{2}+4\cdot \gamma } \right] \\ -\sigma_{cr4} \cdot \left( {1+4\cdot \delta } \right) \\ \end{array}} \\ \end{array} }} \right|=0 \]
