Stability of a Beam of a Square Cross-Section Simply Supported in and out of the Bending Plane Subjected to a Concentrated Transverse Bending Force Applied in the Middle of the Span at the Level of the Longitudinal Axis (Transverse Bending)
Objective: Determination of the critical value of the concentrated transverse bending force applied in the middle of the span at the level of the longitudinal axis of a beam of a square cross-section simply supported in and out of the bending plane corresponding to the moment of its buckling.
Initial data files:
|
File name |
Description |
|---|---|
|
Bar model |
|
|
Shell element model |
Problem formulation: The beam of a square cross-section simply supported in and out of the bending plane is subjected to the action of the concentrated transverse bending force P, applied in the middle of its span at the level of the longitudinal axis. Determine the critical value of the concentrated transverse bending force P, corresponding to the moment of buckling of the simply supported beam.
References: A.S. Volmir. Stability of Deformable Systems, Moscow, Nauka, 1967, p.218
Initial data:
| L = 10.0 m | - length of the simply supported beam; |
| h = b = 1.0 m | - side of the square cross-section of the simply supported beam; |
| E = 3.0·107 kN/m2 | - elastic modulus of the simply supported beam material; |
| ν = 0.2 | - Poisson’s ratio; |
| P = 106 кН | - initial value of the concentrated transverse bending force applied in the middle of the span at the level of the longitudinal axis of the beam. |
Finite element model: Design model – general type system. Two design models are considered:
Bar model (B), 10 elements of type 5, the spacing of the finite element mesh along the longitudinal axis is 1.0 m. Boundary conditions are provided by imposing constraints on the nodes of the simply supported ends of the beam in the directions of the degrees of freedom X, Y, Z, UX. The action with the initial value of the concentrated transverse bending force P is specified in the node in the middle of the beam span. Number of nodes in the design model – 11;
Reissner-Mindlin shell element model (P), 2560 eight-node elements of type 150, the spacing of the finite element mesh along the longitudinal axis and along the height of the beam is 0.0625 m. The shell is supported by vertical high-rigidity bars (h = b = 1.0 m; E = 3.0·109 kN/m2; ν = 0.2), 64 elements of type 5. Boundary conditions are provided by imposing constraints on the nodes of the ends of the beam lying on its longitudinal axis in the directions of the degrees of freedom X, Y, Z and on all other nodes of the ends of the beam in the direction of the degree of freedom Y. The action with the initial value of the concentrated transverse bending force P is specified in the node in the middle of the beam span at the level of the longitudinal axis of the beam. Number of nodes in the design model – 8033.
Results in SCAD

Design model. Bar model
Design model. Reissner-Mindlin shell element model
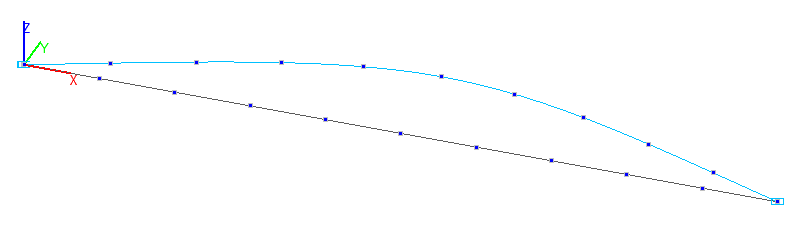
1-st buckling mode. Bar model
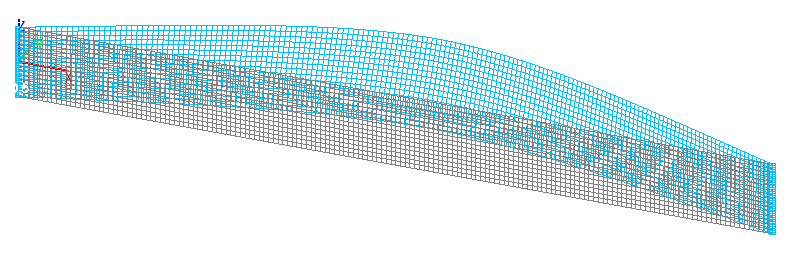
1- st Buckling mode. Reissner-Mindlin shell element model
Comparison of solutions:
Critical value of the concentrated transverse bending force Pcr (kN), applied in the middle of the span at the level of the longitudinal axis of the beam simply supported in and out of the bending plane
|
Design model |
Theory |
SCAD |
Deviation, % |
|---|---|---|---|
|
Bar |
355055 |
0,353193∙1000000=353193 |
0,52 |
|
Reissner-Mindlin shell element |
355055 |
0,344706∙1000000=344706 |
2,91 |
Notes: In the analytical solution the critical value of the concentrated transverse bending force Pcr, corresponding to the moment of buckling of the simply supported beam can be determined according to the following formula:
\[ P=\frac{16,94\cdot \sqrt {E\cdot I_{z} \cdot G\cdot I_{x} } }{L^{2}} \quad G=\frac{E}{2\cdot \left( {1+\nu } \right)} \]
\( I_{z} =\frac{h\cdot b^{3}}{12} \) – minimum bending inertia moment (out of the moment plane);
\( I_{y} =\frac{b\cdot h^{3}}{12} \) – maximum bending inertia moment (in the moment plane);
\( I_{x} =k_{f} \cdot h\cdot b^{3} \) – free torsional inertia moment, where:
\[ k_{f} =\frac{1}{3}\cdot \left\{ {1-\frac{192}{\pi^{5}}\cdot \frac{b}{h}\cdot \sum\limits_{n=1}^\infty {\left[ {\sin^{2}\left( {\frac{n\cdot \pi }{2}} \right)\cdot \frac{1}{n^{5}}\cdot th\left( {\frac{n\cdot \pi \cdot h}{2\cdot b}} \right)} \right]} } \right\} \]
