Beam on the Elastic Horizontal Subgrade Subjected to Concentrated Vertical Forces
Objective: Determination of the stress-strain state of a beam on the elastic horizontal subgrade subjected to concentrated vertical forces.
Initial data files:
|
File name |
Description |
|---|---|
|
Design model – bar elements on the elastic subgrade |
|
|
Design model – bar elements on elastic supports in the form of elements of constraints of finite rigidity of type 51 |
Problem formulation: The beam on the elastic horizontal subgrade with the stiffness k constant along the length is subjected to three concentrated vertical forces of the same value F, applied at the edges (points A and B) and in the middle of the span (point C). Determine the vertical displacements Z in the middle of the beam span (point C) and at its edges (points A and B), rotation angles UY of the beam edges, as well as the bending moment M in the middle of the beam span.
References: M. Courtand et P. Lebelle, Formulaire du beton arme, t.2, Paris, Eyrolles,1976, p. 382.
Initial data:
| E = 2.1•1011 Pa | - elastic modulus; |
| l = 0.5•π•(10.0)0.5 = 4.967294133 m | - beam length; |
| b = 1.0 m | - beam width; |
| Iy = 1.0•10-4 m4 | - cross-sectional moment of inertia of the beam; |
| kz = 8.4•105 N/m3 | - subsoil parameter; |
| F = 1.0•104 N | - value of the concentrated vertical force. |
Finite element model: Two variants of the design model are considered.
Variant 1:
Design model – grade beam / plate, 12 bar elements of type 3 on the elastic subgrade directed along the Z1 axis of the local coordinate system. Number of nodes in the design model – 13.
Variant 2:
Design model – grade beam / plate, 12 bar elements of type 3 on the elastic supports in the form of 13 elements of constraints of finite rigidity of type 51 directed along the Z axis of the global coordinate system. Stiffness of intermediate elastic supports: kz∙b∙l/12 = 347711 N/m, stiffness of end elastic supports: 0.5∙kz∙b∙l/12 = 173855 N/m. In order to prevent the dimensional instability of the system, a constraint in the direction of the degree of freedom UX is imposed along the beam symmetry axis and the minimum torsional stiffness of the beam is introduced GIx = 1.0 N∙m2. Number of nodes in the design model – 13.
Results in SCAD

Design and deformed models. Variant 1

Design and deformed models. Variant 2

Values of vertical displacements Z (m) for the design model according to variant 1
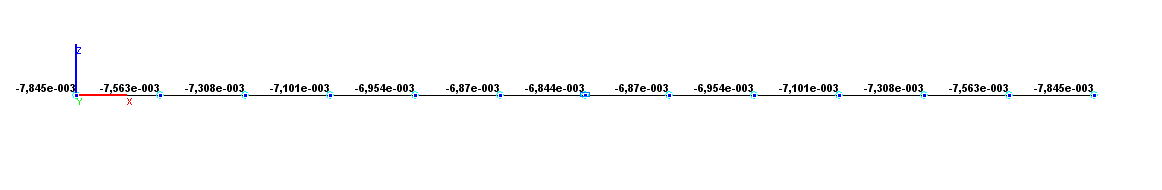
Values of vertical displacements Z (m) for the design model according to variant 2
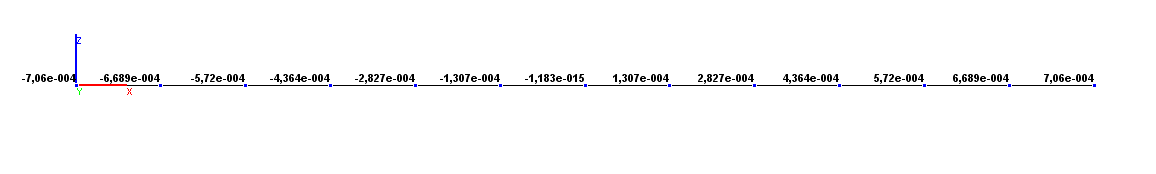
Values of rotation angles UY (rad) for the design model according to variant 1

Values of rotation angles UY (rad) for the design model according to variant 2

Values of bending moments M (N∙m) for the design model according to variant 1
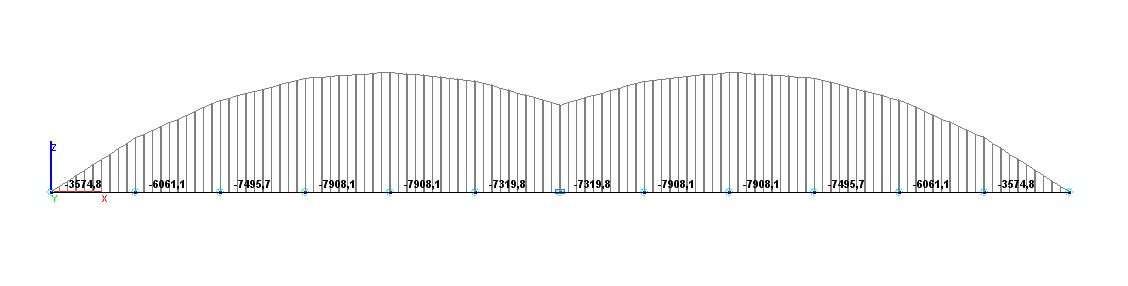
Values of bending moments M (N∙m) for the design model according to variant 2
Comparison of solutions:
|
Parameter |
Theory |
SCAD DM according to variant 1 |
Deviations, % |
SCAD DM according to variant 2 |
Deviations, % |
|---|---|---|---|---|---|
|
Vertical displacement ZC, m |
-6.844∙10-3 |
-6.843∙10-3 |
0.01 |
-6.844∙10-3 |
0.00 |
|
Vertical displacement ZA, m |
-7.854∙10-3 |
-7.859∙10-3 |
0.06 |
-7.845∙10-3 |
0.11 |
|
Rotation angle UYA, rad |
-7.060∙10-4 |
-7.060∙10-4 |
0.00 |
-6.945∙10-4 |
1.63 |
|
Bending moment MC, N∙m |
-5759.0 |
-5758.8 |
0.00 |
-5742.6 |
0.28 |