Amplitude-Frequency Characteristic of a System with One Degree of Freedom

Objective: Plotting the amplitude-frequency characteristic of a single-mass elastic system under harmonic excitation.

Initial data files: TestАЧХ.SPR

Problem formulation: The behavior of a single-mass elastic system subjected to an excitation by a harmonic time-varying force with different excitation frequencies.

References: Panovko Ya.G. Introduction to the Theory of Mechanical Oscillations — M.: Nauka, 1991, § II.6.

Initial data:

М = 10 kN	- weight of the mass;
С = 100 kN	- stiffness;
Р = 10 kN	- amplitude value of the force;
ξ = 0.025	- damping parameter (in fractions of the critical value).

Finite element model: One node where a point mass is specified is supported by a single-node elastic constraint (finite element of type 51).

Results in SCAD

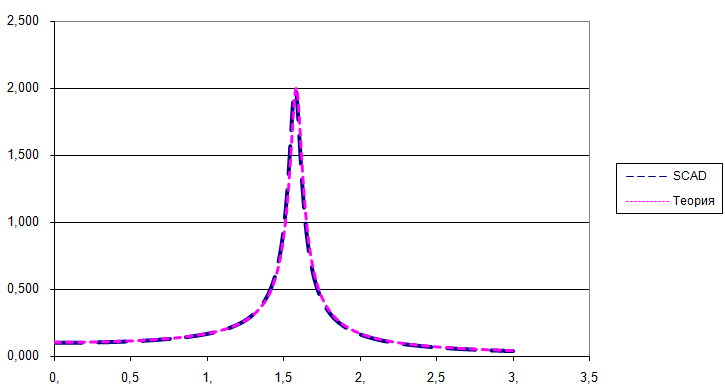
Amplitude-frequency characteristics

Comparison of solutions:

Frequency	Displacement of the node
	SCAD	Theory
Hz	m
0,	0,1000	0,1000
0,01	0,1000	0,1000
0,02	0,1000	0,1000
0,03	0,1000	0,1000
0,04	0,1001	0,1001
0,05	0,1001	0,1001
0,06	0,1001	0,1001
0,07	0,1002	0,1002
0,08	0,1003	0,1003
0,09	0,1003	0,1003
0,1	0,1004	0,1004
0,11	0,1005	0,1005
0,12	0,1006	0,1006
0,13	0,1007	0,1007
0,14	0,1008	0,1008
0,15	0,1009	0,1009
0,16	0,1010	0,1010
0,17	0,1012	0,1012
0,18	0,1013	0,1013
0,19	0,1015	0,1015
0,2	0,1016	0,1016
0,21	0,1018	0,1018
0,22	0,1020	0,1020
0,23	0,1022	0,1022
0,24	0,1024	0,1024
0,25	0,1026	0,1026
0,26	0,1028	0,1028
0,27	0,1030	0,1030
0,28	0,1033	0,1032
0,29	0,1035	0,1035
0,3	0,1038	0,1037
0,31	0,1040	0,1040
0,32	0,1043	0,1043
0,33	0,1046	0,1046
0,34	0,1049	0,1048
0,35	0,1052	0,1052
0,36	0,1055	0,1055
0,37	0,1058	0,1058
0,38	0,1062	0,1061
0,39	0,1065	0,1065
0,4	0,1069	0,1068
0,41	0,1072	0,1072
0,42	0,1076	0,1076
0,43	0,1080	0,1080
0,44	0,1084	0,1084
0,45	0,1089	0,1088
0,46	0,1093	0,1092
0,47	0,1097	0,1097
0,48	0,1102	0,1102
0,49	0,1107	0,1106
0,5	0,1112	0,1111
0,51	0,1117	0,1116
0,52	0,1122	0,1121
0,53	0,1127	0,1127
0,54	0,1133	0,1132
0,55	0,1138	0,1138
0,56	0,1144	0,1143
0,57	0,1150	0,1149
0,58	0,1156	0,1155
0,59	0,1163	0,1162
0,6	0,1169	0,1168
0,61	0,1176	0,1175
0,62	0,1183	0,1182
0,63	0,1190	0,1189
0,64	0,1197	0,1196
0,65	0,1204	0,1203
0,66	0,1212	0,1211
0,67	0,1220	0,1219
0,68	0,1228	0,1227
0,69	0,1237	0,1235
0,7	0,1245	0,1244
0,71	0,1254	0,1253
0,72	0,1263	0,1262
0,73	0,1272	0,1271
0,74	0,1282	0,1280
0,75	0,1292	0,1290
0,76	0,1302	0,1300
0,77	0,1313	0,1311
0,78	0,1324	0,1322
0,79	0,1335	0,1333
0,8	0,1346	0,1344
0,81	0,1358	0,1356
0,82	0,1370	0,1368
0,83	0,1383	0,1380
0,84	0,1396	0,1393
0,85	0,1409	0,1406
0,86	0,1423	0,1420
0,87	0,1437	0,1434
0,88	0,1452	0,1449
0,89	0,1467	0,1464
0,9	0,1482	0,1479
0,91	0,1498	0,1495
0,92	0,1515	0,1512
0,93	0,1532	0,1529
0,94	0,1550	0,1546
0,95	0,1569	0,1564
0,96	0,1588	0,1583
0,97	0,1607	0,1603
0,98	0,1628	0,1623
0,99	0,1649	0,1644
1,	0,1671	0,1666
1,01	0,1694	0,1689
1,02	0,1718	0,1712
1,03	0,1742	0,1736
1,04	0,1768	0,1762
1,05	0,1794	0,1788
1,06	0,1822	0,1815
1,07	0,1851	0,1844
1,08	0,1881	0,1873
1,09	0,1912	0,1904
1,1	0,1945	0,1936
1,11	0,1979	0,1970
1,12	0,2014	0,2005
1,13	0,2051	0,2042
1,14	0,2090	0,2080
1,15	0,2131	0,2120
1,16	0,2174	0,2162
1,17	0,2219	0,2207
1,18	0,2266	0,2253
1,19	0,2316	0,2302
1,2	0,2368	0,2354
1,21	0,2424	0,2408
1,22	0,2482	0,2465
1,23	0,2544	0,2526
1,24	0,2609	0,2590
1,25	0,2679	0,2658
1,26	0,2752	0,2731
1,27	0,2831	0,2808
1,28	0,2915	0,2890
1,29	0,3004	0,2977
1,3	0,3100	0,3071
1,31	0,3203	0,3172
1,32	0,3314	0,3280
1,33	0,3434	0,3396
1,34	0,3563	0,3522
1,35	0,3704	0,3659
1,36	0,3857	0,3807
1,37	0,4024	0,3970
1,38	0,4207	0,4147
1,39	0,4409	0,4342
1,4	0,4633	0,4558
1,41	0,4881	0,4797
1,42	0,5159	0,5064
1,43	0,5471	0,5364
1,44	0,5824	0,5702
1,45	0,6226	0,6086
1,46	0,6688	0,6525
1,47	0,7222	0,7032
1,48	0,7845	0,7621
1,49	0,8578	0,8312
1,5	0,9449	0,9130
1,51	1,0490	1,0106
1,52	1,1740	1,1276
1,53	1,3230	1,2675
1,54	1,4965	1,4323
1,55	1,6863	1,6178
1,56	1,8651	1,8048
1,57	1,9824	1,9508
1,58	1,9869	2,0000
1,59	1,8746	1,9270
1,6	1,6930	1,7643
1,61	1,4959	1,5684
1,62	1,3140	1,3792
1,63	1,1575	1,2132
1,64	1,0264	1,0732
1,65	0,9174	0,9565
1,66	0,8265	0,8594
1,67	0,7501	0,7780
1,68	0,6854	0,7092
1,69	0,6301	0,6506
1,7	0,5824	0,6003
1,71	0,5409	0,5566
1,72	0,5045	0,5184
1,73	0,4724	0,4848
1,74	0,4439	0,4550
1,75	0,4184	0,4284
1,76	0,3956	0,4046
1,77	0,3749	0,3831
1,78	0,3561	0,3636
1,79	0,3390	0,3459
1,8	0,3234	0,3297
1,81	0,3091	0,3149
1,82	0,2959	0,3013
1,83	0,2837	0,2887
1,84	0,2724	0,2771
1,85	0,2619	0,2663
1,86	0,2521	0,2562
1,87	0,2430	0,2468
1,88	0,2344	0,2381
1,89	0,2264	0,2299
1,9	0,2189	0,2222
1,91	0,2119	0,2149
1,92	0,2052	0,2081
1,93	0,1989	0,2017
1,94	0,1930	0,1956
1,95	0,1873	0,1898
1,96	0,1820	0,1844
1,97	0,1769	0,1792
1,98	0,1721	0,1743
1,99	0,1675	0,1696
2,	0,1631	0,1651
2,01	0,1590	0,1609
2,02	0,1550	0,1568
2,03	0,1512	0,1529
2,04	0,1475	0,1492
2,05	0,1440	0,1457
2,06	0,1407	0,1423
2,07	0,1375	0,1390
2,08	0,1344	0,1359
2,09	0,1314	0,1329
2,1	0,1286	0,1300
2,11	0,1259	0,1272
2,12	0,1232	0,1245
2,13	0,1207	0,1219
2,14	0,1182	0,1194
2,15	0,1159	0,1170
2,16	0,1136	0,1147
2,17	0,1114	0,1125
2,18	0,1093	0,1103
2,19	0,1072	0,1082
2,2	0,1052	0,1062
2,21	0,1033	0,1043
2,22	0,1014	0,1024
2,23	0,0996	0,1005
2,24	0,0979	0,0988
2,25	0,0962	0,0971
2,26	0,0945	0,0954
2,27	0,0929	0,0938
2,28	0,0914	0,0922
2,29	0,0899	0,0907
2,3	0,0884	0,0892
2,31	0,0870	0,0877
2,32	0,0856	0,0863
2,33	0,0842	0,0850
2,34	0,0829	0,0836
2,35	0,0817	0,0823
2,36	0,0804	0,0811
2,37	0,0792	0,0799
2,38	0,0780	0,0787
2,39	0,0769	0,0775
2,4	0,0757	0,0764
2,41	0,0747	0,0753
2,42	0,0736	0,0742
2,43	0,0725	0,0731
2,44	0,0715	0,0721
2,45	0,0705	0,0711
2,46	0,0696	0,0701
2,47	0,0686	0,0692
2,48	0,0677	0,0682
2,49	0,0668	0,0673
2,5	0,0659	0,0664
2,51	0,0650	0,0655
2,52	0,0642	0,0647
2,53	0,0634	0,0639
2,54	0,0626	0,0630
2,55	0,0618	0,0622
2,56	0,0610	0,0615
2,57	0,0602	0,0607
2,58	0,0595	0,0599
2,59	0,0588	0,0592
2,6	0,0581	0,0585
2,61	0,0574	0,0578
2,62	0,0567	0,0571
2,63	0,0560	0,0564
2,64	0,0553	0,0557
2,65	0,0547	0,0551
2,66	0,0541	0,0545
2,67	0,0535	0,0538
2,68	0,0528	0,0532
2,69	0,0522	0,0526
2,7	0,0517	0,0520
2,71	0,0511	0,0514
2,72	0,0505	0,0509
2,73	0,0500	0,0503
2,74	0,0494	0,0498
2,75	0,0489	0,0492
2,76	0,0484	0,0487
2,77	0,0479	0,0482
2,78	0,0473	0,0477
2,79	0,0469	0,0472
2,8	0,0464	0,0467
2,81	0,0459	0,0462
2,82	0,0454	0,0457
2,83	0,0449	0,0452
2,84	0,0445	0,0448
2,85	0,0440	0,0443
2,86	0,0436	0,0439
2,87	0,0432	0,0435
2,88	0,0427	0,0430
2,89	0,0423	0,0426
2,9	0,0419	0,0422
2,91	0,0415	0,0418
2,92	0,0411	0,0414
2,93	0,0407	0,0410
2,94	0,0403	0,0406
2,95	0,0399	0,0402
2,96	0,0396	0,0398
2,97	0,0392	0,0394
2,98	0,0388	0,0391
2,99	0,0385	0,0387
3,	0,0381	0,0384

	Theory	SCAD	Deviation
Frequency at which the maximum displacement occurs (Hz)	1.58	1.58	0 %
Maximum displacement (m)	2,0000	1,9869	0.65 %

Notes: In the analytical solution the vertical displacement is described by the following transfer function:

\[ \frac{1}{\sqrt {\left( {1-\frac{\theta^{2}}{\omega^{2}}} \right)^{2}+\left( {2\xi \theta /\omega } \right)^{2}} ,} \]

where w — natural frequency of the undamped system.