Plane System of Two Coaxial Bars Subjected to Temperature Variation
Objective: Determination of the stress state of a plane system of two coaxial bars subjected to temperature variation.
Initial data file: B1_v11.3.spr
Problem formulation: The system consists of two coaxial horizontal bars of square cross-section, rigidly connected in the common node and clamped at the opposite nodes. The system is subjected to the temperature variation ∆t relative to the assembly temperature. Determine normal stresses σ in the cross-sections of the bars of the system.
References: S.P. Timoshenko, Strength of Materials, Volume 1: Elementary Theory and Problems, Moscow, Nauka, 1965, p.35.
Initial data:
| Es = 2.0·106 kgf/cm2 | - elastic modulus of steel; |
| αs = 1.25·10-5 1/ ºC | - linear thermal expansion coefficient of steel; |
| L1 = 100.0 cm | - length of the left bar; |
| F1 = 1.0·1.0 cm2 | - cross-sectional area of the left bar; |
| L2 = 100.0 cm | - length of the right bar; |
| F2 = 1.0·2.0 cm2 | - cross-sectional area of the right bar; |
| Δt = 60 ºC | - temperature variation of the system. |
Finite element model: Design model – plane frame, 2 elements of type 2. Boundary conditions are provided by imposing constraints in the end nodes of the system in the directions of the degrees of freedom X, Z, UY. The effect of the temperature variation of the system ∆t relative to the assembly temperature is specified as uniform along the longitudinal axes of all bar elements. Number of nodes in the design model – 3.
Results in SCAD

 Design model
Design model
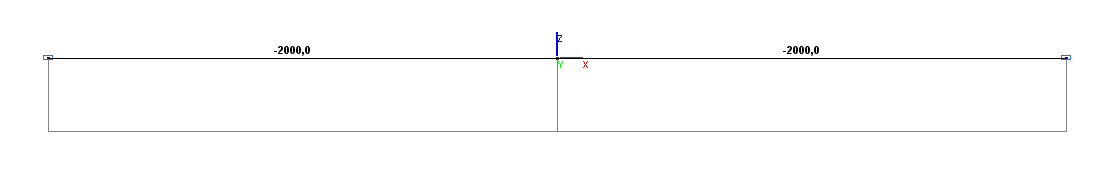
Longitudinal force diagram N (kgf)
Comparison of solutions:
|
Parameter |
Theory |
SCAD |
Deviations, % |
|---|---|---|---|
|
Normal stresses σ (left bar), kgf/cm2 |
-2000.000 |
-2000.0 / (1.0 * 1.0) = = -2000.000 |
0.00 |
|
Normal stresses σ (right bar), kgf/cm2 |
-1000.000 |
-2000.0 / (1.0 * 2.0) = = -1000.000 |
0.00 |
Notes: In the analytical solution, the normal stresses σ in the cross-sections of the bars of the system are determined according to the following formulas:
\[ \sigma_{l} =\frac{\Delta t\cdot \alpha_{s} \cdot E_{s} \cdot \left( {L_{1} +L_{2} } \right)\cdot F_{2} }{L_{1} \cdot F_{2} +L_{2} \cdot F_{1} }; \quad \sigma_{r} =\frac{\Delta t\cdot \alpha_{s} \cdot E_{s} \cdot \left( {L_{1} +L_{2} } \right)\cdot F_{1} }{L_{1} \cdot F_{2} +L_{2} \cdot F_{1} }. \]
