Strain State of a Split Circular Ring Subjected to Two Mutually Perpendicular Forces Px and Py, Acting in the Plane of the Ring
Objective: Strain state of a split circular ring under bending in the plane without taking into account the transverse shear deformations.
Initial data file: 4_6.spr
Problem formulation: The split circular ring is subjected to two mutually perpendicular forces Px and Py, acting in the plane of the ring axes. Determine the strain state of the ring.
References: Strength Analysis in Mechanical Engineering / S. D. Ponomarev, V. L. Biderman, K. K. Likharev, et al., In three volumes. Volume 1. M.: Mashgiz, 1956.
Initial data:
| E = 2.0·1011 Pa | - elastic modulus; |
| R = 1.3 m | - radius of the ring axis; |
| F = 1·10-2 m2 | - cross-sectional area; |
| I = 5·10-6 m4 | - cross-sectional moment of inertia; |
| Px = Py = 1 kN | - value of the concentrated force. |
Finite element model: Design model – plane model, 120 bar elements of type 2, 121 nodes.
Results in SCAD

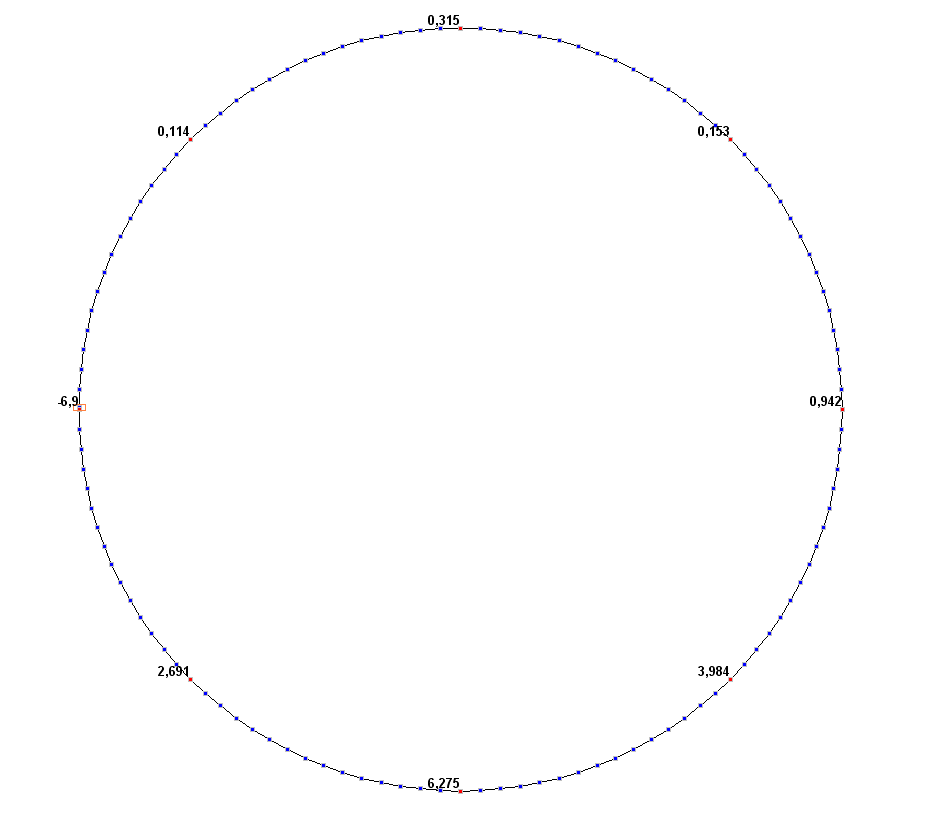
Values of displacements u (mm)
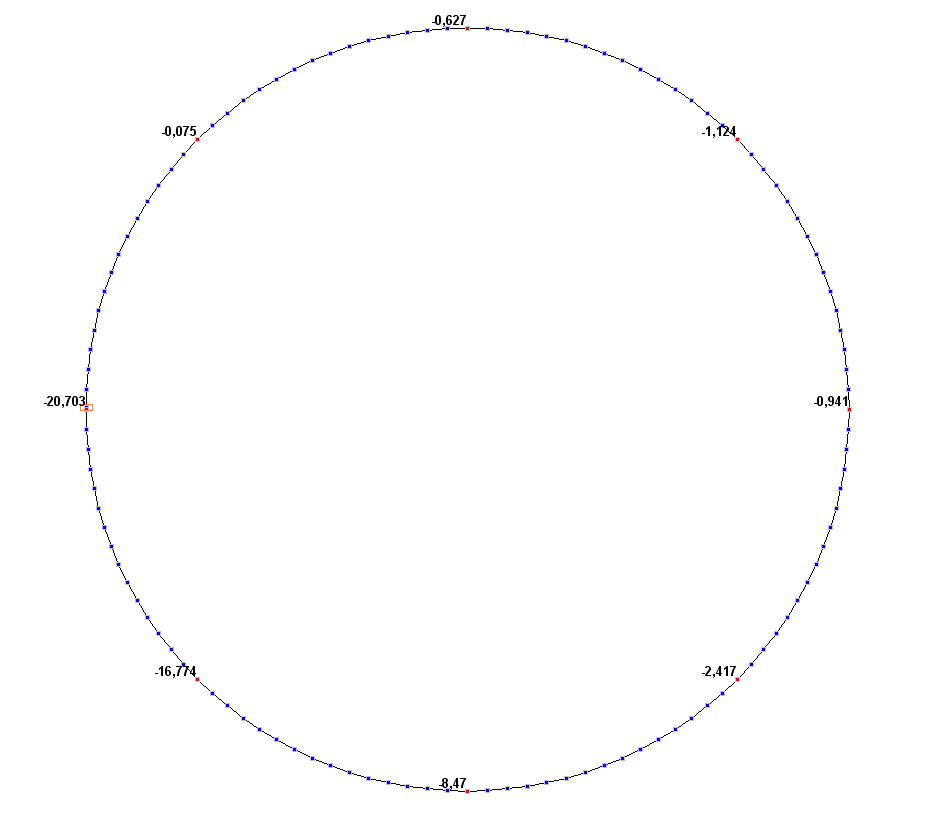
Values of displacements v (mm)
Comparison of solutions:
|
Angle φ, degree |
Displacements along the x axis |
Displacements along the y axis |
||||
|---|---|---|---|---|---|---|
|
Theory |
SCAD |
Deviations, % |
Theory |
SCAD |
Deviations, % |
|
|
0 |
-6.902 |
-6.900 |
0.03 |
-20.706 |
-20.703 |
0.01 |
|
45 |
2.690 |
2.691 |
0.04 |
-16.777 |
-16.774 |
0.02 |
|
90 |
6.275 |
6.275 |
0.00 |
-8.472 |
-8.470 |
0.02 |
|
135 |
3.984 |
3.984 |
0.00 |
-2.419 |
-2.417 |
0.08 |
|
180 |
0.943 |
0.942 |
0.11 |
-0.943 |
-0.941 |
0.21 |
|
225 |
0.154 |
0.153 |
0.65 |
-1.125 |
-1.124 |
0.09 |
|
270 |
0.316 |
0.315 |
0.32 |
-0.627 |
-0.627 |
0.00 |
|
315 |
0.114 |
0.114 |
0.00 |
-0.074 |
-0.075 |
1.35 |
|
360 |
0.000 |
0.000 |
0.00 |
0.000 |
0.000 |
0.00 |
Notes: In the analytical solution the displacements of the points of the ring in the directions x and y are determined according to the following formulas:
\[ u\left( \phi \right)=\frac{P_{x} \cdot R^{3}}{E\cdot I}\cdot \beta_{1} \left( \phi \right)+\frac{P_{y} \cdot R^{3}}{E\cdot I}\cdot \beta_{2} \left( \phi \right), \] where: \[ \beta_{1} \left( \phi \right)=-0.5\cdot \left( {2\cdot \pi -\phi } \right)-\sin \left( \phi \right)+0.5\cdot \sin \left( \phi \right)\cdot \cos \left( \phi \right); \] \[ \beta_{2} \left( \phi \right)=1+\left( {2\cdot \pi -\phi } \right)\cdot \sin \left( \phi \right)-\cos \left( \phi \right)+0.5\cdot \sin^{2}\left( \phi \right); \] \[ v\left( \phi \right)=\frac{P_{x} \cdot R^{3}}{E\cdot I}\cdot \gamma_{1} \left( \phi \right)+\frac{P_{y} \cdot R^{3}}{E\cdot I}\cdot \gamma_{2} \left( \phi \right), \] where: \[ \gamma_{1} \left( \phi \right)=-1+\cos \left( \phi \right)+0.5\cdot \sin ^{2}\left( \phi \right); \] \[ \gamma_{2} \left( \phi \right)=-0.5\cdot \left( {2\cdot \pi -\phi } \right)-\left( {2\cdot \pi -\phi } \right)\cdot \cos \left( \phi \right)-\sin \left( \phi \right)-0.5\cdot \sin \left( \phi \right)\cdot \cos \left( \phi \right). \]
