Triangular Dam Subjected to Its Self-Weight and Hydrostatic Pressure
Objective: Determination of the stress state of a triangular dam of unit thickness in Cartesian coordinates subjected to its self-weight and hydrostatic pressure.
Initial data file: 4_25.spr
Problem formulation: A horizontal load distributed according to the linear law with a unit volume weight γ acting in the plane of the dam is applied to the surface of the vertical face of the triangular dam of unit thickness. The dam is also subjected to the self-weight γ1. Determine the stress tensor components in Cartesian coordinates σx, σy, τxy in the horizontal section of the dam located at the depth of y0 = 5.0 m from the top of the dam.
References: V. I. Samul, Fundamentals of the Elasticity and Plasticity Theory. — Moscow: High school, 1982.
Initial data:
| E = 3.0·107 kPa | - elastic modulus of the dam material; |
| μ = 0.2 | - Poisson’s ratio of the dam material; |
| h = 1.0 m | - thickness of the dam; |
| β = 30º | - apex angle of the dam; |
| H = 15.0 m | - height of the dam; |
| γ = 10.0 kN/m3 | - specific weight of liquid; |
| γ1 = 20.0 кН/м3 | - specific weight of the dam material. |
Finite element model: Design model – plane frame, plate elements – 452 eight-node elements of type 30 and 23 six-node elements of type 25. The spacing of the finite element mesh in the horizontal OX and vertical OY directions is 0.25 m. The direction of the output of internal forces is along the OX and OY axes of the global coordinate system. Since there are no forces distributed according to the law of the analytical solution at the fixed end of the dam, in order to obtain an exact solution at the depth y0 = 5.0 m from the top of the dam under the self-weight and the hydrostatic pressure, the height of the dam to the fixed end is taken as H = 15.0 m. Number of nodes in the design model – 1506.
Results in SCAD
Design model
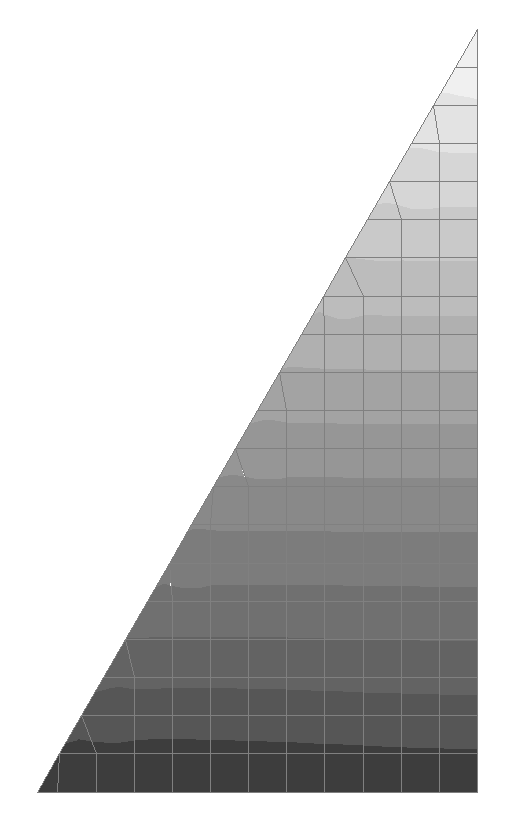
Values of stresses σx (kN/m2)
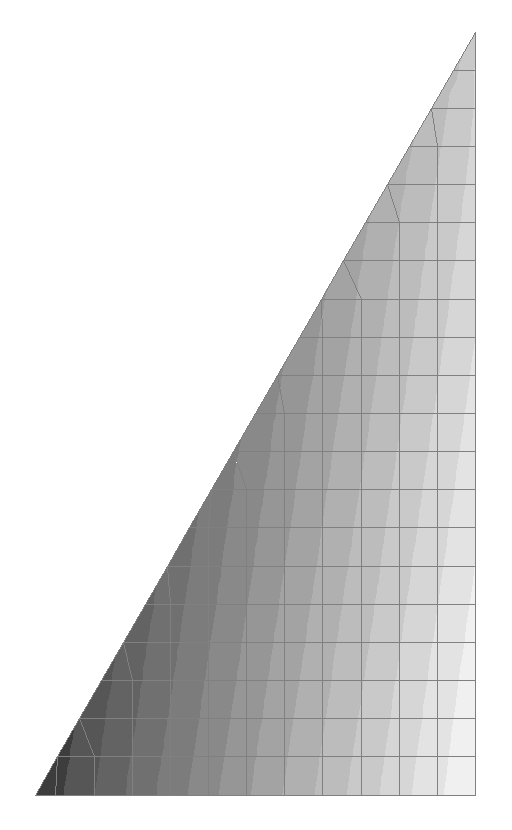

Values of stresses σy (kN/m2)


Values of stresses τxy (kN/m2)
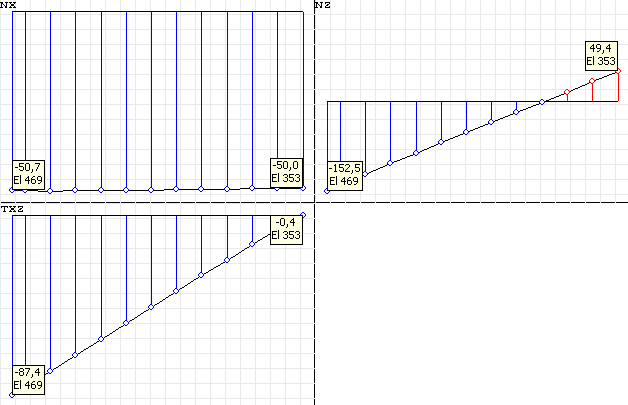
Values of stresses σx, σy, τxy (kN/m2) in the horizontal section of the dam located at the depth of y0 = 5.0 m from the top of the dam
Comparison of solutions:
Stress tensor components in Cartesian coordinates σx, σy, τxy in the horizontal section of the dam located at the depth of y0 = 5.0 m from the top of the dam.
|
Parameter |
On the inclined face of the dam (x = y0·tgβ = 2.8868 m) |
||
|---|---|---|---|
|
Theory |
SCAD |
Deviations, % |
|
|
σx (kN/m2) |
-50.00 |
-50.69 |
1.38 |
|
σy (kN/m2) |
-150.00 |
-152.55 |
1.70 |
|
τxy (kN/m2) |
-86.60 |
-87.42 |
0.95 |
|
Parameter |
On the vertical face of the dam (x = 0.0000 m) |
||
|---|---|---|---|
|
Theory |
SCAD |
Deviations, % |
|
|
σx (kN/m2) |
-50.00 |
-50.00 |
0.00 |
|
σy (kN/m2) |
50.00 |
49.43 |
1.14 |
|
τxy (kN/m2) |
0.00 |
-0.43 |
─ |
Notes: In the analytical solution the stresses σx, σy, τxy in the body of the dam subjected to its self-weight and hydrostatic pressure are determined according to the following formulas (V. I. Samul, Fundamentals of the Elasticity and Plasticity Theory. — Moscow: High school, 1982, p. 77):
\[ \sigma_{x} =-\gamma \cdot y; \quad \sigma_{y} =\left( {\frac{\gamma_{1} }{tg\beta }-\frac{2\cdot \gamma }{tg^{3}\beta }} \right)\cdot x+\left( {\frac{\gamma }{tg^{2}\beta }-\gamma _{1} } \right)\cdot y; \quad \tau_{xy} =-\frac{\gamma \cdot x}{tg^{2}\beta }. \]
