Rectangular Narrow Cantilever Plate Subjected to a Uniformly Distributed Transverse Load
Objective: Determination of the strain state of a rectangular narrow cantilever plate subjected to a uniformly distributed transverse load.
Initial data file: SSLS01_v11.3.spr
Problem formulation: The rectangular narrow cantilever plate is subjected to the transverse load uniformly distributed over its area P. Determine the transverse displacement Z of the free edge of the plate.
References: S. Timoshenko, Resistance des materiaux, t.1, Paris, Librairie Polytechnique Beranger, 1949.
Initial data:
| E = 2.1∙1011 Pa | - elastic modulus; |
| ν = 0.0 | - Poisson’s ratio; |
| l = 1.0 m | - length of the plate; |
| b = 0.1 m | - width of the plate; |
| h = 0.005 m | - thickness of the plate; |
| P = 1.7∙103 N/m2 | - value of the uniformly distributed transverse load. |
Finite element model: Design model – grade beam / plate, 10 plate elements of type 11. Boundary conditions are provided by imposing constraints in the directions of the degrees of freedom Z, UX, UY for the clamped edge. Number of nodes in the design model – 22.
Results in SCAD
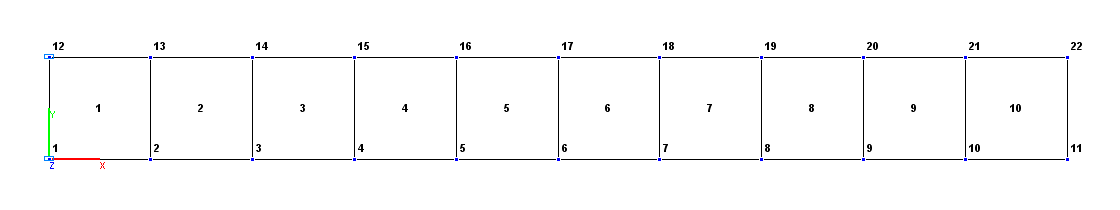
Design model
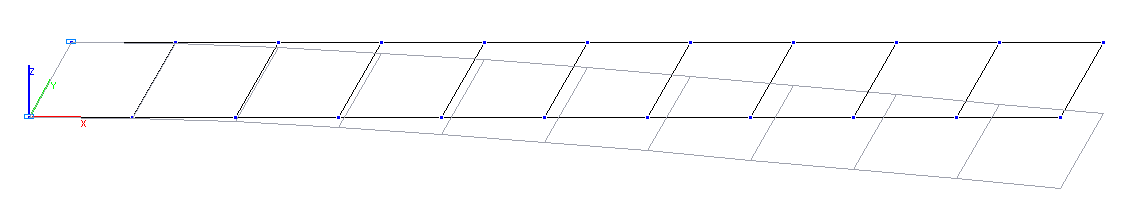

Deformed model


Values of transverse displacements Z (m)
Comparison of solutions:
|
Parameter |
Theory |
SCAD |
Deviations, % |
|---|---|---|---|
|
Transverse displacement of the free edge Z, m |
-9.714∙10-2 |
-9.714∙10-2 |
0.00 |
Notes: In the analytical solution the transverse displacement Z of the free edge of the plate is determined according to the following formula:
\[ Z=\frac{3\cdot P\cdot l^{4}}{2\cdot E\cdot h^{3}}. \]
