Cylindrical Body Free from Restraints Subjected to a Longitudinal Load Uniformly Distributed over the Edges
Objective: Determination of the strain state of a cylindrical body free from restraints subjected to a longitudinal load uniformly distributed over the edges.
Initial data file: SSLV01_v11.5.spr
Problem formulation: The cylindrical body free from restraints is subjected to a longitudinal load uniformly distributed over the edges F/A. Determine the meridional ∆L and radial ∆R displacements of the points E, D, A (C) of the side surface of the cylinder at the distances from its transverse symmetry plane along the generatrix L/3, 2∙L/3, L respectively, as well as the point B of the center of its edge surface.
References: P. Germain, Introduction a la mecanique des milieux continus, Paris, Masson, 1986.
Initial data:
| E = 2.0·105 Pa | - elastic modulus; |
| ν = 0.3 | - Poisson’s ratio; |
| R = 1.0 m | - radius of the cylinder; |
| L = 4.0 m | - length of the cylinder; |
| F/A = 1.0·102 Pa | - load uniformly distributed over the edges. |
Finite element model: Design model – axisymmetric problem, axisymmetric elements – 120 shell elements of type 61. The spacing of the finite element mesh in the meridian direction is 0.25 m and in the radial direction is 0.10 m. The dimensional stability of the design model is provided by imposing constraints according to its symmetry conditions. Number of nodes in the design model – 143.
Results in SCAD
Design and deformed models

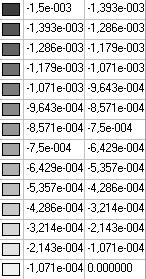
Values of meridional displacements Z (∆L) m
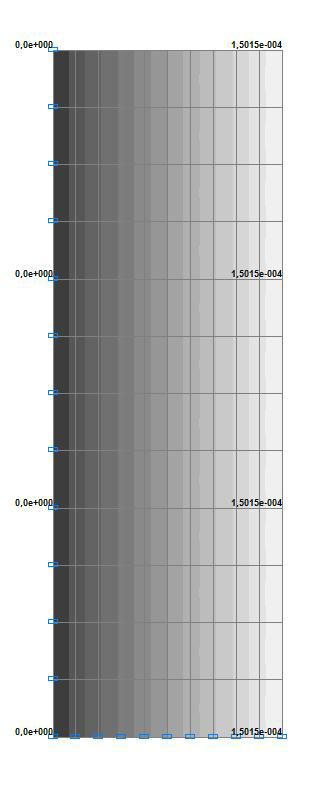
Values of radial displacements X (∆R) m
Comparison of solutions:
|
Parameter |
Theory |
SCAD |
Deviations, % |
|---|---|---|---|
|
Meridional displacement ∆L (point E), m |
-0.500∙10-3 |
-0.500∙10-3 |
0.00 |
|
Radial displacement ∆R (point E), m |
-0.150∙110-3 |
-0.150∙10-3 |
0.00 |
|
Meridional displacement ∆L (point D), m |
-1.000∙10-3 |
-1.000∙10-3 |
0.00 |
|
Radial displacement ∆R (point D), m |
-0.150∙10-3 |
-0.150∙10-3 |
0.00 |
|
Meridional displacement ∆L (points A and C), m |
-1.500∙10-3 |
-1.500∙10-3 |
0.00 |
|
Radial displacement ∆R (points A and C), m |
-0.150∙10-3 |
-0.150∙10-3 |
0.00 |
|
Meridional displacement ∆L (point B), m |
-1.500∙10-3 |
-1.500∙10-3 |
0.00 |
|
Radial displacement ∆R (point B), m |
0.000∙10-3 |
0.000∙10-3 |
0.00 |
Notes: In the analytical solution the meridional ∆L and radial ∆R displacements can be determined according to the following formulas:
\[ \Delta L=\frac{P\cdot X}{E}; \quad \Delta R=\frac{\nu \cdot P\cdot R}{E}. \]
