Simply Supported Weightless Beam with Two Concentrated Masses and Transverse Harmonic Exciting Force Applied to One of Them
Objective: Determination of the strain state of a simply supported weightless beam with two concentrated masses subjected to a transverse harmonic exciting force applied to one of them.
Problem formulation: Two identical loads of mass m are attached to the simply supported beam of constant cross-section at a quarter span distance from each support. The mass of the beam is neglected in comparison with the masses of the loads. The force P0 is applied to one of the masses at the initial time and varies harmonically with the frequency ω. Determine the natural oscillation modes and natural frequencies p of the simply supported beam, as well as the deflections η in the cross-sections of the beam with the attached masses with time.
References: S.D. Ponomarev, V.L. Biederman, K.K. Likharev, V.M. Makushin, N.N. Malinin, V.I. Feodos’yev, Fundamentals of Modern Methods for Strength Analysis in Mechanical Engineering. Dynamic Analysis. Stability. Creep. Moscow, Mashgiz, 1952, p. 153.
Initial data:
| E = 3.0·106 tf/m2 | - elastic modulus; |
| ν = 0.2 | - Poisson’s ratio; |
| b = 0.4 m | - width of the rectangular cross-section of the beam; |
| h = 0.8 m | - height of the rectangular cross-section of the beam; |
| l = 8.0 m | - beam span length; |
| m = 3.0 tf·s2/m | - value of the concentrated masses attached to the beam; |
| P0 = 76.8 tf | - amplitude value of the harmonic exciting force applied to one of the masses; |
| g = 10.00 m/s2 | - gravitational acceleration; |
| I = b·h3/12 = 0.017067 | - cross-sectional moment of inertia of the beam. |
The following values of frequencies of the harmonic exciting force ωi depending on the values of natural frequencies of the beam pi are considered:
ωj = 0.5·p1; 0.95·p1; 1.05·p1; 0.5·(p1+ p2); 0.95·p2; 1.05·p2; 1.5·p2.
Finite element model: Design model – plane frame, 32 bar elements of type 2. Boundary conditions of the simply supported ends of the beam are provided by imposing constraints in the direction of the degree of freedom Z. The dimensional stability of the design model is provided by imposing a constraint in the node of the cross-section along the symmetry axis of the beam in the direction of the degree of freedom X. The concentrated masses are specified by transforming the static nodal loads m·g.
The calculation is performed in two stages: first the natural oscillation modes and natural frequencies p are determined by the modal analysis, and then the deflections η in the cross-sections of the beam with the attached masses with time are determined by the direct integration of the equations of motion method. The action of the transverse harmonic exciting force is described by the graph of the load variation with time and is given in the form of a nodal force acting along the Z axis of the global coordinate system with the scale factor of 1.0 and the delay time 0.0 s. Intervals between the time points of the load variation graph are equal to Δtint = Tj/100, where Tj – period of the harmonic exciting force, and correspond to the integration step. When plotting the graph, the action of the transverse harmonic exciting force is taken as Pn = P0·cos(ωj·n·Δtint) at the time points n·Δtint. The duration of the process is equal to t = 2·Tj. Critical damping ratios for the 1-st and 2-nd natural frequencies are taken with the minimum value ξ = 0.0001. The conversion factor for the added static loading is equal to k = 0.981 (mass generation). Number of nodes in the design model – 33. The modal integration method is used in the calculation. The determination of the natural oscillation modes and natural frequencies is performed by the method of subspace iteration. The matrix of concentrated masses is used in the calculation.
Results in SCAD
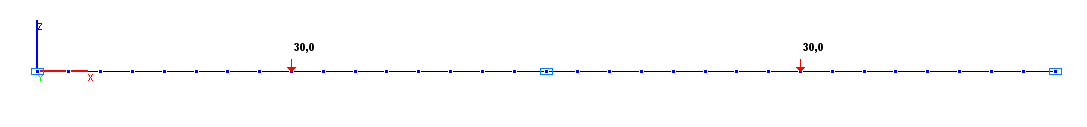
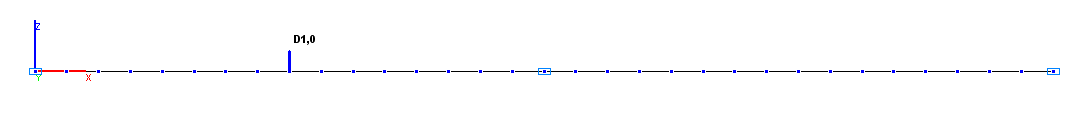
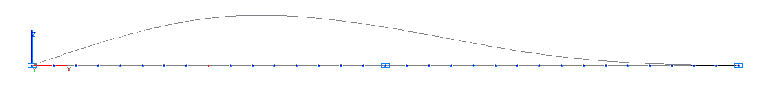
Design model
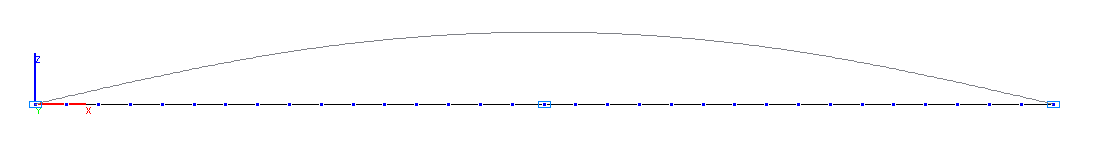
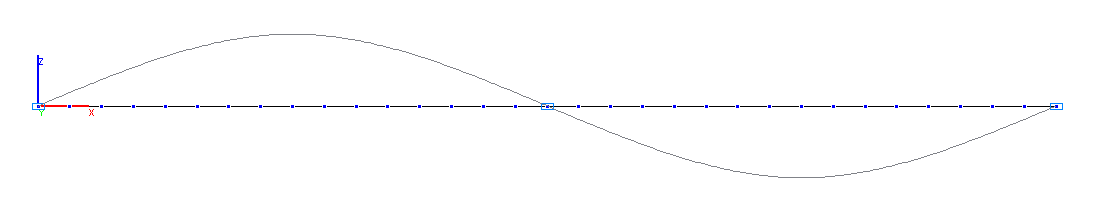
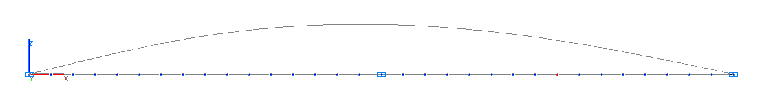
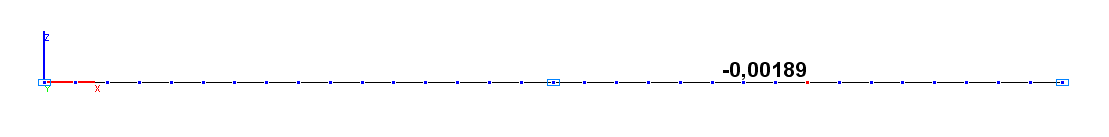
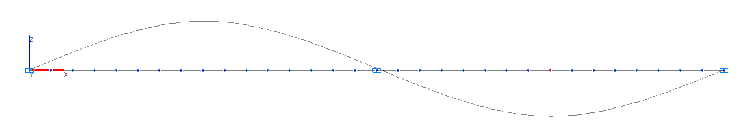
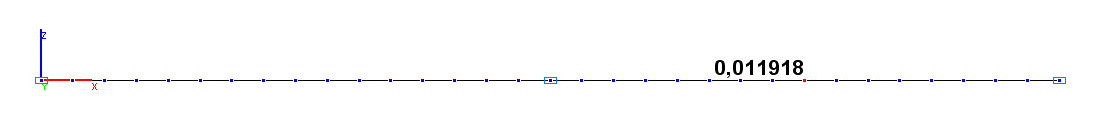
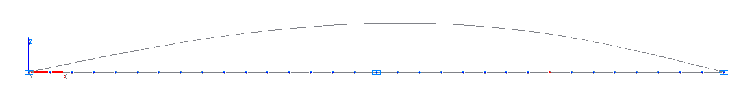
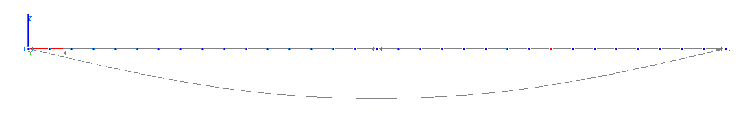
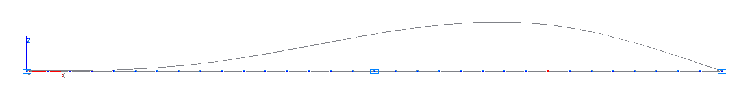
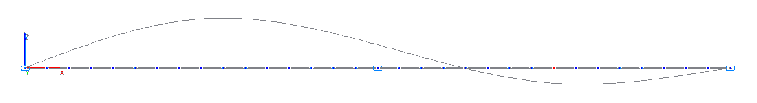
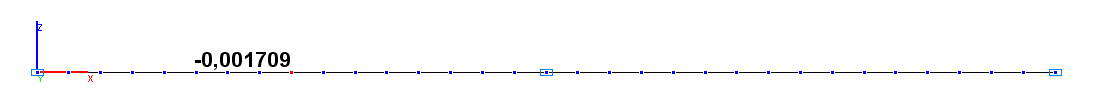
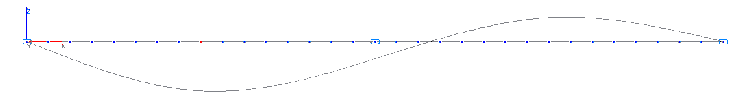
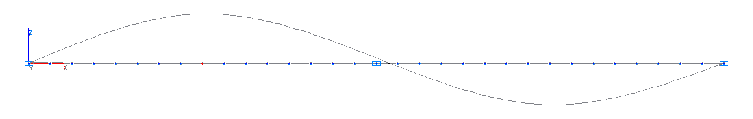
1-st and 2-nd natural oscillation modes
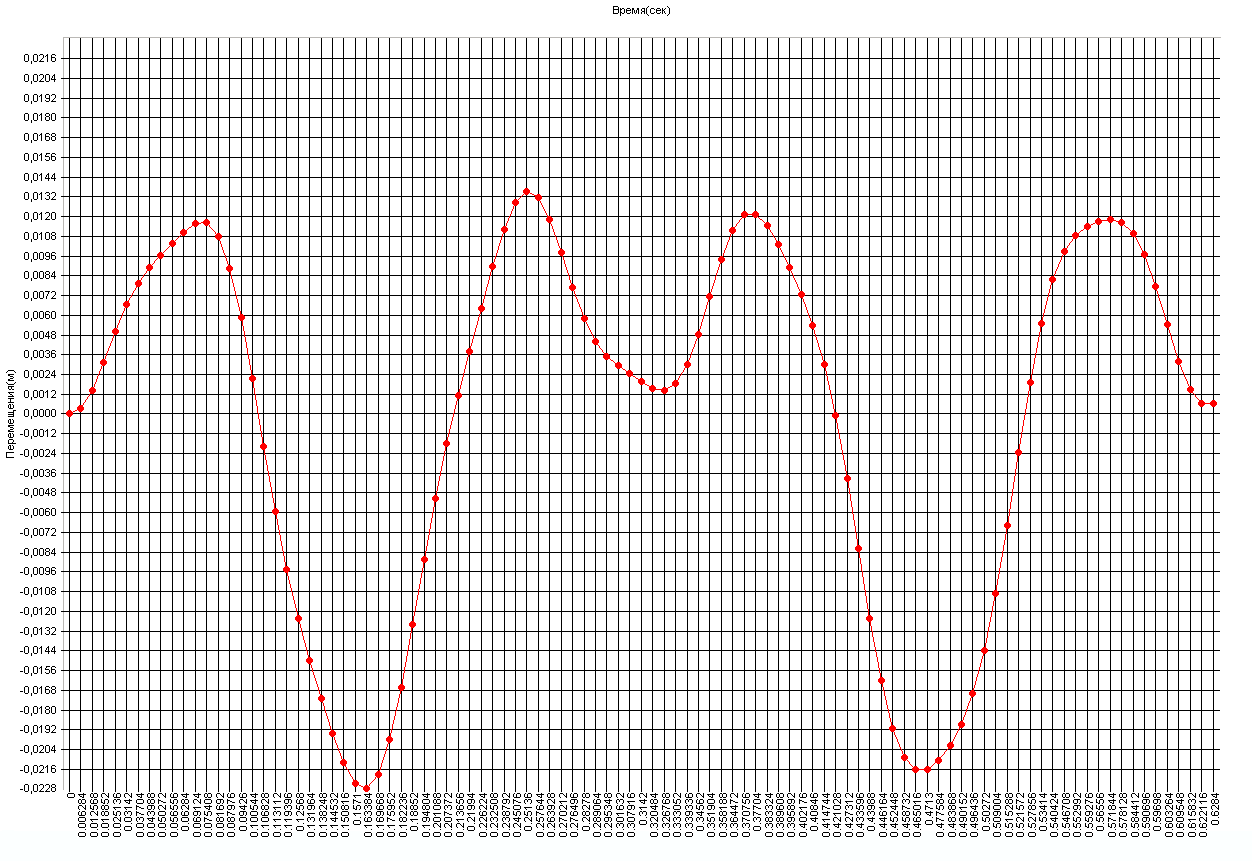
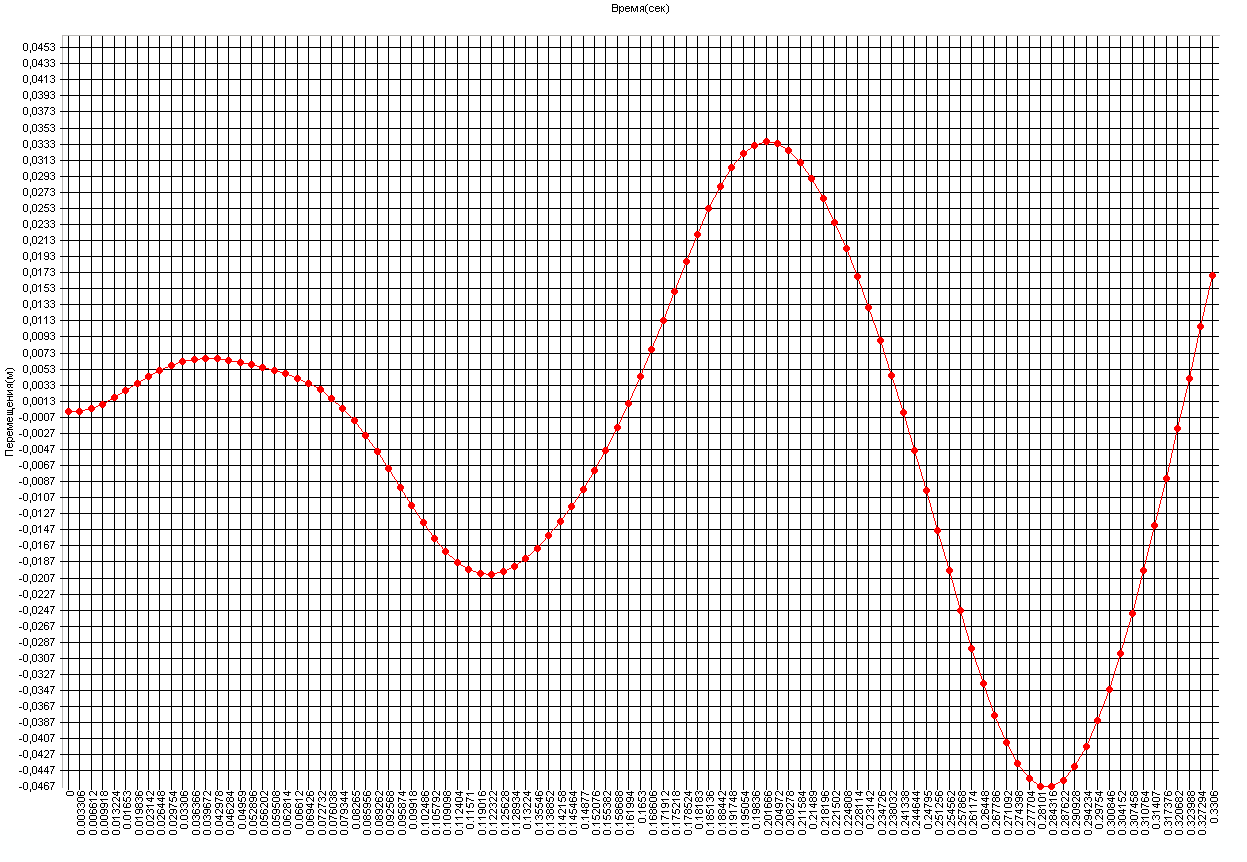
Graph of the variation of the deflection η1 in the cross-section of the beam with the attached mass subjected to the shear force with time (m).
Frequency of the harmonic exciting force ω1 = 0.5·p1

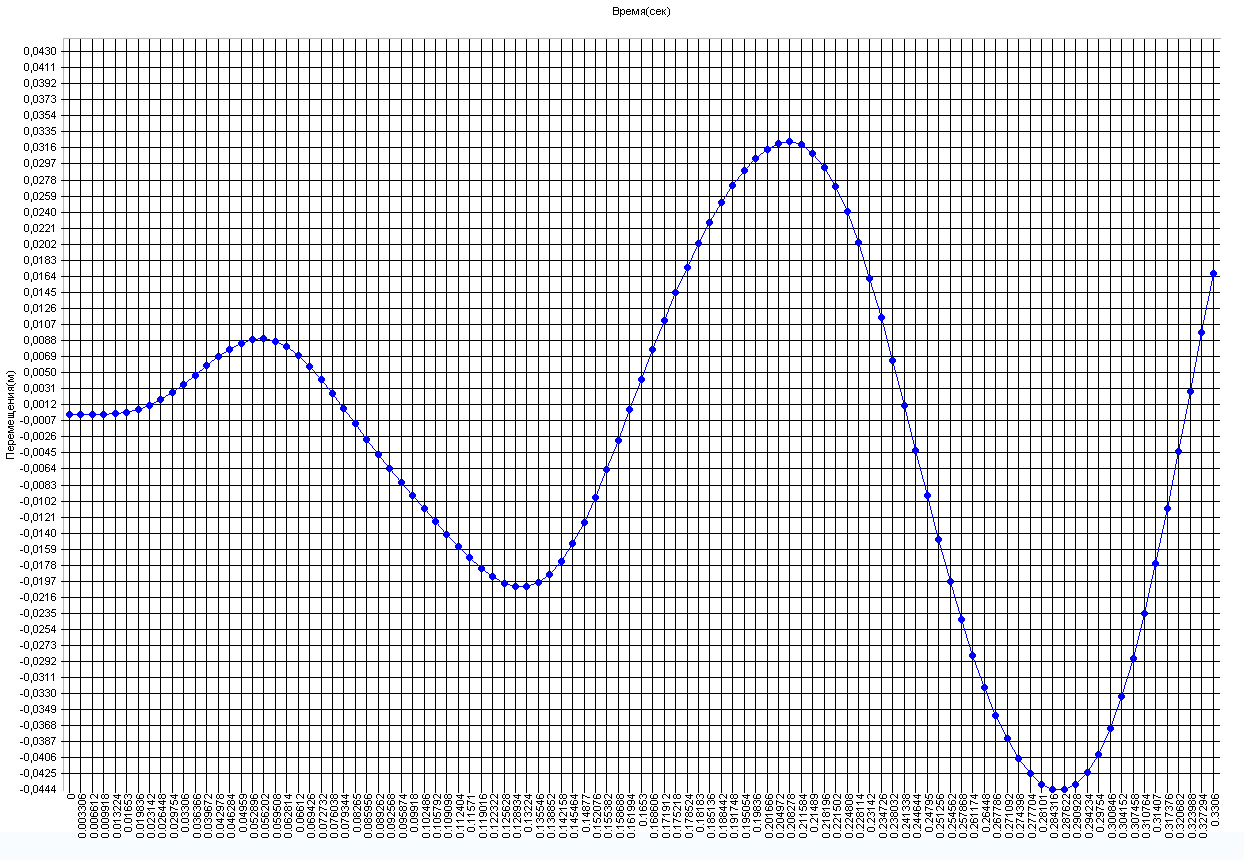
Graph of the variation of the deflection η2 in the cross-section of the beam
with the attached mass not subjected to the shear force with time (m).
Frequency of the harmonic exciting force ω1 = 0.5·p1
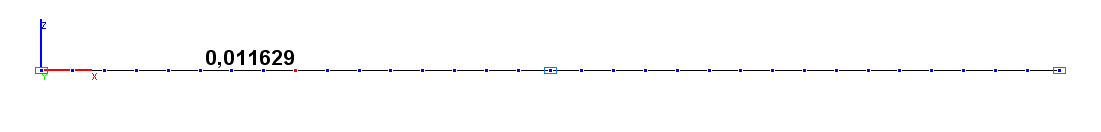
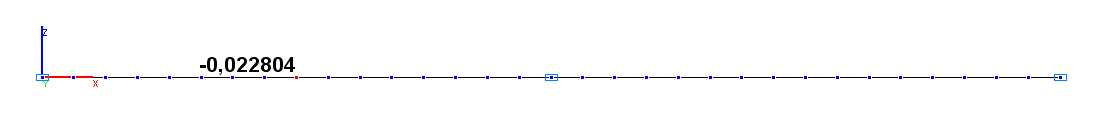




Amplitude values of the deflection η1 in the cross-section of the beam
with the attached mass subjected to the shear force
and the deformed models at the respective time points (m).
Frequency of the harmonic exciting force ω1 = 0.5·p1
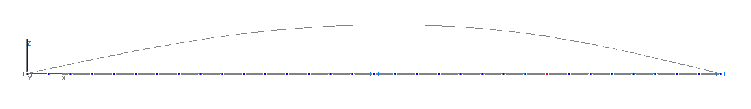
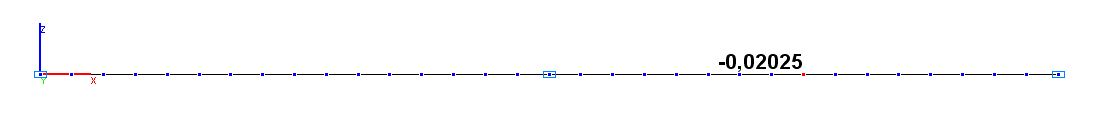
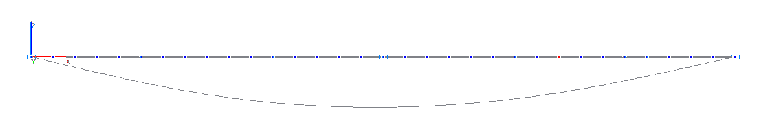
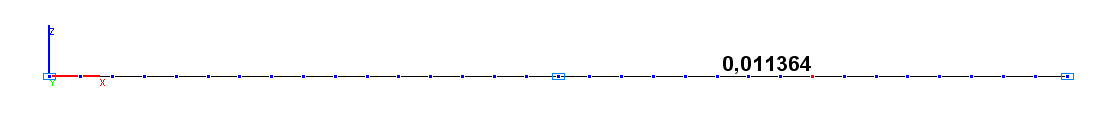
Amplitude values of the deflection η2 in the cross-section of the beam
with the attached mass not subjected to the shear force
and the deformed models at the respective time points (m). Frequency of the harmonic exciting force ω1 = 0.5·p1
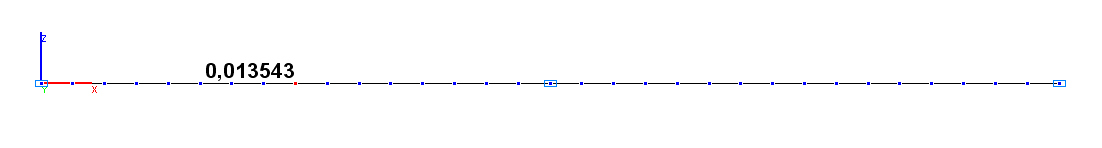
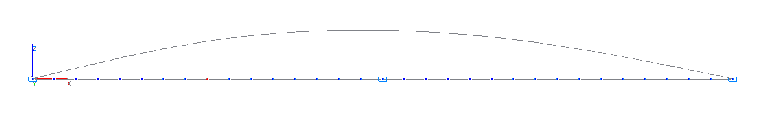
Amplitude value of the deflection η1 in the cross-section of the beam
with the attached mass subjected to the shear force
and the deformed model at the respective time point (m).
Frequency of the harmonic exciting force ω2 = 0.95·p1
Graph of the variation of the deflection η2 in the cross-section of the beam
with the attached mass not subjected to the shear force with time (m).
Frequency of the harmonic exciting force ω2 = 0.95·p1
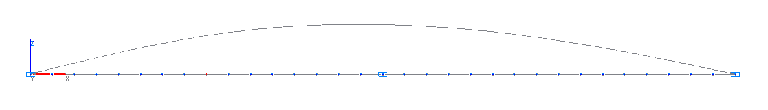
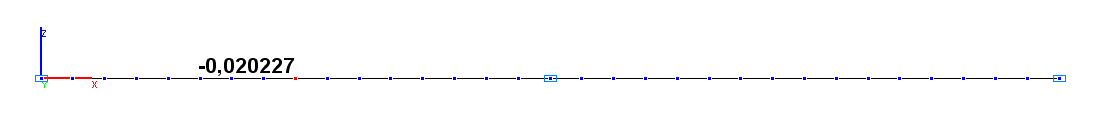
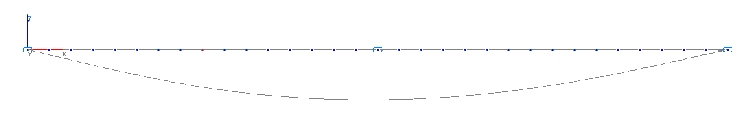
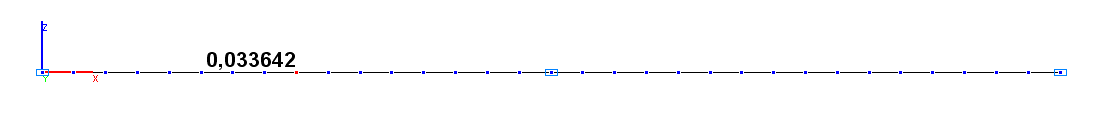
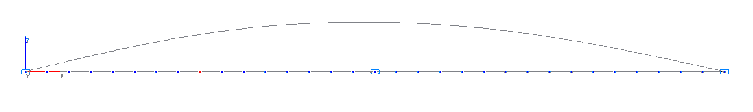
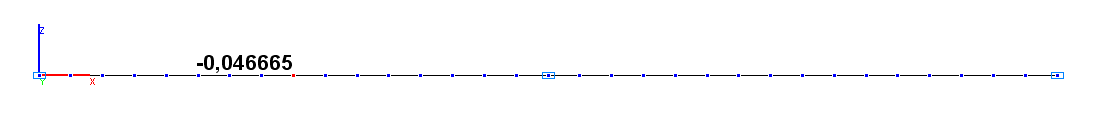
Amplitude values of the deflection η1 in the cross-section of the beam
with the attached mass subjected to the shear force
and the deformed models at the respective time points (m).
Frequency of the harmonic exciting force ω2 = 0.95·p1
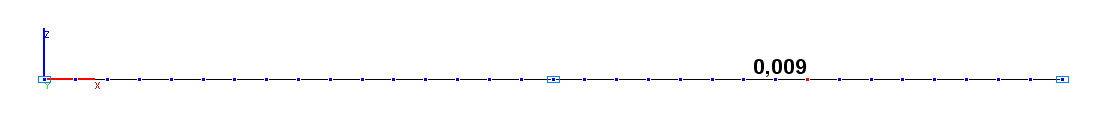
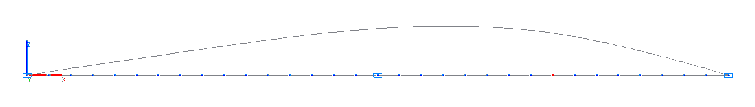
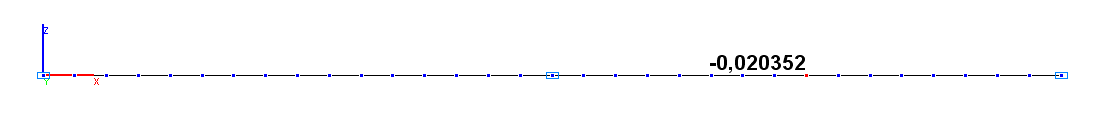
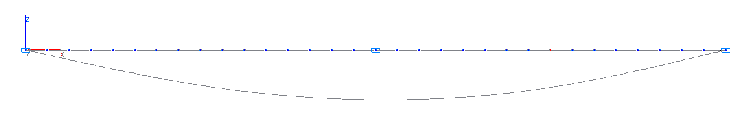


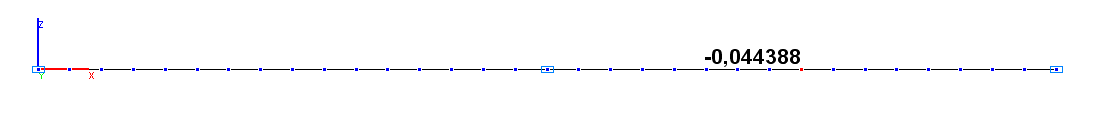

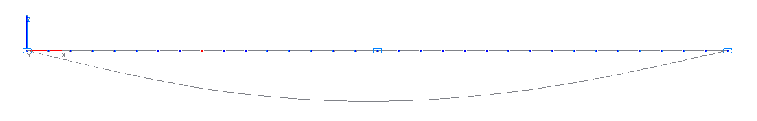
Amplitude values of the deflection η2 in the cross-section of the beam
with the attached mass not subjected to the shear force
and the deformed models at the respective time points (m).
Frequency of the harmonic exciting force ω2 = 0.95·p1
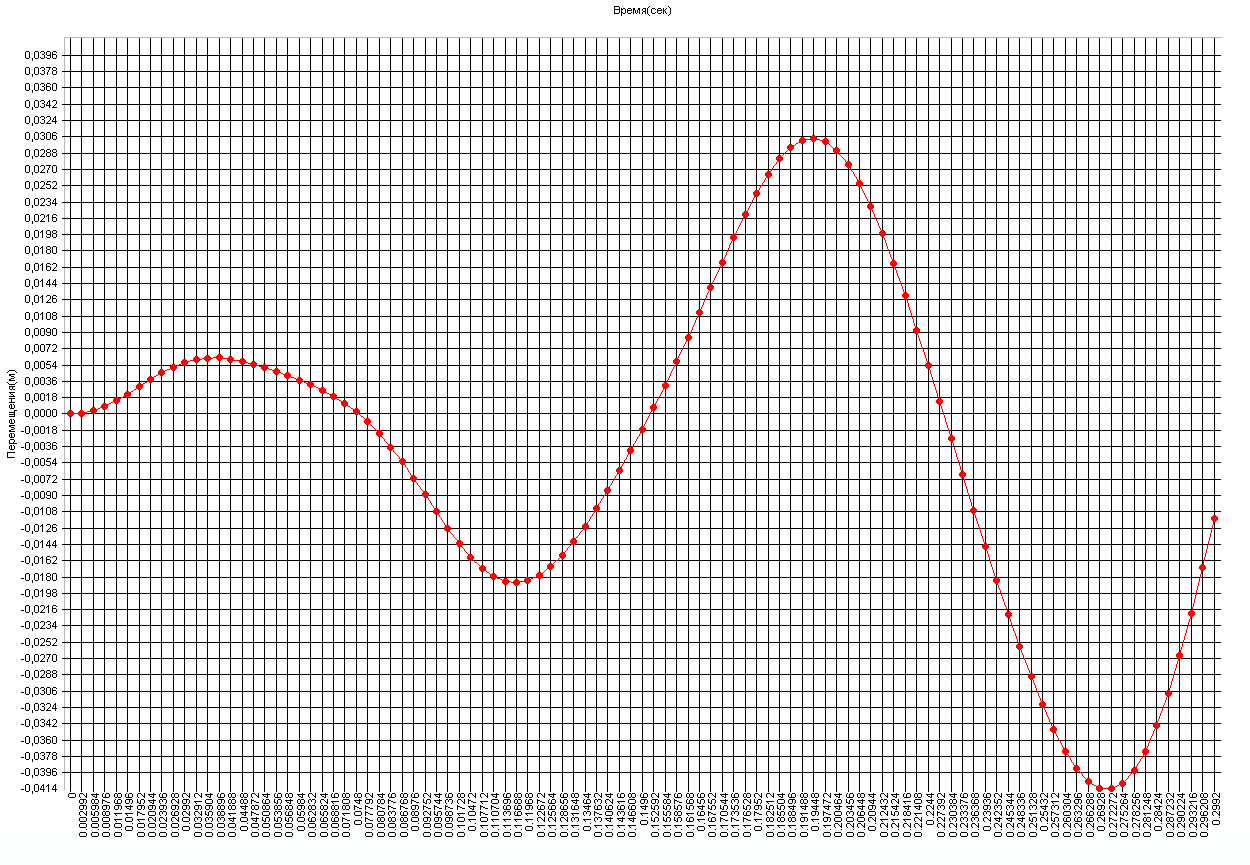
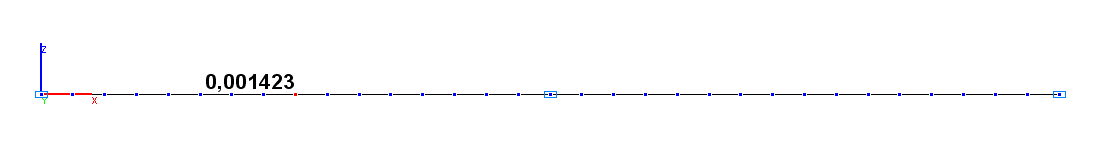
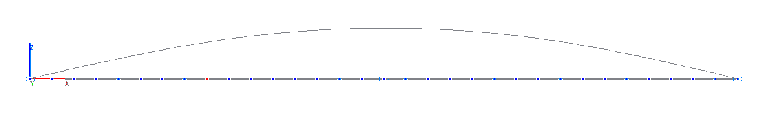
Amplitude value of the deflection η1 in the cross-section of the beam
with the attached mass subjected to the shear force
and the deformed model at the respective time point (m).
Frequency of the harmonic exciting force ω3 = 1.05·p1
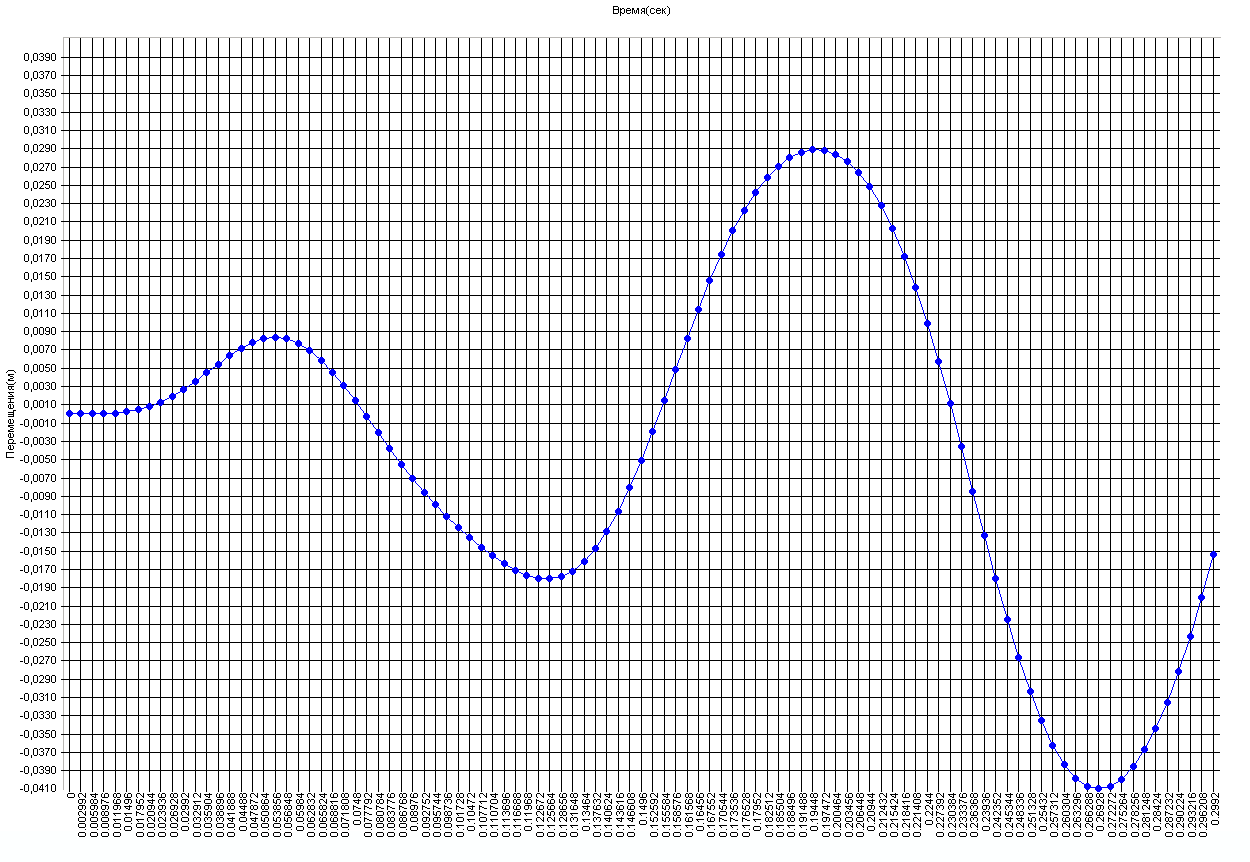
Graph of the variation of the deflection η2 in the cross-section of the beam
with the attached mass not subjected to the shear force, with time (m).
Frequency of the harmonic exciting force ω3 = 1.05·p1



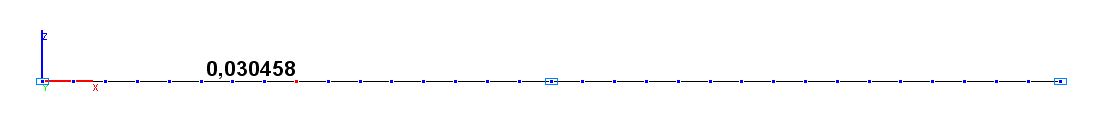
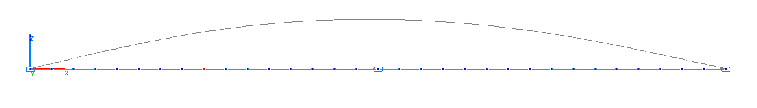
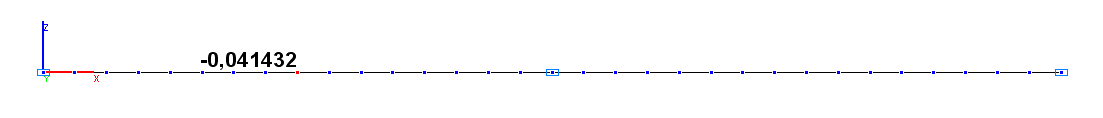

Amplitude values of the deflection η1 in the cross-section of the beam
with the attached mass subjected to the shear force
and the deformed models at the respective time points (m).
Frequency of the harmonic exciting force ω3 = 1.05·p1
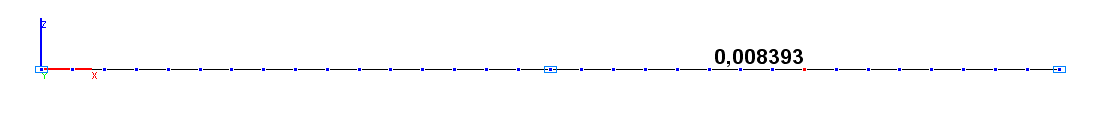
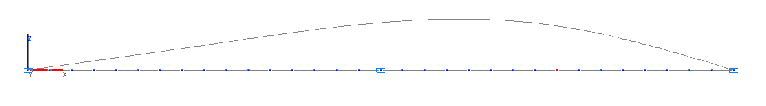
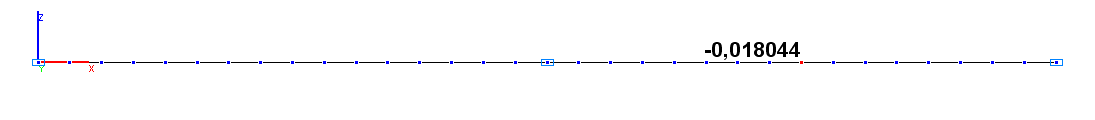
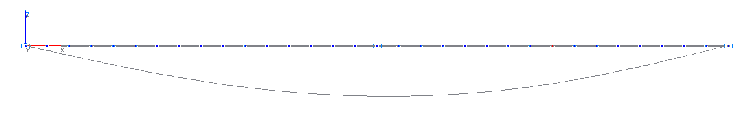
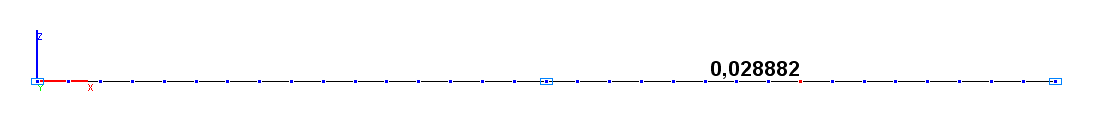


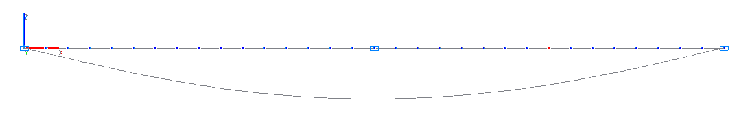
Amplitude values of the deflection η2 in the cross-section of the beam
with the attached mass not subjected to the shear force
and the deformed models at the respective time points (m).
Frequency of the harmonic exciting force ω3 = 1.05·p1
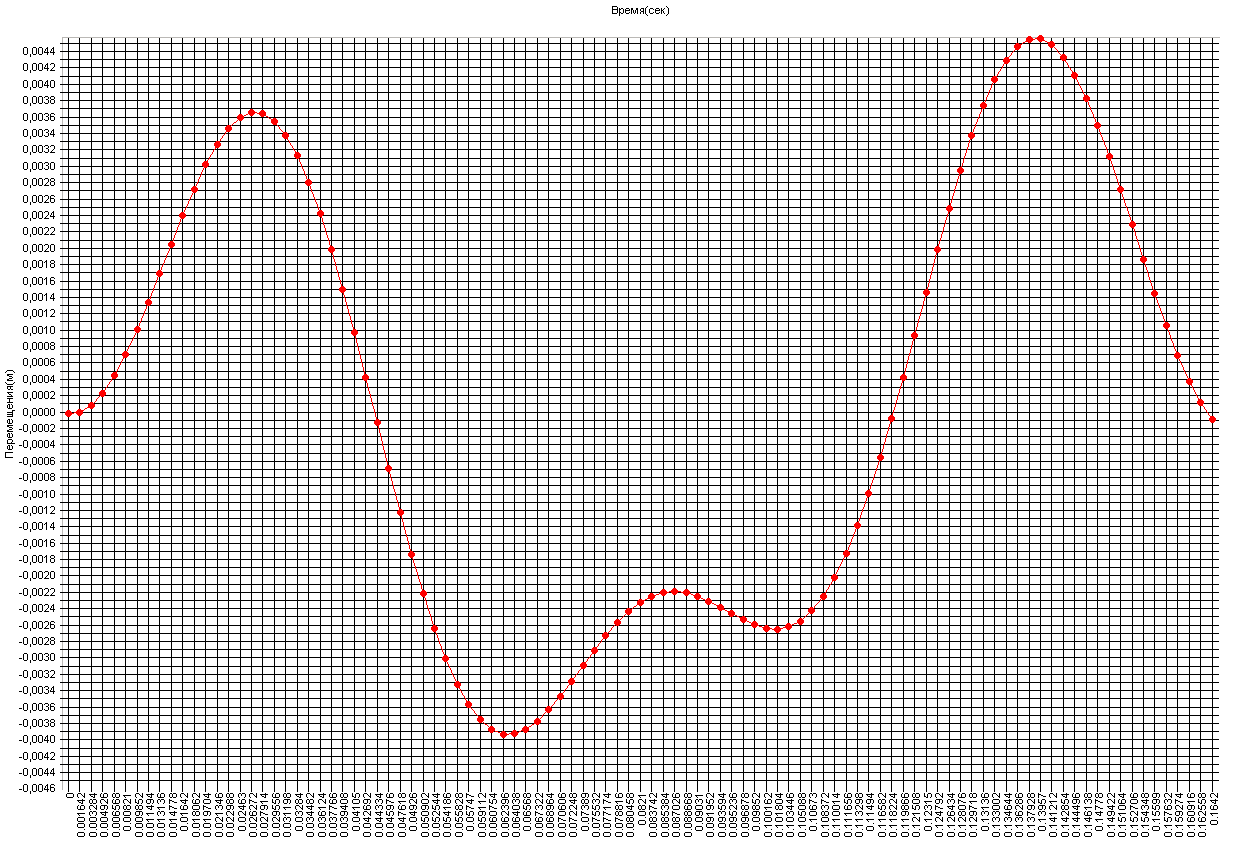
Amplitude value of the deflection η1 in the cross-section of the beam
with the attached mass subjected to the shear force
and the deformed model at the respective time point (m).
Frequency of the harmonic exciting force ω4 = 0.5·(p1+ p2)
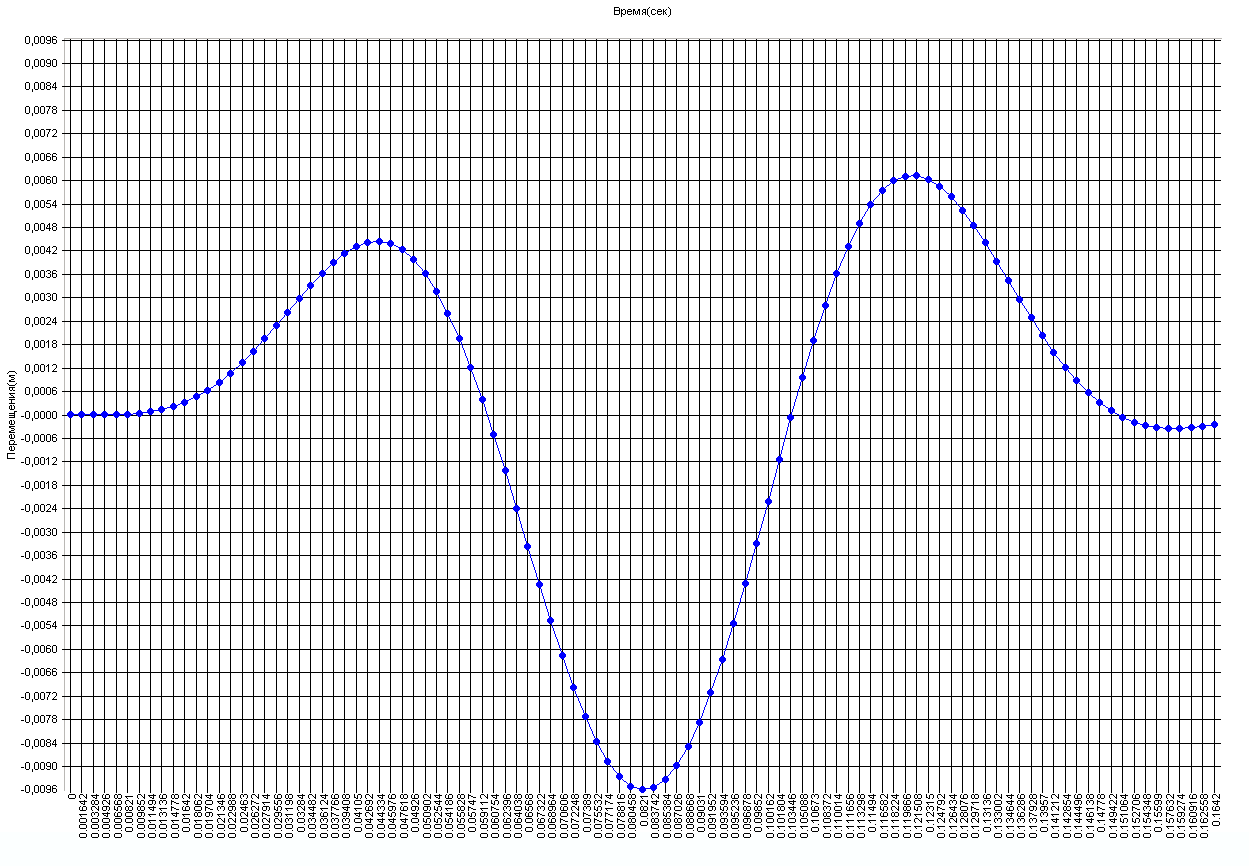
Graph of the variation of the deflection η2 in the cross-section of the beam
with the attached mass not subjected to the shear force, with time (m).
Frequency of the harmonic exciting force ω4 = 0.5·(p1+ p2)




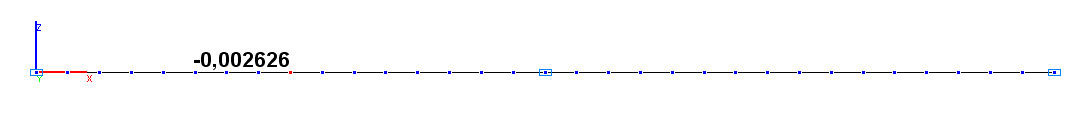
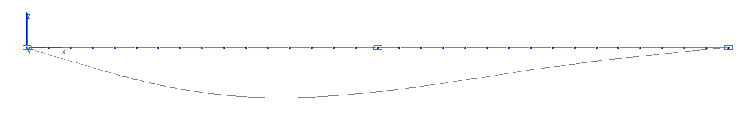
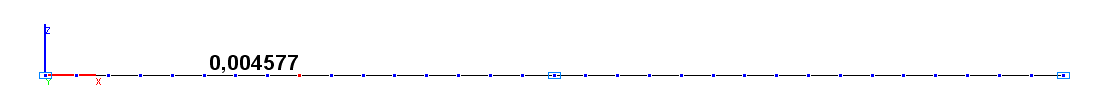
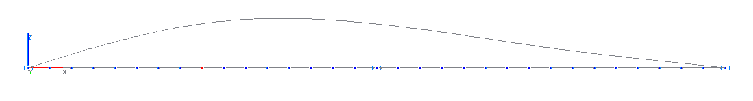
Amplitude values of the deflection η1 in the cross-section of the beam
with the attached mass subjected to the shear force
and the deformed models at the respective time points (m).
Frequency of the harmonic exciting force ω4 = 0.5·(p1+ p2)

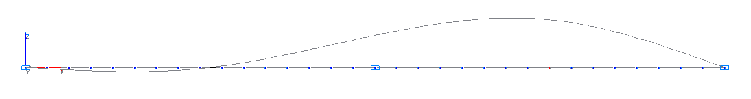
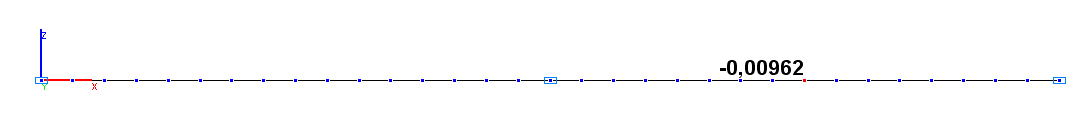
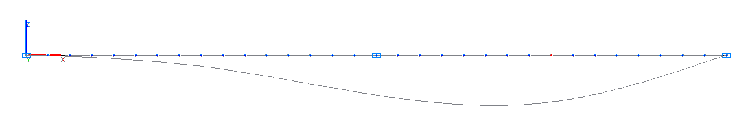
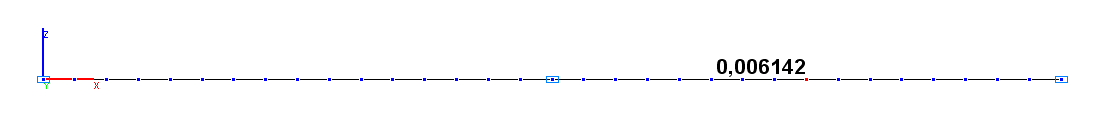

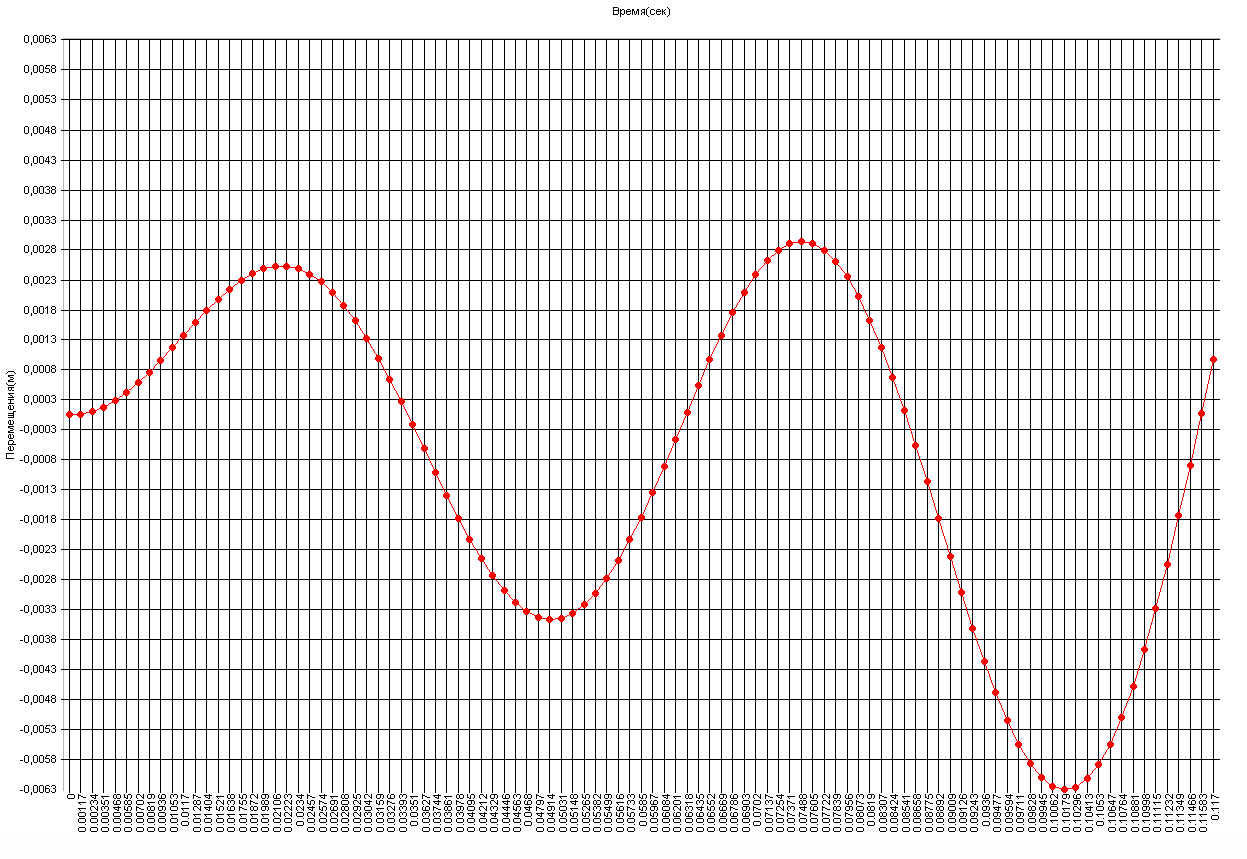
Amplitude values of the deflection η2 in the cross-section of the beam
with the attached mass not subjected to the shear force
and the deformed models at the respective time points (m).
Frequency of the harmonic exciting force ω4 = 0.5·(p1+ p2)
Amplitude value of the deflection η1 in the cross-section of the beam
with the attached mass subjected to the shear force
and the deformed model at the respective time point (m).
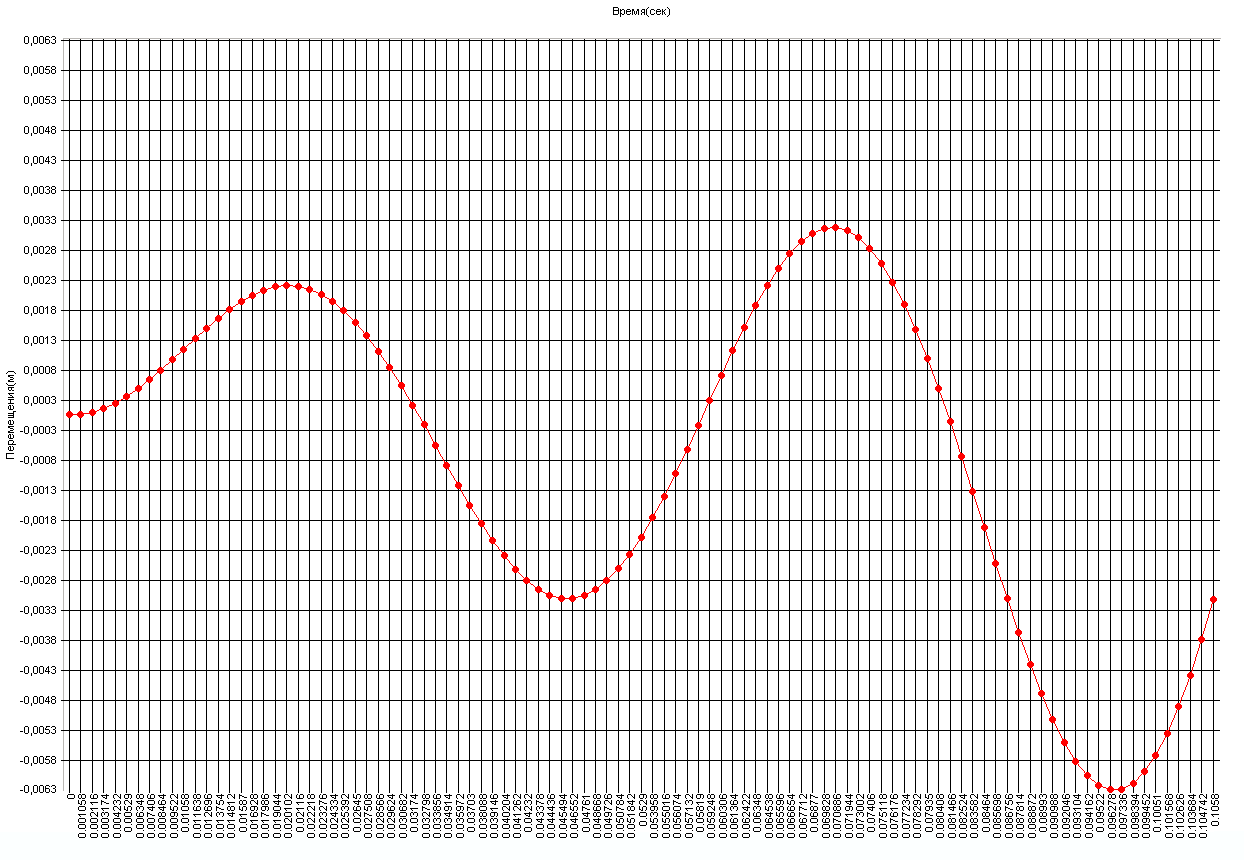
Frequency of the harmonic exciting force ω5 = 0.95·p2
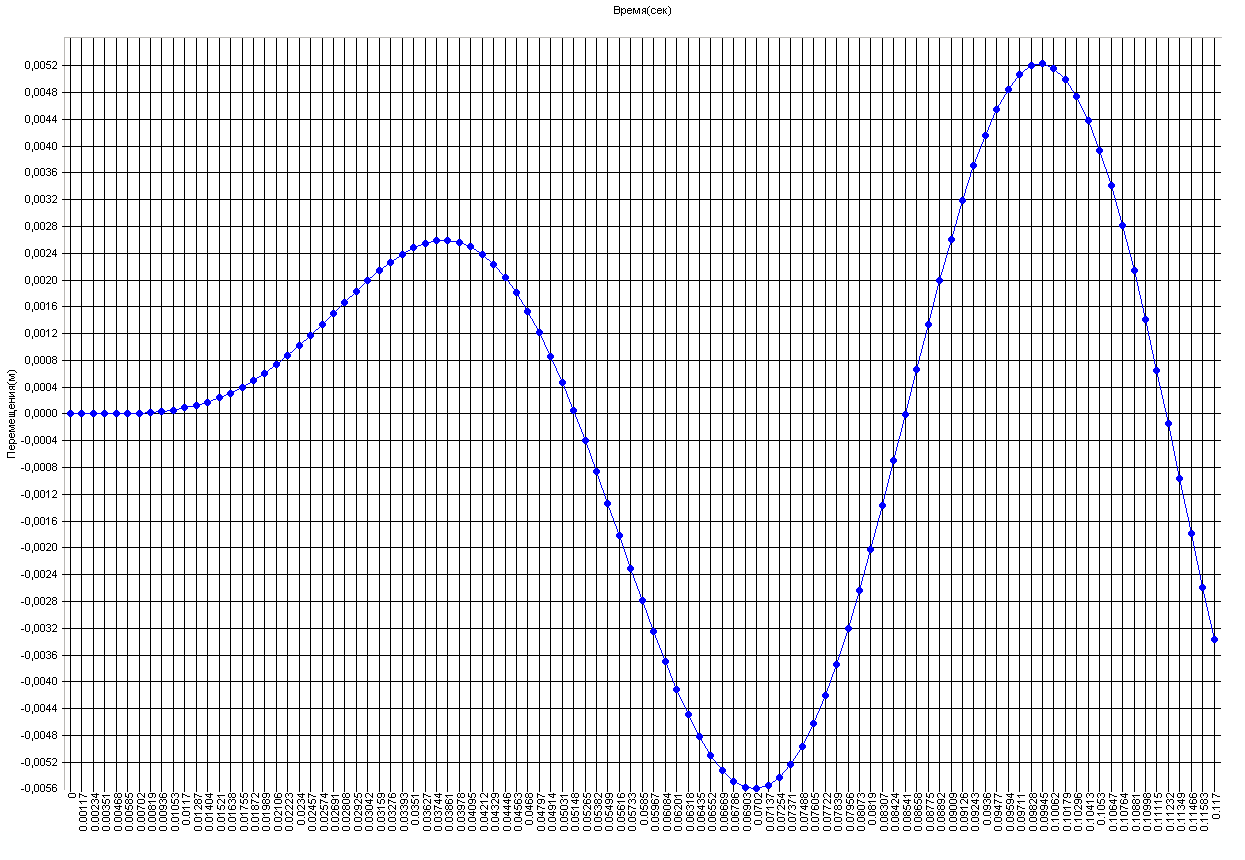
Graph of the variation of the deflection η2 in the cross-section of the beam
with the attached mass not subjected to the shear force, with time (m).
Frequency of the harmonic exciting force ω5 = 0.95·p2
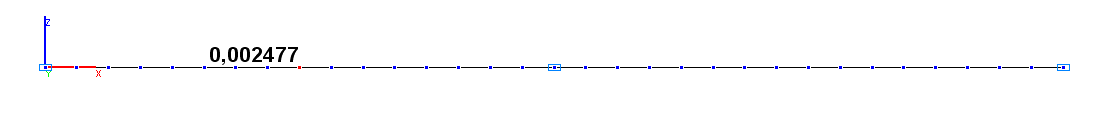


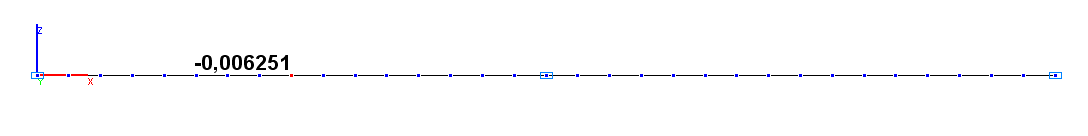
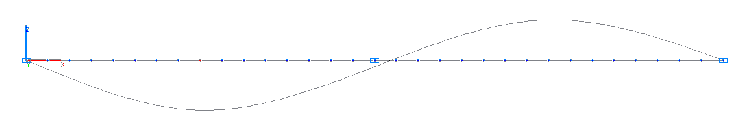
Amplitude values of the deflection η1 in the cross-section of the beam
with the attached mass subjected to the shear force
and the deformed models at the respective time points (m).
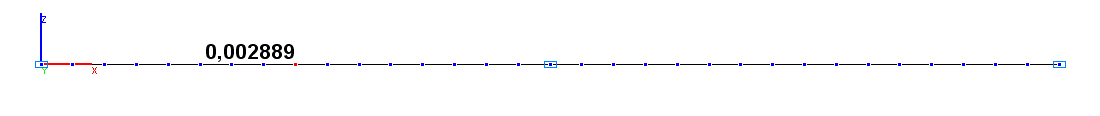
Frequency of the harmonic exciting force ω5 = 0.95·p2
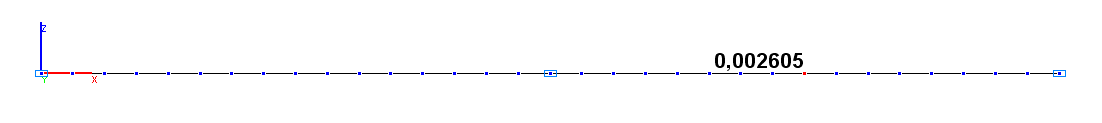
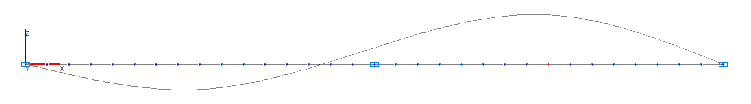
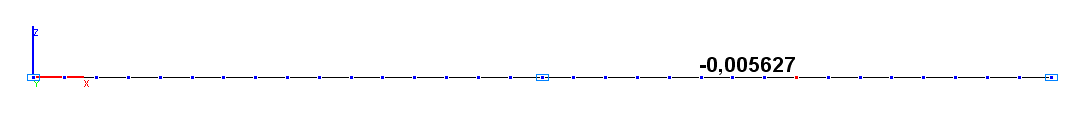


Amplitude values of the deflection η2 in the cross-section of the beam
with the attached mass not subjected to the shear force
and the deformed models at the respective time points (m).
Frequency of the harmonic exciting force ω5 = 0.95·p2
Amplitude value of the deflection η1 in the cross-section of the beam
with the attached mass subjected to the shear force
and the deformed model at the respective time point (m).
Frequency of the harmonic exciting force ω6 = 1.05·p2
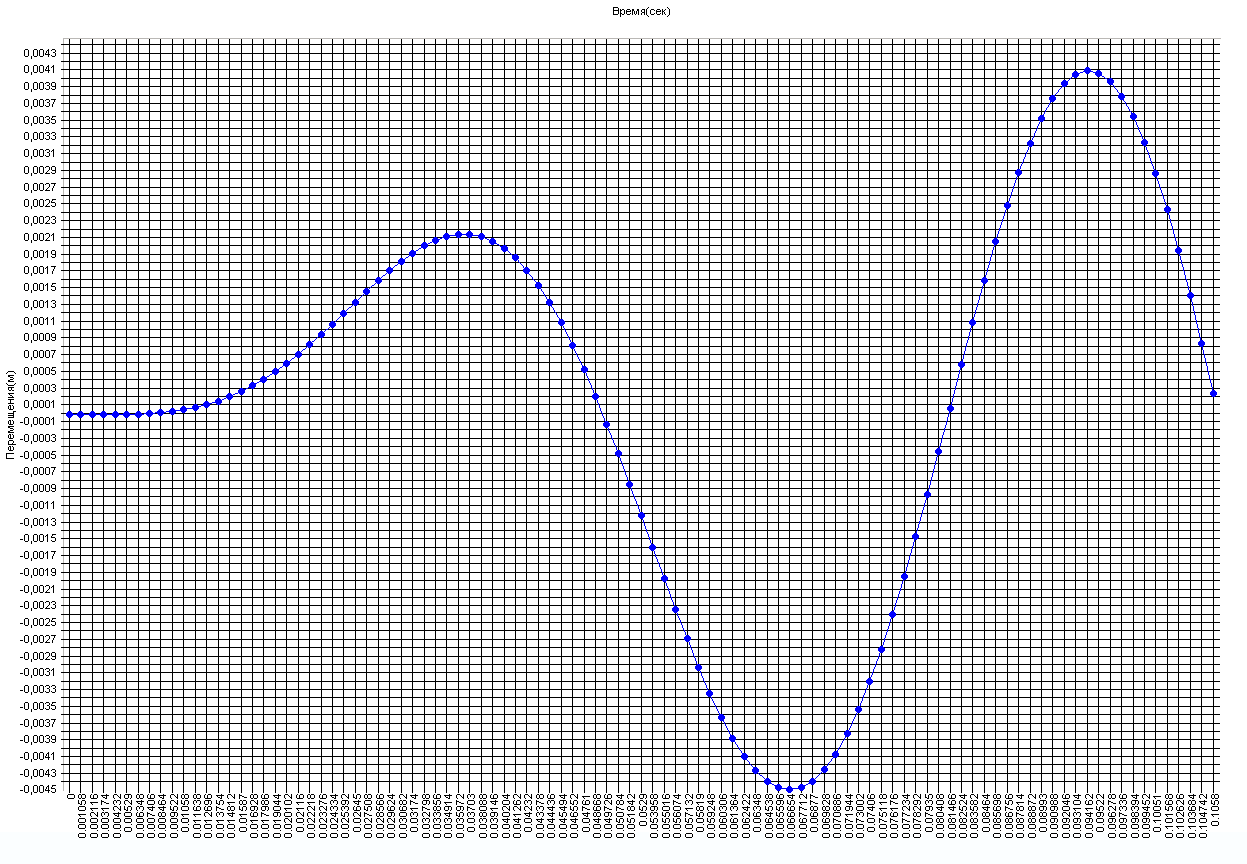
Graph of the variation of the deflection η2 in the cross-section of the beam
with the attached mass not subjected to the shear force, with time (m).
Frequency of the harmonic exciting force ω6 = 1.05·p2
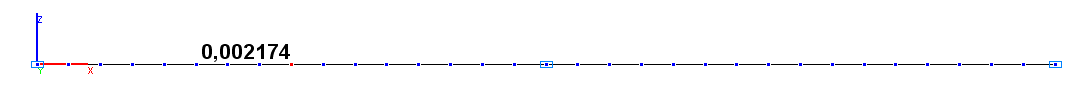
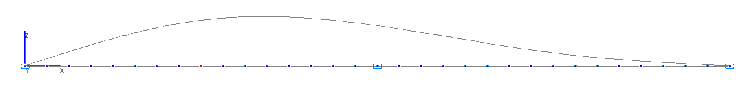



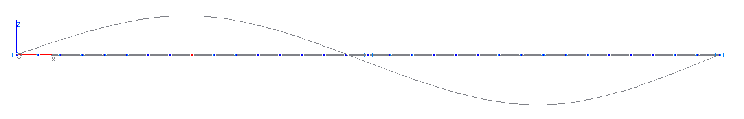

Amplitude values of the deflection η1 in the cross-section of the beam
with the attached mass subjected to the shear force
and the deformed models at the respective time points (m).
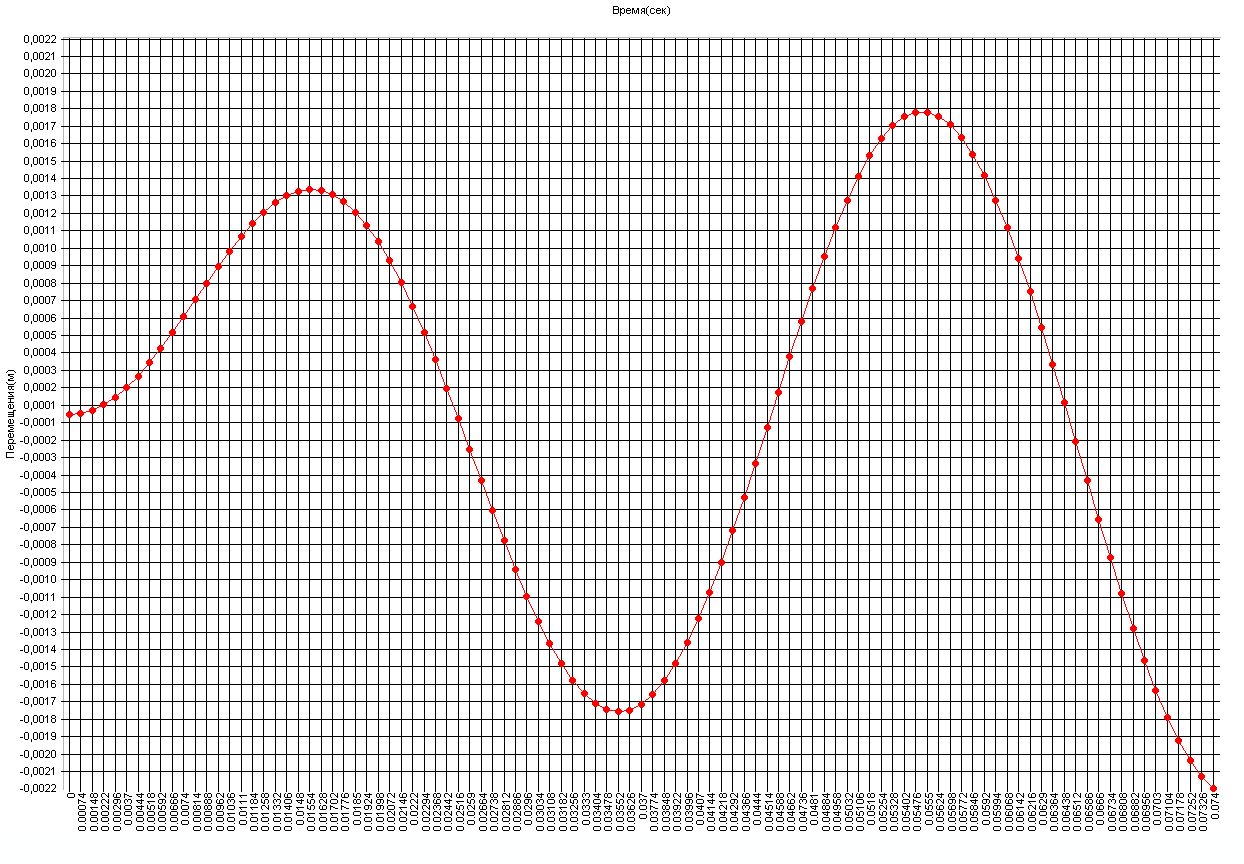
Frequency of the harmonic exciting force ω6 = 1.05·p2

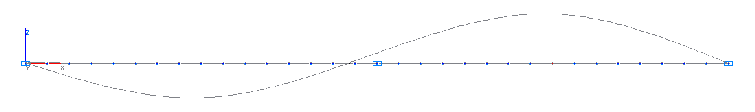


Amplitude values of the deflection η2 in the cross-section of the beam
with the attached mass not subjected to the shear force
and the deformed models at the respective time points (m).
Frequency of the harmonic exciting force ω6 = 1.05·p2
Amplitude value of the deflection η1 in the cross-section of the beam
with the attached mass subjected to the shear force
and the deformed model at the respective time point (m).
Frequency of the harmonic exciting force ω7 = 1.5·p2
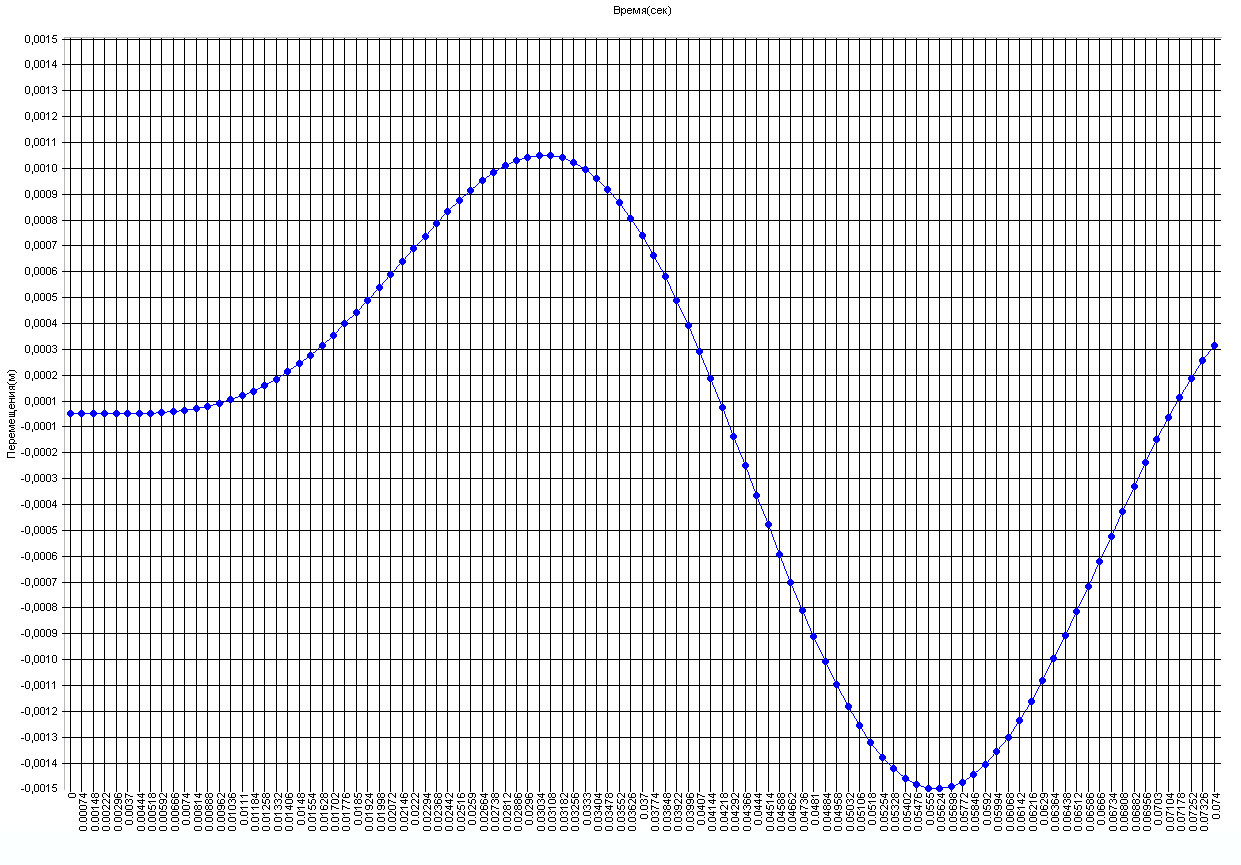
Graph of the variation of the deflection η2 in the cross-section of the beam
with the attached mass not subjected to the shear force with time (m).
Frequency of the harmonic exciting force ω7 = 1.5·p2


Amplitude values of the deflection η1 in the cross-section of the beam
with the attached mass subjected to the shear force
and the deformed models at the respective time points (m).
Frequency of the harmonic exciting force ω7 = 1.5·p2


Amplitude values of the deflection η2 in the cross-section of the beam
with the attached mass not subjected to the shear force
and the deformed models at the respective time points (m).
Frequency of the harmonic exciting force ω7 = 1.5·p2
Comparison of solutions:
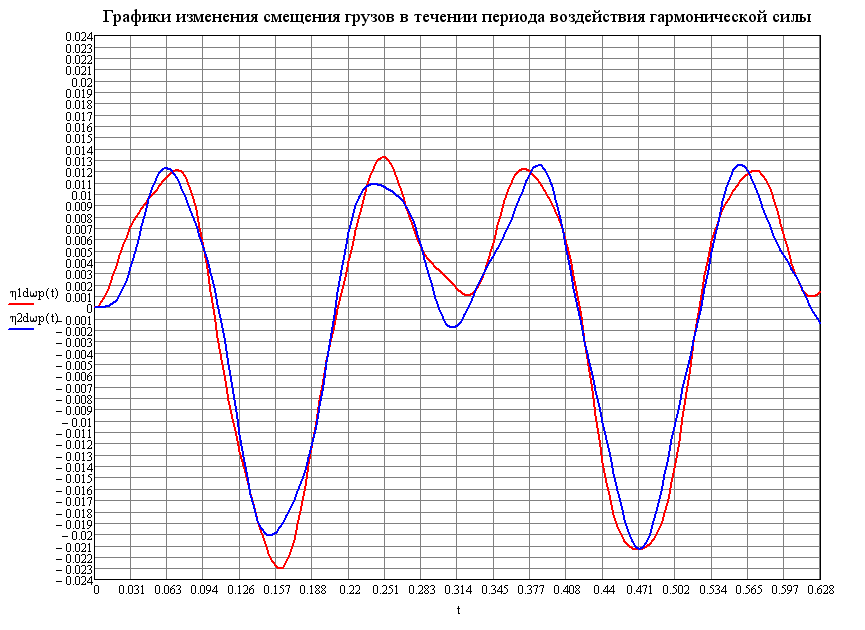
Graphs of the variation of the deflections η1 and η2 in the cross-sections of the beam
with the attached masses with time according to the theoretical solution (m)
Frequency of the harmonic exciting force ω1 = 0.5·p1
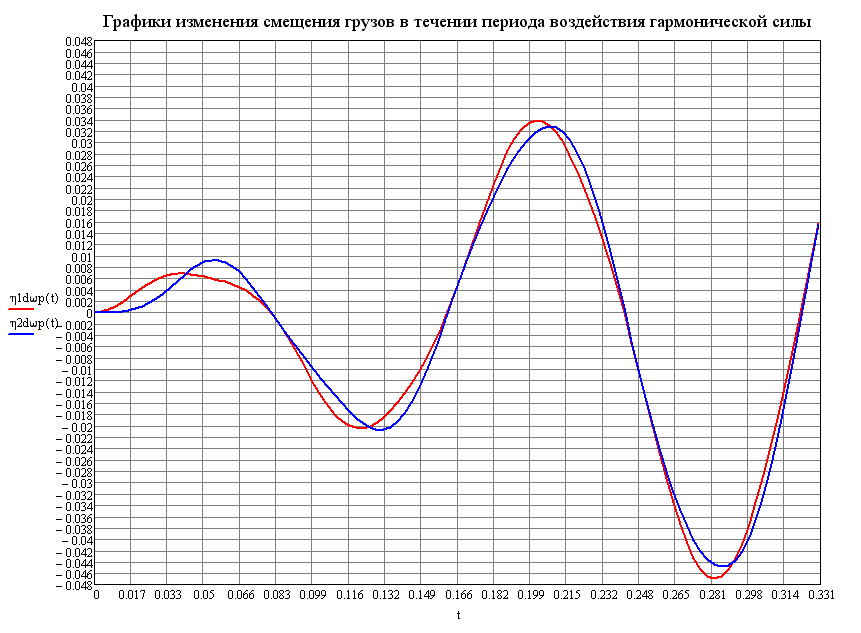
Graphs of the variation of the deflections η1 and η2 in the cross-sections of the beam
with the attached masses with time according to the theoretical solution (m)
Frequency of the harmonic exciting force ω2 = 0.95·p1
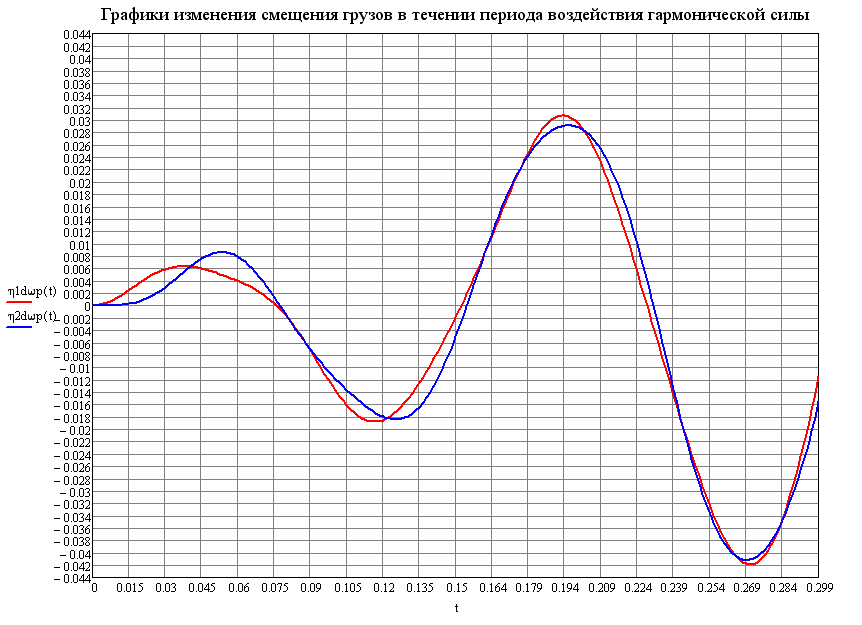
Graphs of the variation of the deflections η1 and η2 in the cross-sections of the beam
with the attached masses with time according to the theoretical solution (m)
Frequency of the harmonic exciting force ω3 = 1.05·p1
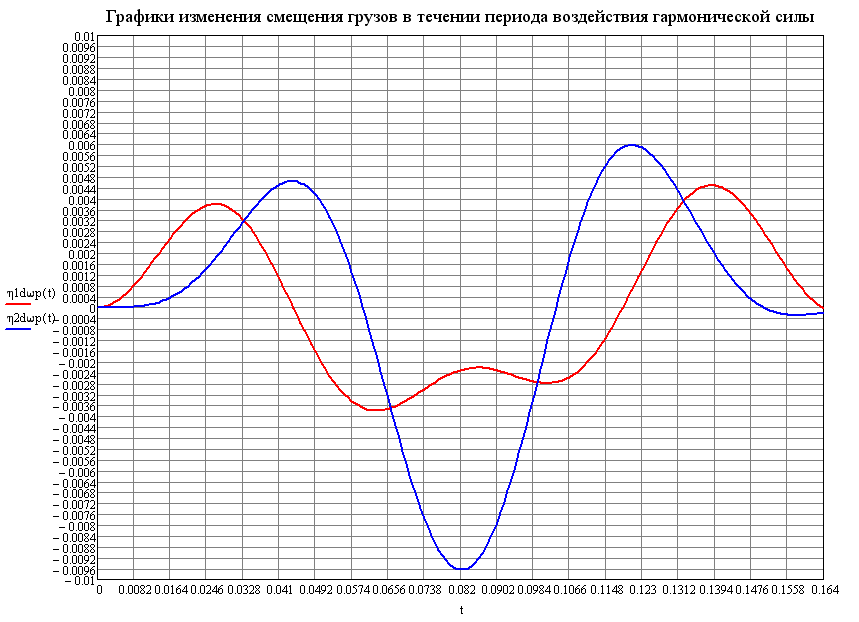
Graphs of the variation of the deflections η1 and η2 in the cross-sections of the beam
with the attached masses with time according to the theoretical solution (m)
Frequency of the harmonic exciting force ω4 = 0.5·(p1+ p2)
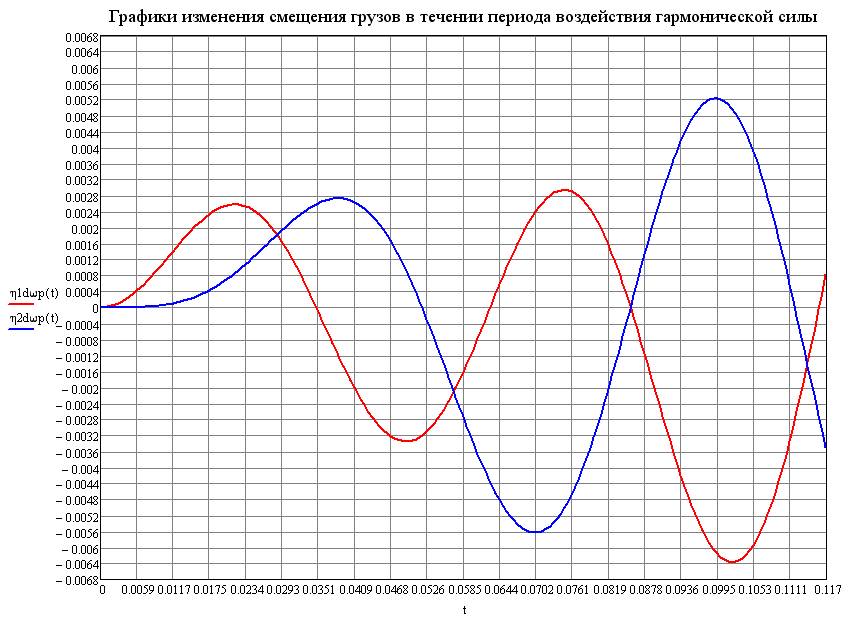
Graphs of the variation of the deflections η1 and η2 in the cross-sections of the beam
with the attached masses with time according to the theoretical solution (m)
Frequency of the harmonic exciting force ω5 = 0.95·p2
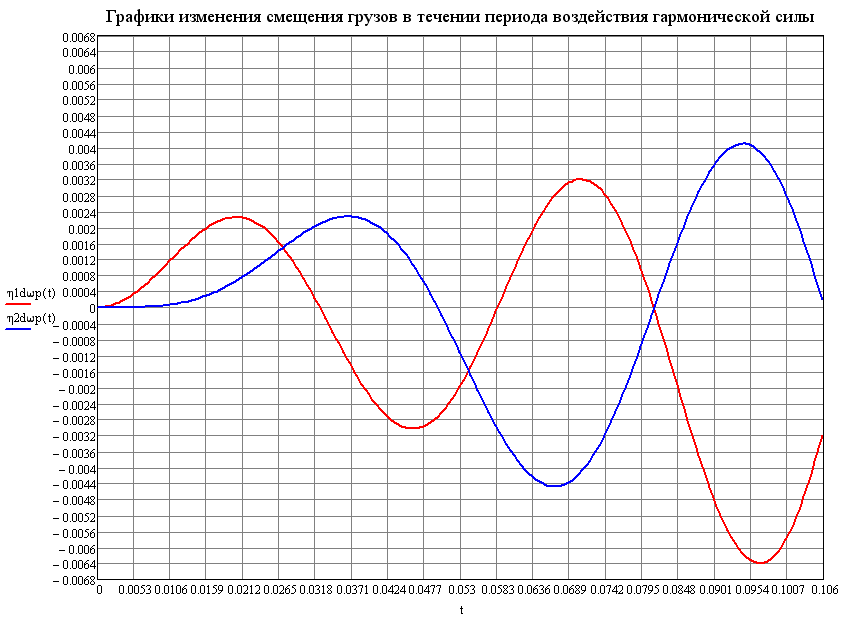
Graphs of the variation of the deflections η1 and η2 in the cross-sections of the beam
with the attached masses with time according to the theoretical solution (m)
Frequency of the harmonic exciting force ω6 = 1.05·p2
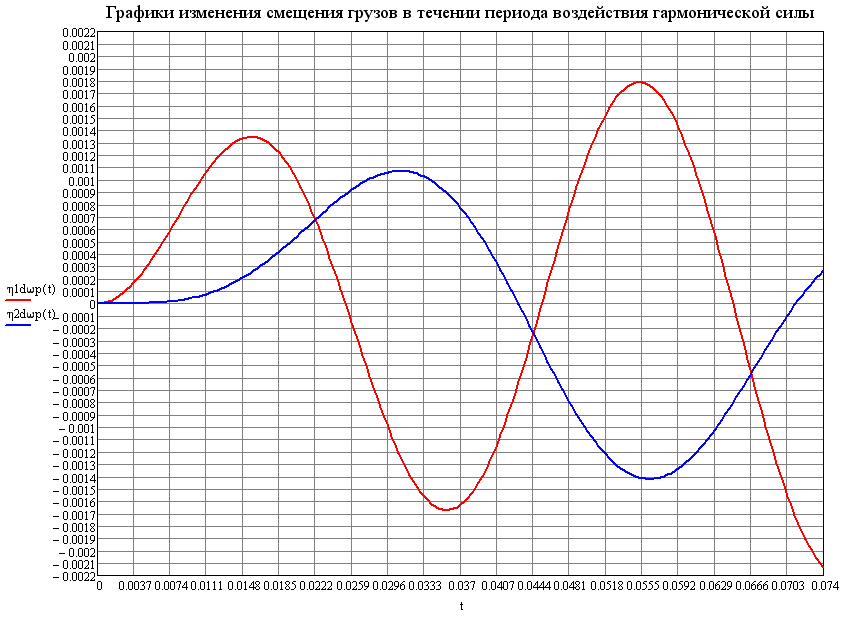
Graphs of the variation of the deflections η1 and η2 in the cross-sections of the beam
with the attached masses with time according to the theoretical solution (m)
Frequency of the harmonic exciting force ω7 = 1.5·p2
Natural frequencies p, rad/s
|
Oscillation mode |
Theory |
SCAD |
Deviations, % |
|---|---|---|---|
|
1 |
40.000 |
40.000 |
0.00 |
|
2 |
113.137 |
113.137 |
0.00 |
Amplitude values of the deflections η in the cross-sections of the beam
with the attached masses
at the frequency of the harmonic exciting force ω1 = 0.5·p1
|
Nodal mass |
Theory |
SCAD |
|||
|---|---|---|---|---|---|
|
Time, s |
Deflection, m |
Time, s |
Deflection, m |
Deviations, % |
|
|
1 |
0.0715 |
0.012129 |
0.0754 |
0.011629 |
4.12 |
|
1 |
0.0161 |
-0.023034 |
0.1634 |
-0.022804 |
1.00 |
|
1 |
0.2497 |
0.013302 |
0.2514 |
0.013543 |
1.81 |
|
1 |
0.3230 |
0.001094 |
0.3268 |
0.001423 |
─ |
|
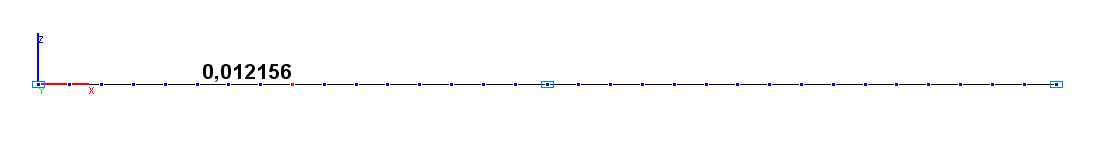
1 |
0.3714 |
0.012253 |
0.3770 |
0.012156 |
0.79 |
|
1 |
0.4700 |
-0.021344 |
0.4650 |
-0.021635 |
1.36 |
|
1 |
0.5714 |
0.012072 |
0.5718 |
0.011818 |
2.10 |
|
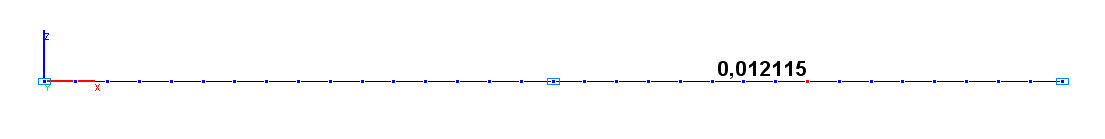
2 |
0.0621 |
0.012314 |
0.0628 |
0.012115 |
1.62 |
|
2 |
0.1515 |
-0.020119 |
0.1508 |
-0.020250 |
0.65 |
|
2 |
0.2412 |
0.010905 |
0.2451 |
0.011364 |
4.21 |
|
2 |
0.3099 |
-0.001817 |
0.3142 |
-0.001890 |
─ |
|
2 |
0.3845 |
0.012586 |
0.3896 |
0.011918 |
5.31 |
|
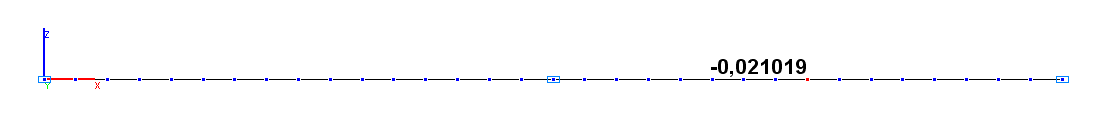
2 |
0.4716 |
-0.021331 |
0.4776 |
-0.021019 |
1.46 |
|
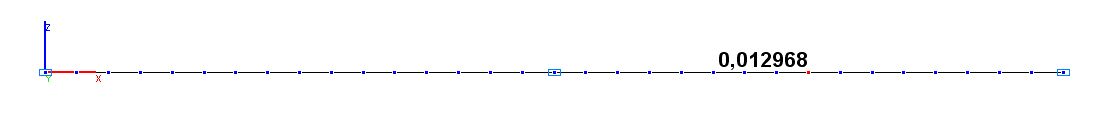
2 |
0.5586 |
0.012664 |
0.5656 |
0.012968 |
2.40 |
Amplitude values of the deflections η in the cross-sections of the beam
with the attached masses
at the frequency of the harmonic exciting force ω2 = 0.95·p1
|
Nodal mass |
Theory |
SCAD |
|||
|---|---|---|---|---|---|
|
Time, s |
Deflection, m |
Time, s |
Deflection, m |
Deviations, % |
|
|
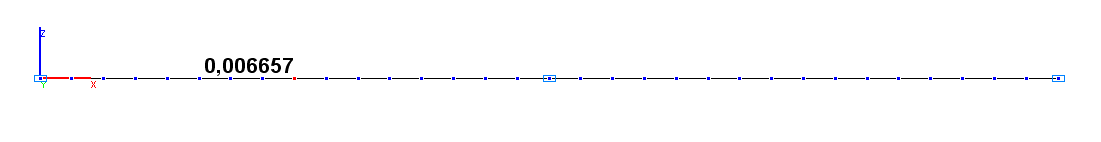
1 |
0.0398 |
0.006829 |
0.0397 |
0.006657 |
2.52 |
|
1 |
0.1210 |
-0.020424 |
0.1223 |
-0.020227 |
0.96 |
|
1 |
0.2018 |
0.033848 |
0.2017 |
0.033642 |
0.61 |
|
1 |
0.2826 |
-0.046989 |
0.2843 |
-0.046665 |
0.69 |
|
2 |
0.0547 |
0.009260 |
0.0562 |
0.009000 |
2.81 |
|
2 |
0.1303 |
-0.020792 |
0.1289 |
-0.020352 |
2.12 |
|
2 |
0.2078 |
0.032790 |
0.2083 |
0.032331 |
1.40 |
|
2 |
0.2863 |
-0.044911 |
0.2876 |
-0.044388 |
1.16 |
Amplitude values of the deflections η in the cross-sections of the beam
with the attached masses
at the frequency of the harmonic exciting force ω3 = 1.05·p1
|
Nodal mass |
Theory |
SCAD |
|||
|---|---|---|---|---|---|
|
Time, s |
Deflection, m |
Time, s |
Deflection, m |
Deviations, % |
|
|
1 |
0.0374 |
0.006369 |
0.0389 |
0.006194 |
2.75 |
|
1 |
0.1161 |
-0.018850 |
0.1167 |
-0.018642 |
1.10 |
|
1 |
0.1938 |
0.030767 |
0.1945 |
0.030458 |
1.00 |
|
1 |
0.2710 |
-0.041915 |
0.2723 |
-0.041432 |
1.15 |
|
2 |
0.0534 |
0.008636 |
0.0539 |
0.008393 |
2.81 |
|
2 |
0.1249 |
-0.018428 |
0.1257 |
-0.018044 |
1.76 |
|
2 |
0.1959 |
0.029186 |
0.1945 |
0.028882 |
1.04 |
|
2 |
0.2695 |
-0.041172 |
0.2693 |
-0.041014 |
0.38 |
Amplitude values of the deflections η in the cross-sections of the beam
with the attached masses
at the frequency of the harmonic exciting force ω4 = 0.5·(p1+ p2)
|
Nodal mass |
Theory |
SCAD |
|||
|---|---|---|---|---|---|
|
Time, s |
Deflection, m |
Time, s |
Deflection, m |
Deviations, % |
|
|
1 |
0.0267 |
0.003807 |
0.0263 |
0.003681 |
3.31 |
|
1 |
0.0631 |
-0.003795 |
0.0624 |
-0.003906 |
2.92 |
|
1 |
0.0859 |
-0.002215 |
0.0870 |
-0.002164 |
2.30 |
|
1 |
0.1016 |
-0.002783 |
0.1018 |
-0.002626 |
5.64 |
|
1 |
0.1387 |
0.004497 |
0.1396 |
0.004577 |
1.78 |
|
2 |
0.0440 |
0.004643 |
0.0443 |
0.004462 |
3.90 |
|
2 |
0.0823 |
-0.009649 |
0.0821 |
-0.009620 |
0.30 |
|
2 |
0.1207 |
0.005968 |
0.1251 |
0.006142 |
2.92 |
|
2 |
0.1579 |
-0.000288 |
0.1576 |
-0.000339 |
─ |
Amplitude values of the deflections η in the cross-sections of the beam
with the attached masses
at the frequency of the harmonic exciting force ω5 = 0.95·p2
|
Nodal mass |
Theory |
SCAD |
|||
|---|---|---|---|---|---|
|
Time, s |
Deflection, m |
Time, s |
Deflection, m |
Deviations, % |
|
|
1 |
0.0216 |
0.002575 |
0.0222 |
0.002477 |
3.81 |
|
1 |
0.0492 |
-0.003347 |
0.0491 |
-0.003410 |
1.88 |
|
1 |
0.0747 |
0.002938 |
0.0749 |
0.002889 |
1.67 |
|
1 |
0.1018 |
-0.006366 |
0.1018 |
-0.006251 |
1.81 |
|
2 |
0.0383 |
0.002731 |
0.0386 |
0.002605 |
4.61 |
|
2 |
0.0700 |
-0.005629 |
0.0702 |
-0.005627 |
0.04 |
|
2 |
0.0991 |
0.005222 |
0.0995 |
0.005254 |
0.61 |
Amplitude values of the deflections η in the cross-sections of the beam
with the attached masses
at the frequency of the harmonic exciting force ω6 = 1.05·p2
|
Nodal mass |
Theory |
SCAD |
|||
|---|---|---|---|---|---|
|
Time, s |
Deflection, m |
Time, s |
Deflection, m |
Deviations, % |
|
|
1 |
0.0203 |
0.002260 |
0.0201 |
0.002174 |
3.81 |
|
1 |
0.0460 |
-0.003028 |
0.0466 |
-0.003076 |
1.59 |
|
1 |
0.0705 |
0.003206 |
0.0709 |
0.003142 |
2.00 |
|
1 |
0.0967 |
-0.006393 |
0.0963 |
-0.006294 |
1.55 |
|
2 |
0.0366 |
0.002273 |
0.0370 |
0.002159 |
5.02 |
|
2 |
0.0667 |
-0.004473 |
0.0667 |
-0.004479 |
0.13 |
|
2 |
0.0942 |
0.004097 |
0.0942 |
0.004112 |
0.37 |
Amplitude values of the deflections η in the cross-sections of the beam
with the attached masses
at the frequency of the harmonic exciting force ω7 = 1.5·p2
|
Nodal mass |
Theory |
SCAD |
|||
|---|---|---|---|---|---|
|
Time, s |
Deflection, m |
Time, s |
Deflection, m |
Deviations, % |
|
|
1 |
0.0157 |
0.001346 |
0.0155 |
0.001289 |
4.23 |
|
1 |
0.0356 |
-0.001671 |
0.0355 |
-0.001709 |
2.27 |
|
1 |
0.0552 |
0.001788 |
0.0555 |
0.001733 |
3.08 |
|
2 |
0.0308 |
0.001072 |
0.0303 |
0.001006 |
6.16 |
|
2 |
0.0563 |
-0.001420 |
0.0562 |
-0.001459 |
2.75 |
Notes: In the analytical solution the natural frequencies of oscillations p of the simply supported beam are determined according to the following formulas:
\[ p_{1} =\sqrt {\frac{48\cdot E\cdot I}{m\cdot l^{3}}} ; \quad p_{2} =\sqrt {\frac{384\cdot E\cdot I}{m\cdot l^{3}}} . \]
In the analytical solution the deflections η in the cross-sections of the beam with the attached masses with time of the simply supported beam are determined according to the following formulas:
\[ \eta_{1} \left( t \right)=\frac{P_{0} \cdot l^{3}}{768\cdot E\cdot I}\cdot \left[ {\left( {\frac{8}{1-\frac{\omega^{2}}{p_{1} ^{2}}}+\frac{1}{1-\frac{\omega^{2}}{p_{2}^{2}}}} \right)\cdot \cos \left( {\omega \cdot t} \right)-\left( {\frac{8}{1-\frac{\omega^{2}}{p_{1} ^{2}}}\cdot \cos \left( {p_{1} \cdot t} \right)+\frac{1}{1-\frac{\omega ^{2}}{p_{2}^{2}}}\cdot \cos \left( {p_{2} \cdot t} \right)} \right)} \right]; \] \[ \eta_{2} \left( t \right)=\frac{P_{0} \cdot l^{3}}{768\cdot E\cdot I}\cdot \left[ {\left( {\frac{8}{1-\frac{\omega^{2}}{p_{1} ^{2}}}-\frac{1}{1-\frac{\omega^{2}}{p_{2}^{2}}}} \right)\cdot \cos \left( {\omega \cdot t} \right)-\left( {\frac{8}{1-\frac{\omega^{2}}{p_{1} ^{2}}}\cdot \cos \left( {p_{1} \cdot t} \right)-\frac{1}{1-\frac{\omega ^{2}}{p_{2}^{2}}}\cdot \cos \left( {p_{2} \cdot t} \right)} \right)} \right]. \]
