Simply Supported Beam with a Distributed Mass Subjected to a Kinematic Excitation of Supports (Seismic Action)
Objective: Determination of the stress-strain state of a simply supported beam with a distributed mass subjected to a kinematic excitation of supports.
Initial data file: DIN_B_SL.spr, DIN_B_SL.spc
Problem formulation: The simply supported beam of constant cross-section with the uniformly distributed mass μ is subjected to the kinematic excitation of supports according to the specified accelerogram:
\[ \ddot{{z}}(t)=\ddot{{z}}_{s0} \cdot \left( {1-\frac{t}{t_{d} }} \right). \]
Determine the natural oscillation mode and the fundamental natural frequency f of the simply supported beam, as well as the maximum amplitude values of the deflection z and the bending moment M in the cross-section in the middle of the beam span with time t.
References: John M. Biggs, Introduction to Structural Dynamics, McGraw-Hill Book Companies, New York, 1964, p.262.
Initial data:
| E = 3.0·107 psi = 2.1092·107tf/m2 | - elastic modulus; |
| I = 333.333 in4 = 138.7448·10-6 m4 | - cross-sectional moment of inertia of the beam. |
| h = 14 in = 0.3556 m | - height of the cross-section of the beam; |
| L = 240 in = 6.0960 m | - beam span length; |
| μ = 0.2 lb·sec2/in2 = 0.1406 tf·s2/m2 | - value of the uniformly distributed mass of the beam; |
| \(\ddot{{z}}_{s0}=\) ±386.2200 in/sec2 = ±9.81 m/s2 | - amplitude values of the acceleration of the supports according to the accelerogram; |
| td = 0.10 sec = 0.10 s | - half-interval of the kinematic excitation of supports. |
| g = 386.2200 in/sec2 =9.81 m/s2 | - gravitational acceleration. |
Finite element model: Design model – grade beam / plate, 32 bar elements of type 3. Boundary conditions of the simply supported ends of the beam are provided by imposing constraints in the direction of the degree of freedom Z. The dimensional stability of the design model is provided by imposing a constraint in the node of the cross-section along the symmetry axis of the beam in the direction of the degree of freedom UX. The distributed mass is specified by transforming the static load from the self-weight of the beam μ·g.
The kinematic excitation of supports is described by the graph of the acceleration variation with time (accelerogram) and is given in the form of the action along the Z axis of the global coordinate system (direction cosines to the X, Y, Z axes: 0.00, 0.00, 1.00) with the scale factor to the values of the accelerogram equal to 1.00. The height of the beam structure in the model is directed along the Z axis of the global coordinate system. The dissipation factor (energy absorption factor) is taken with the minimum value of ξ = 0.000001. The intervals between the time points of the graph of the acceleration variation with time are equal to Δt = 0.01 s. When plotting the graph the acceleration is taken with the values \( \ddot{z}(t) = \ddot{z_{s0}}(t)\left(1-n\frac{\Delta t}{t_d}\right) \) at the time points n·Δt. The conversion factor for the added static loading is equal to k = 1.000 (mass generation). Number of nodes in the design model – 33. The modal integration method is used in the calculation. The determination of the natural oscillation modes and natural frequencies is performed by the method of subspace iteration. The matrix of concentrated masses is used in the calculation.
Results in SCAD
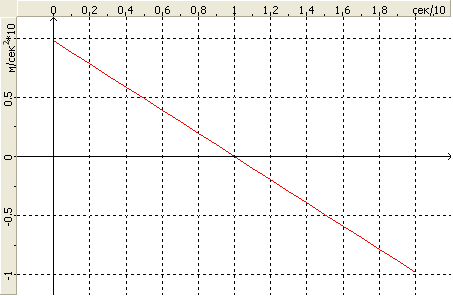
Design model and the specified accelerogram
1-st natural oscillation mode
Amplitude value of the deflection z in the cross-section in the middle of the beam span and the deformed model at the respective time point (m)
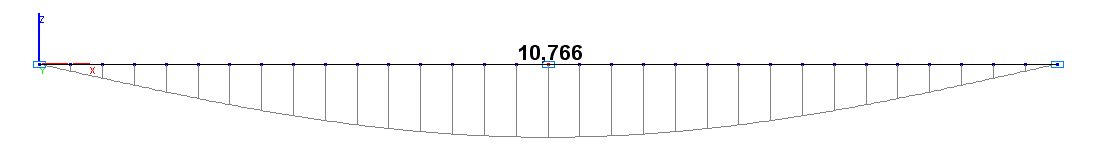
Amplitude value of the bending moment M in the cross-section in the middle of the beam span (tm·m)
Comparison of solutions:
Fundamental natural frequency f, Hz
|
Oscillation mode |
Theory |
SCAD |
Deviations, % |
|---|---|---|---|
|
1 |
6.098 |
6.101 |
0.05 |
Maximum amplitude value of the deflection z in the cross-section in the middle of the beam span, m
|
Theory |
SCAD |
||
|---|---|---|---|
|
Time, s |
Deflection, m |
Deflection, m |
Deviations, % |
|
0.0163982 |
-0.013951 |
-0.013841 |
0.80 |
Maximum amplitude value of the bending moment M in the cross-section in the middle of the beam span, tf·m
|
Theory |
SCAD |
||
|---|---|---|---|
|
Time, s |
Bending moment, tf·m |
Bending moment, tf·m |
Deviations, % |
|
0.0163982 |
10.843 |
10.766 |
0.73 |
Notes: In the analytical solution the fundamental natural frequency f of the simply supported beam is determined according to the following formula:
\[ f=\frac{\pi }{2\cdot L^{2}}\cdot \sqrt {\frac{E\cdot I}{\mu }} \quad . \]
In the analytical solution the deflection z and the bending moment M in the cross-section in the middle of the beam span with time are determined according to the following formula:
\[z\left( t \right)=\frac{4\cdot \ddot{{z}}_{s0} \cdot L^{4}\cdot \mu }{\pi ^{5}\cdot E\cdot I}\cdot \left[ {1-\cos \left( {\frac{\pi^{2}}{L^{2}}\cdot \sqrt {\frac{E\cdot I}{\mu }} \cdot t} \right)+\frac{L^{2}}{\pi^{2}\cdot t_{d} }\cdot \sqrt {\frac{\mu }{E\cdot I}} \cdot \sin \left( {\frac{\pi ^{2}}{L^{2}}\cdot \sqrt {\frac{E\cdot I}{\mu }} \cdot t} \right)-\frac{1}{t_{d} }\cdot t} \right]; \quad at \quad t\le 2\cdot t_{d}; \] \[M\left( t \right)=\frac{4\cdot \ddot{{z}}_{s0} \cdot L^{2}\cdot \mu }{\pi ^{3}}\cdot \left[ {1-\cos \left( {\frac{\pi^{2}}{L^{2}}\cdot \sqrt {\frac{E\cdot I}{\mu }} \cdot t} \right)+\frac{L^{2}}{\pi^{2}\cdot t_{d} }\cdot \sqrt {\frac{\mu }{E\cdot I}} \cdot \sin \left( {\frac{\pi ^{2}}{L^{2}}\cdot \sqrt {\frac{E\cdot I}{\mu }} \cdot t} \right)-\frac{1}{t_{d} }\cdot t} \right]; \quad at \quad t\le 2\cdot t_{d} .\]