Natural Oscillations of a Clamped Circular Plate
Objective: Modal analysis of a clamped circular plate.
Initial data file: 5.6.spr
Problem formulation: Determine the natural oscillation modes and frequencies ω of the clamped circular plate with the density of the material ρ.
References: Chelomei V.N., Vibrations in Technology, Handbook in six volumes: Bolotin V.V., Volume 1, Vibrations of Linear Systems, Moscow, Mechanical engineering, 1978, p. 207.
Initial data:
| E = 2.06·108 kPa | - elastic modulus; |
| ν = 0.3 | - Poisson’s ratio; |
| ρ = 7.85 t/m3 | - density of the material; |
| h = 0.01 m | - thickness of the plate; |
| R = 0.5 m | - outer radius of the plate. |
Finite element model: Design model – grade beam / plate, 1080 four-node plate elements of type 20 and 72 three-node plate elements of type 15. The spacing of the finite element mesh in the radial direction is 0.03125 m and in the tangential direction is 5.0º. Boundary conditions are provided by imposing constraints in the directions of the degrees of freedom Z, UX, UY along the outer contour of the plate. The distributed mass is specified by transforming the static load from the self-weight of the plate ow = γ∙h, where γ = ρ∙g = 77.01 kN/m3. Number of nodes in the design model – 1153. The determination of the natural oscillation modes and natural frequencies is performed by the method of subspace iteration. The matrix of concentrated masses is used in the calculation.
Results in SCAD
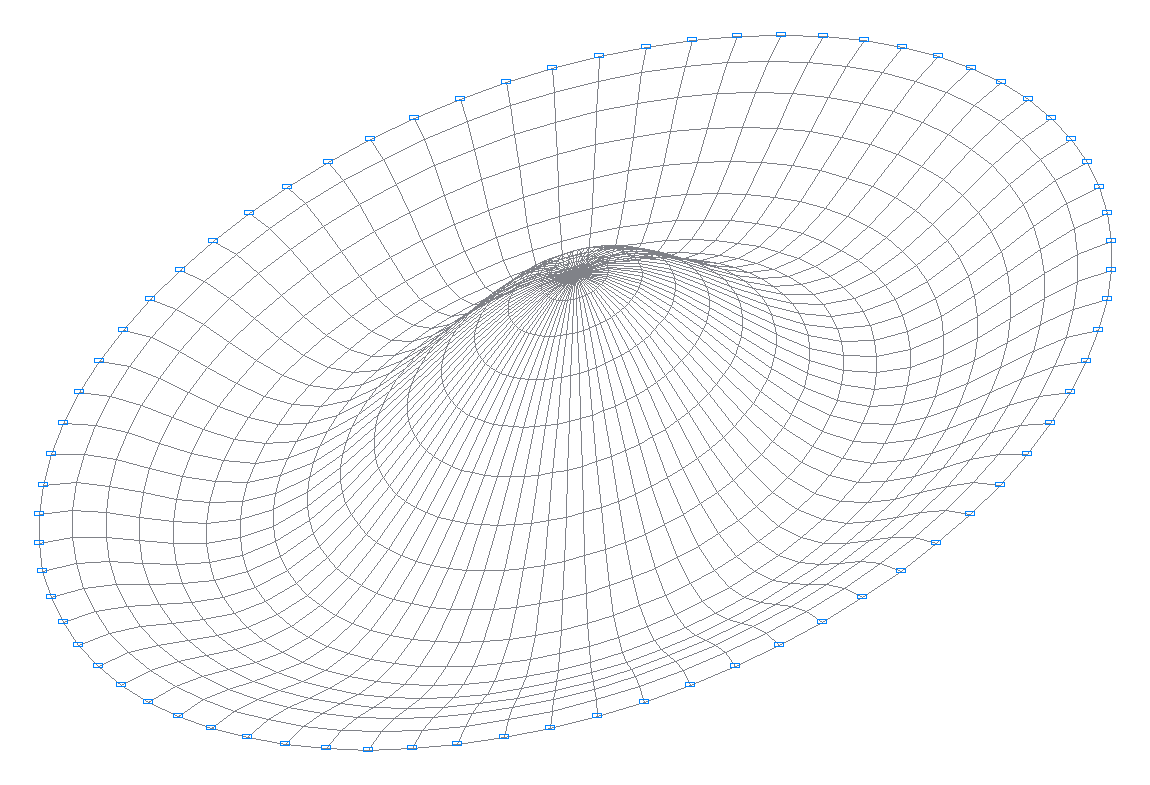
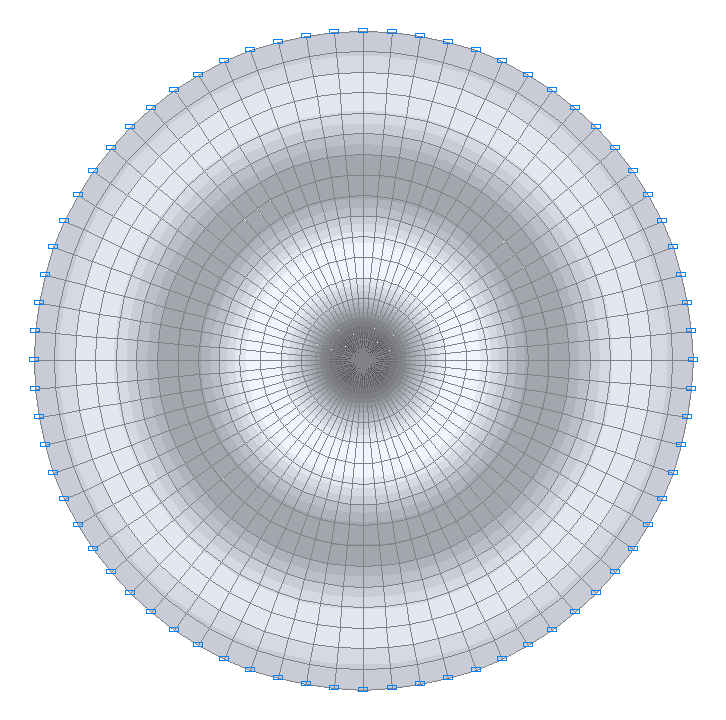
Design model
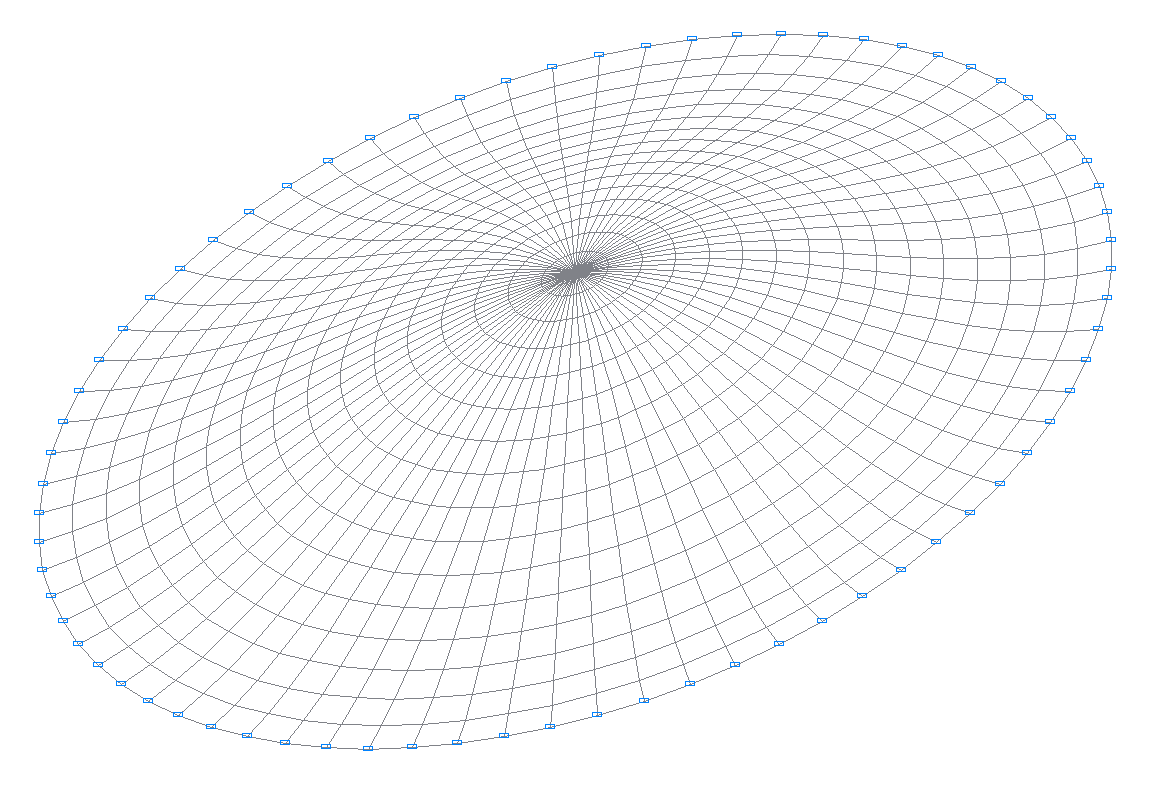
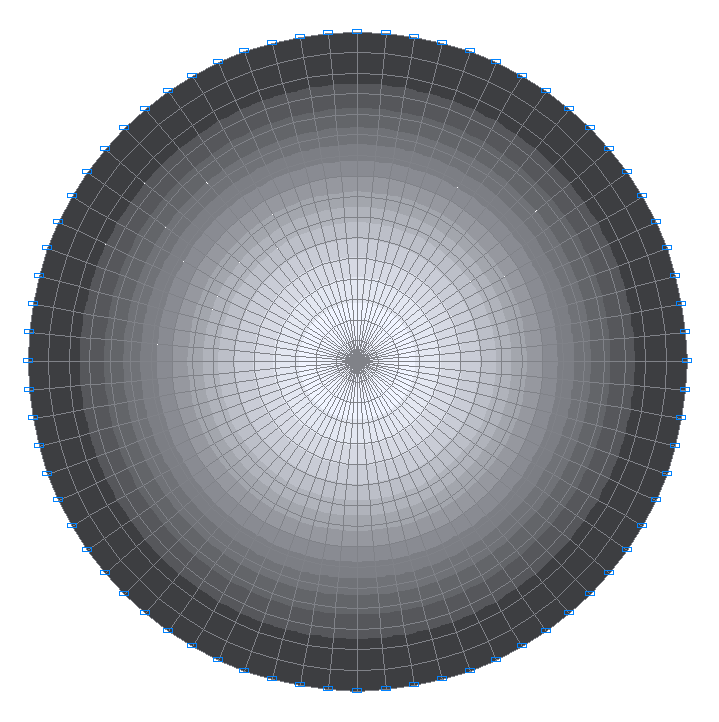

1-st natural oscillation mode
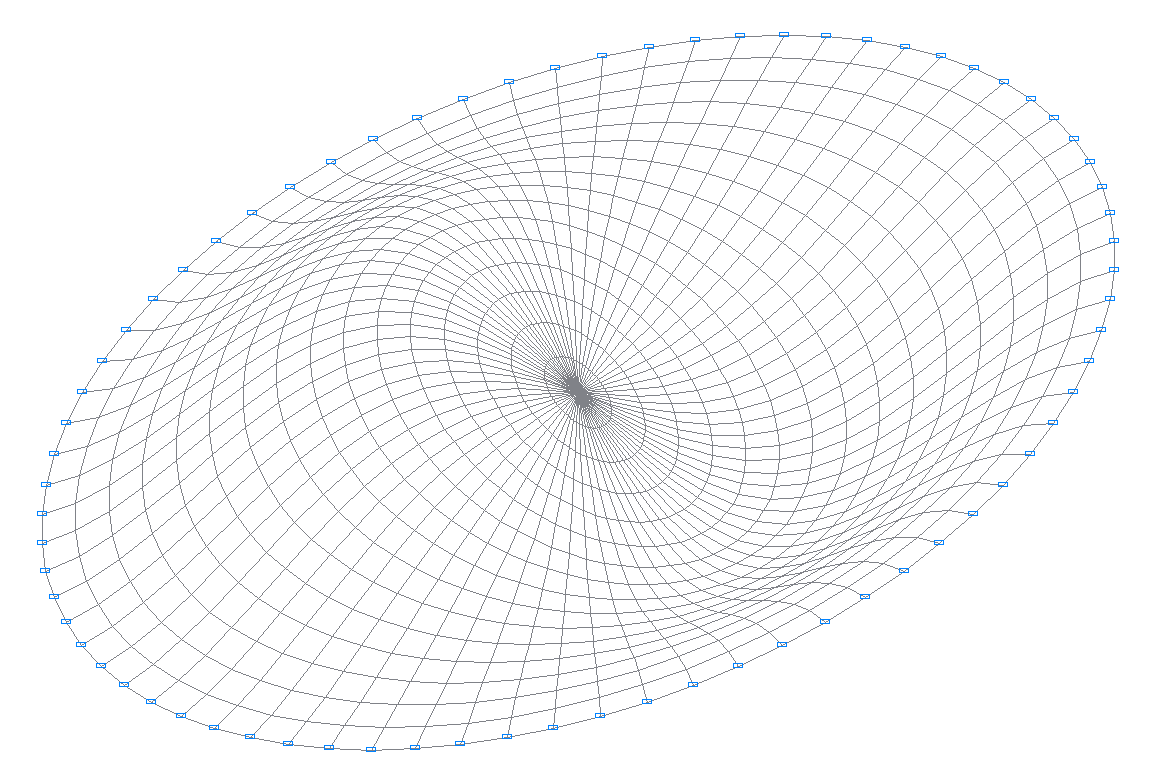
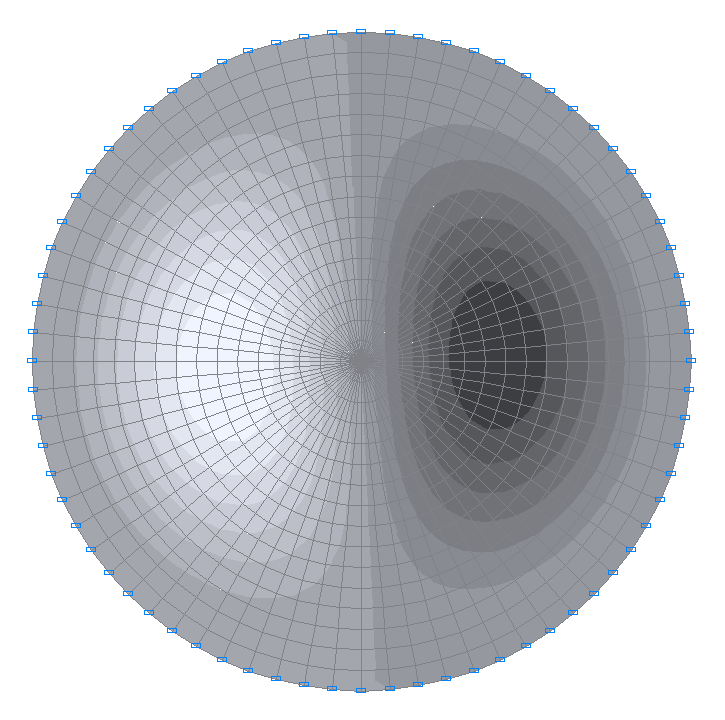

2-nd natural oscillation mode
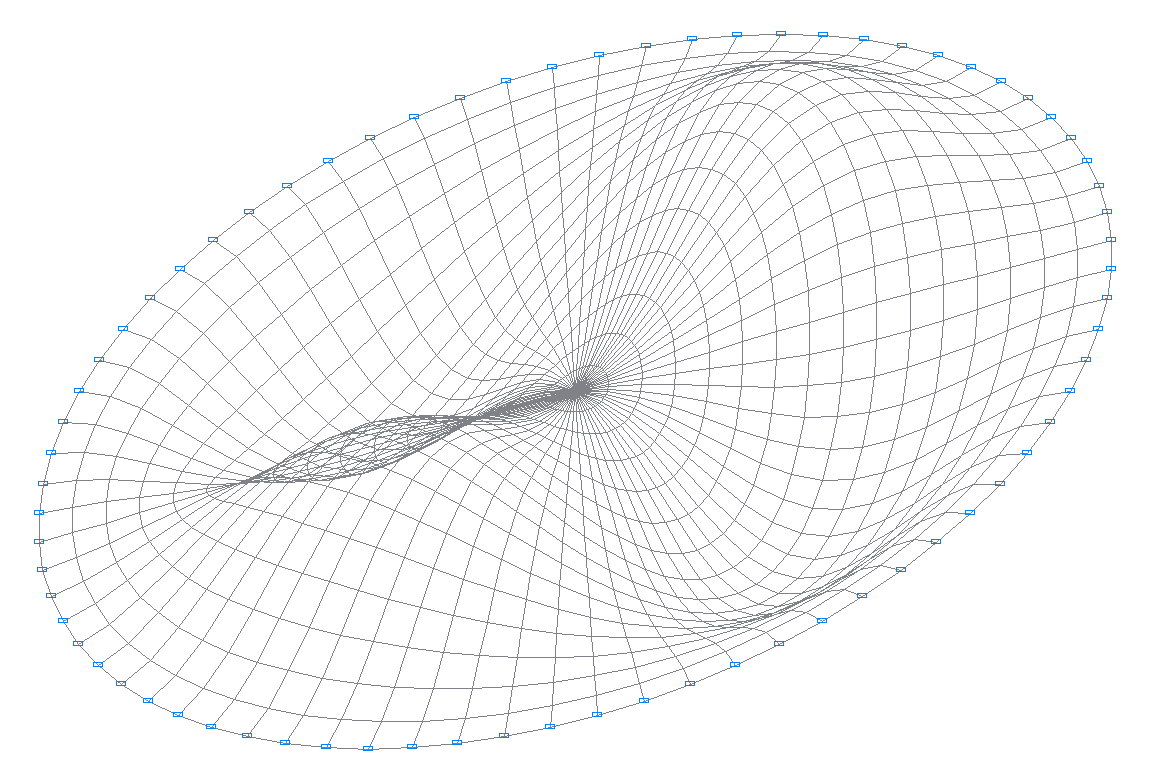
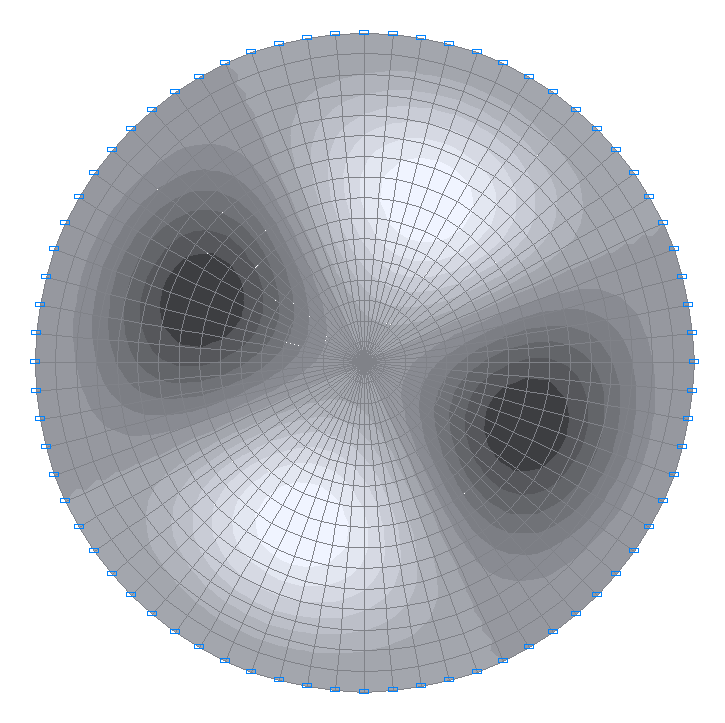

4-th natural oscillation mode
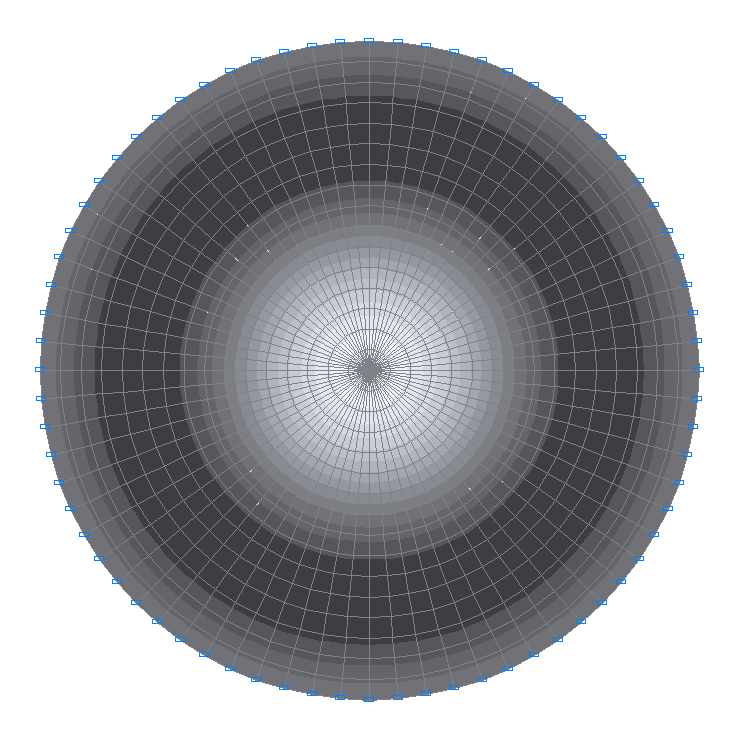

6-th natural oscillation mode

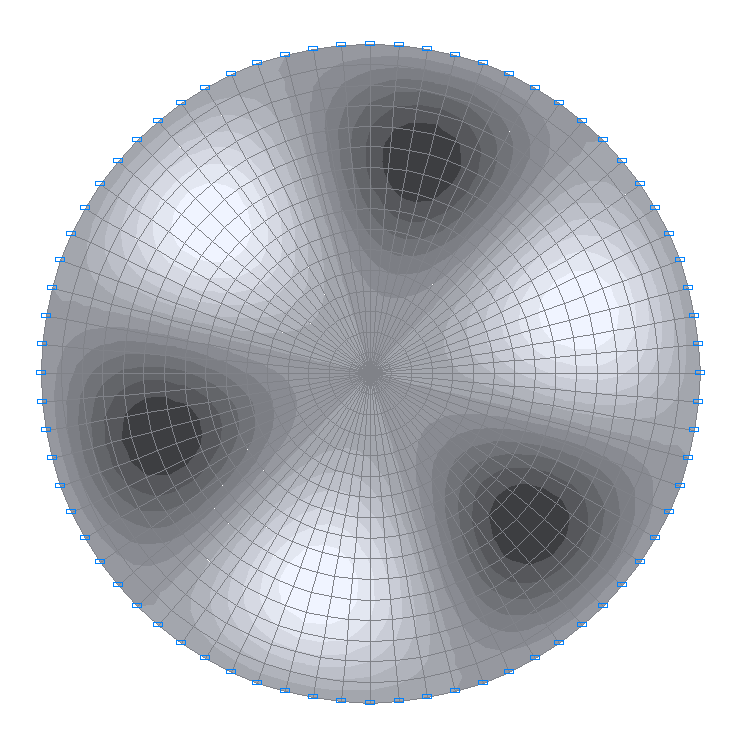

7-th natural oscillation mode

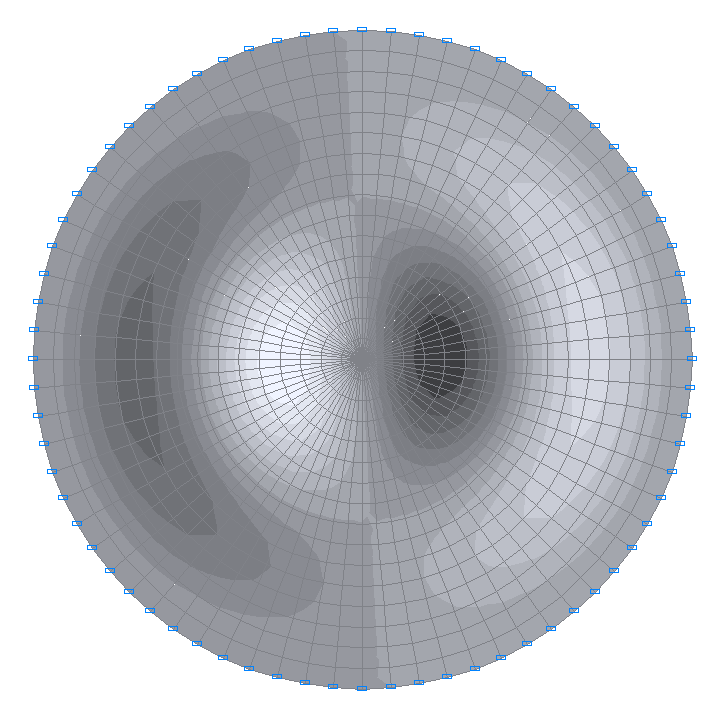

9-th natural oscillation mode
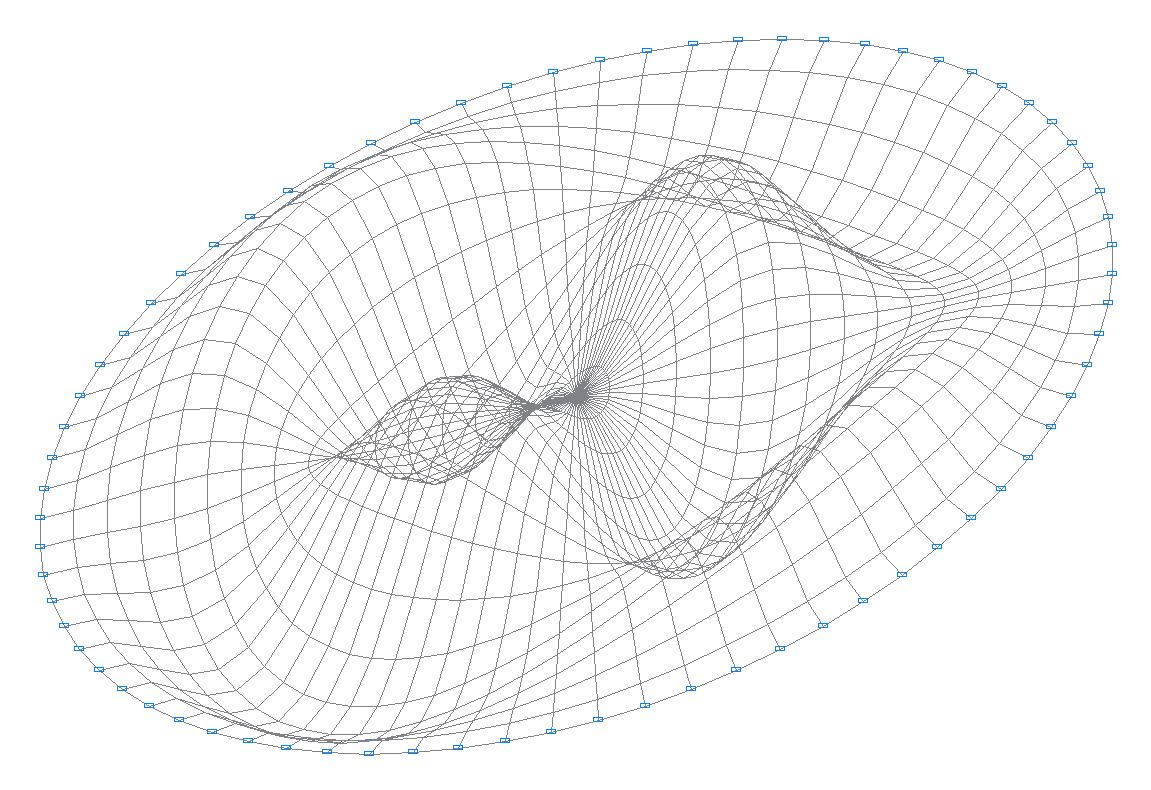
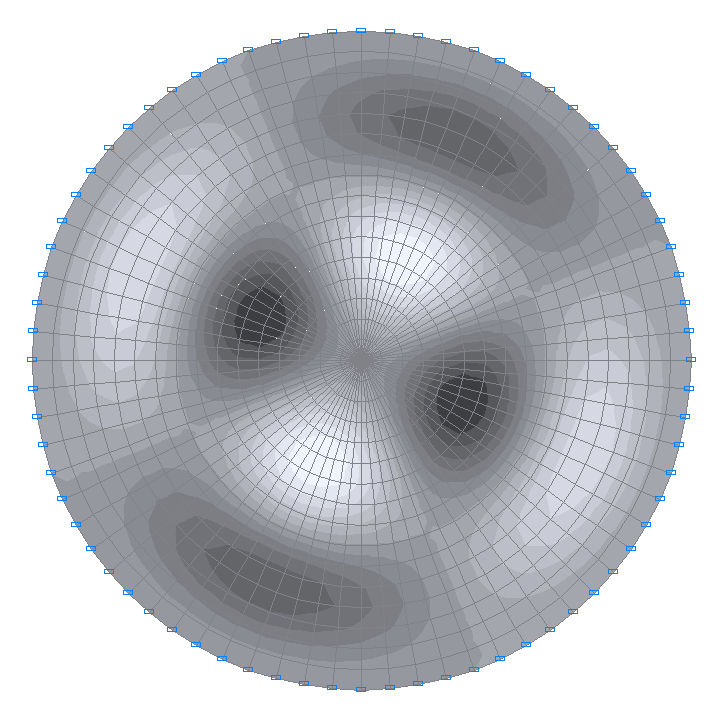

13-th natural oscillation mode



15-th natural oscillation mode
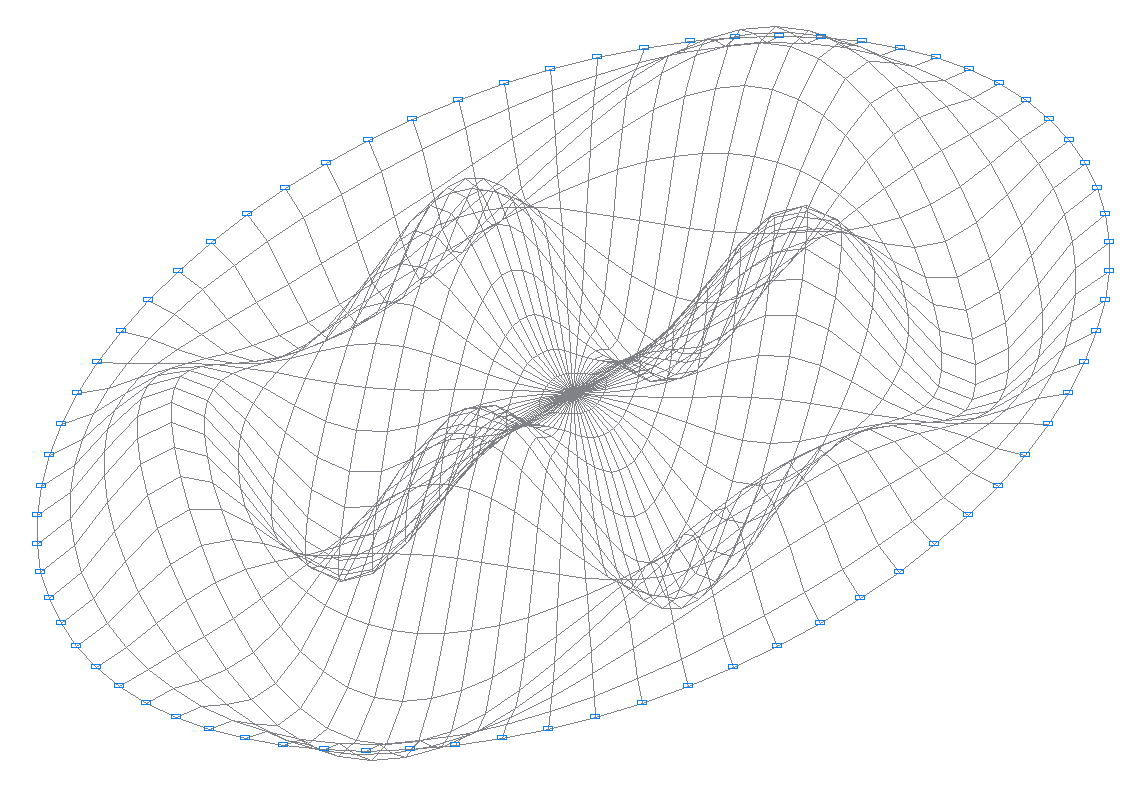
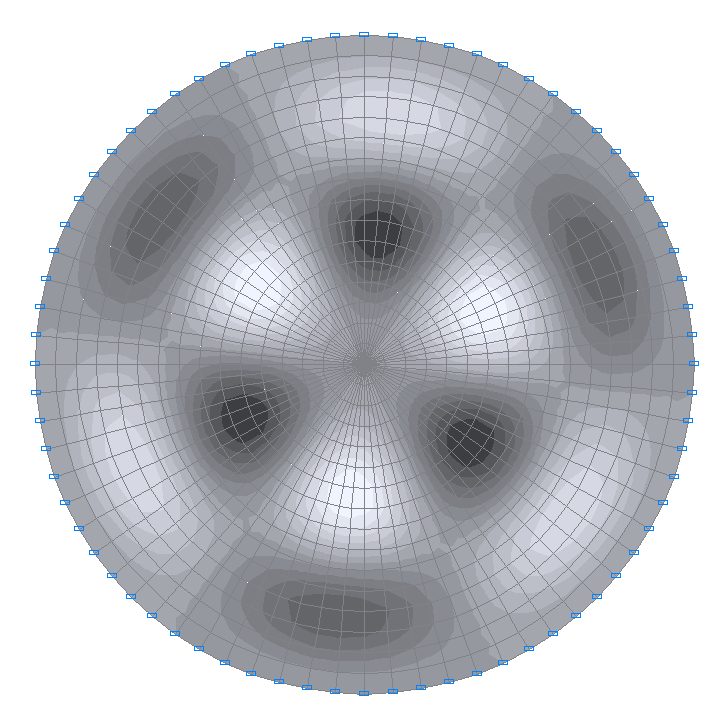

18-th natural oscillation mode
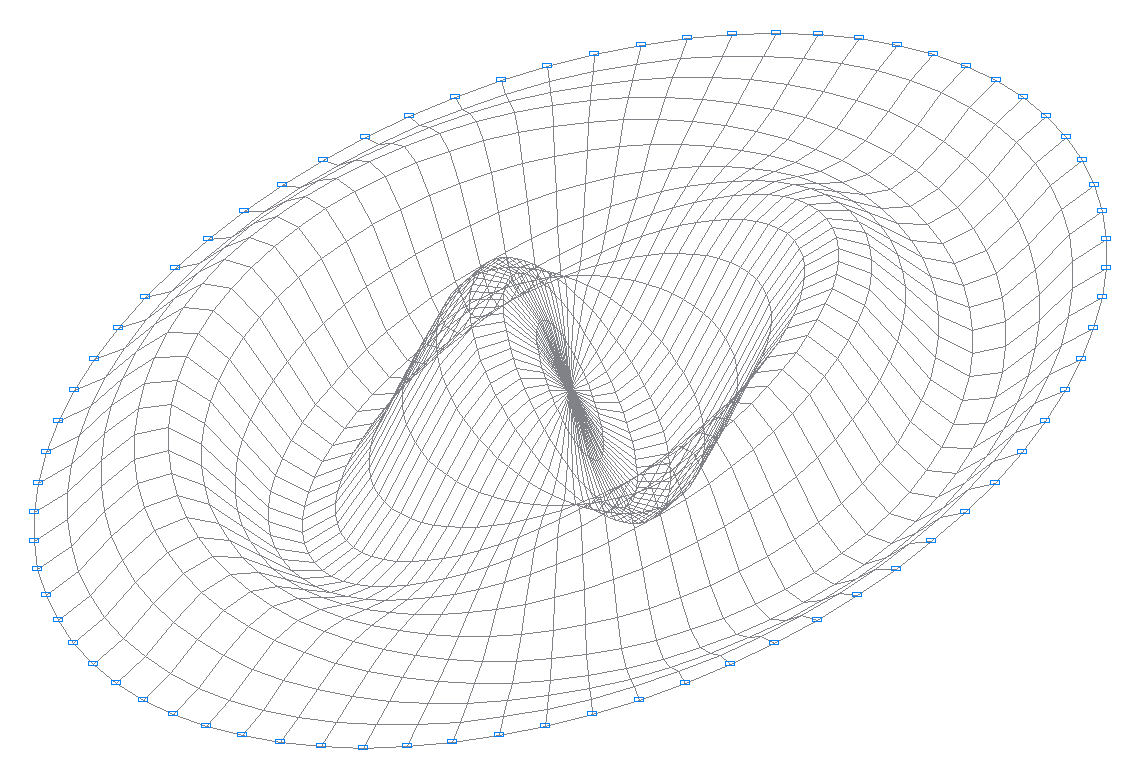


22-nd natural oscillation mode
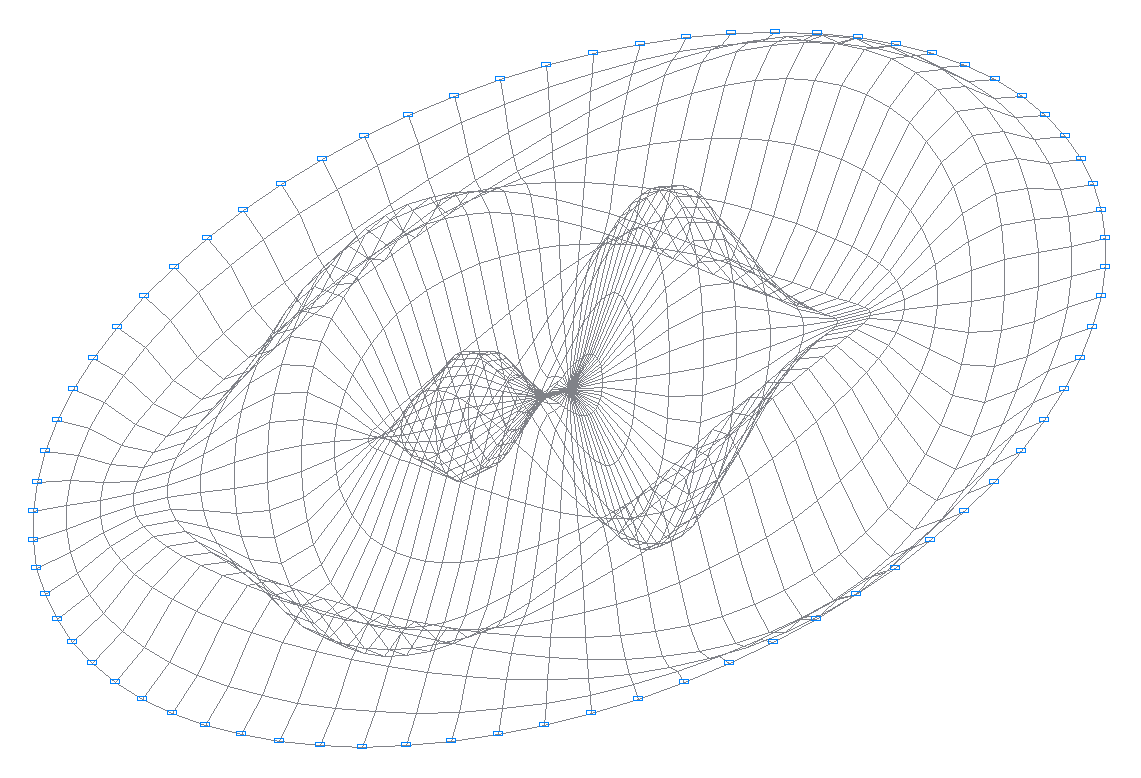
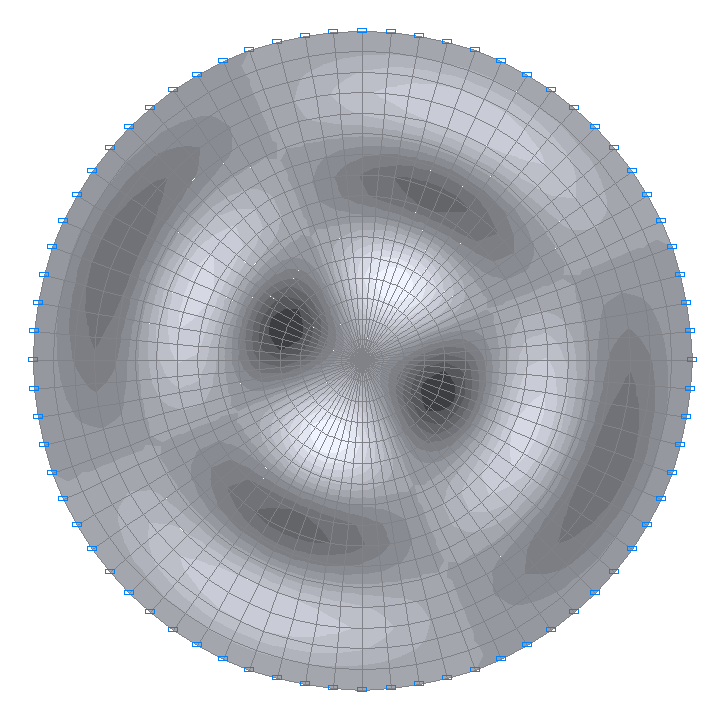

28-th natural oscillation mode
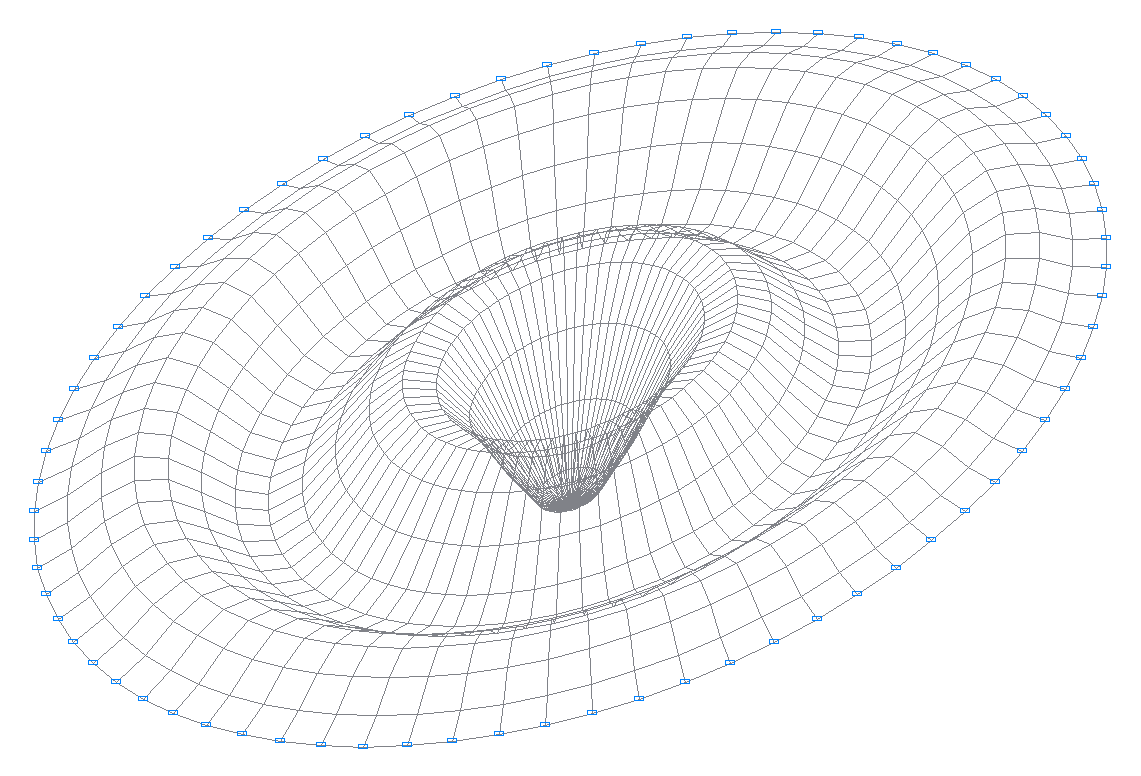

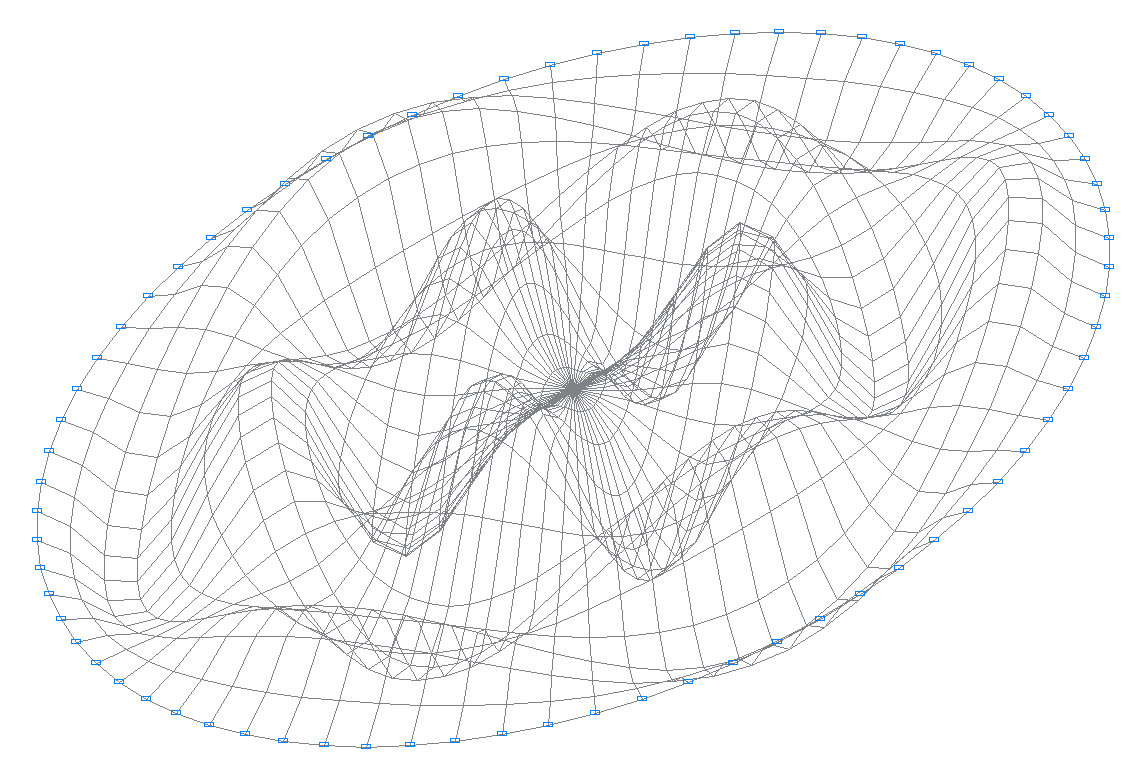
30-th natural oscillation mode


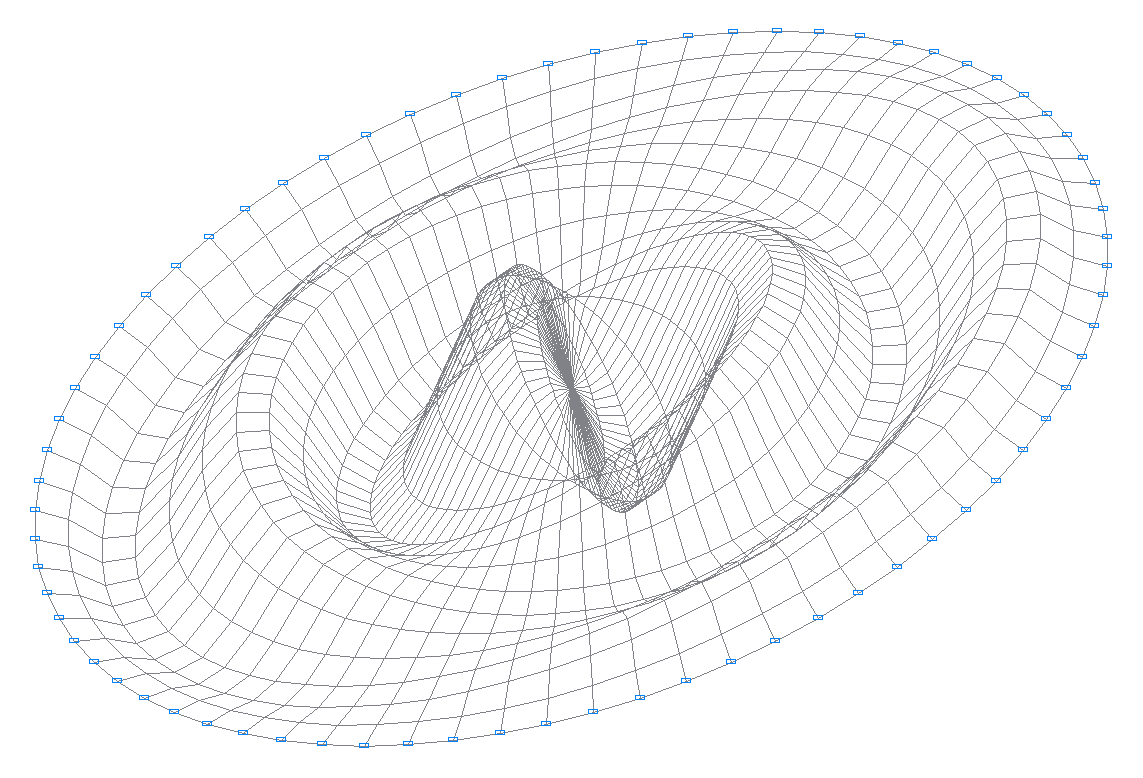
35-th natural oscillation mode
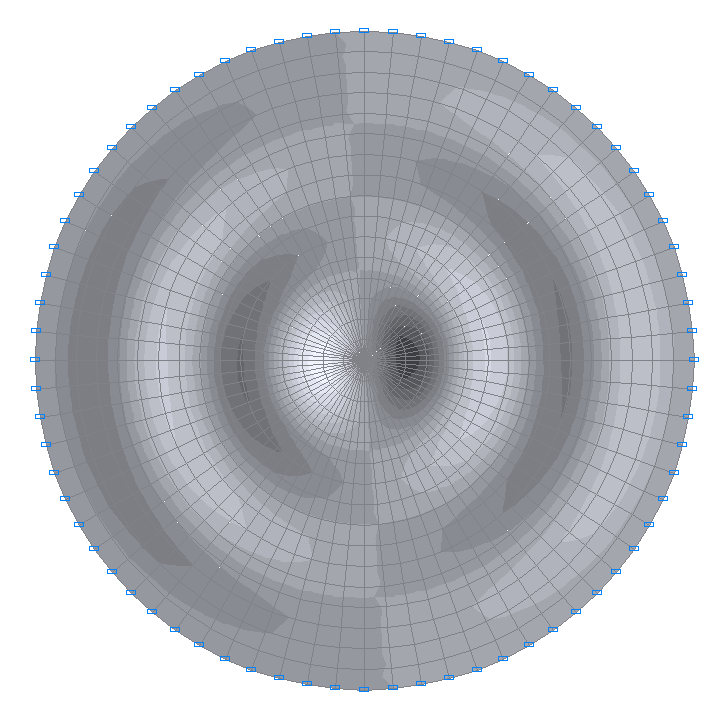

37-th natural oscillation mode
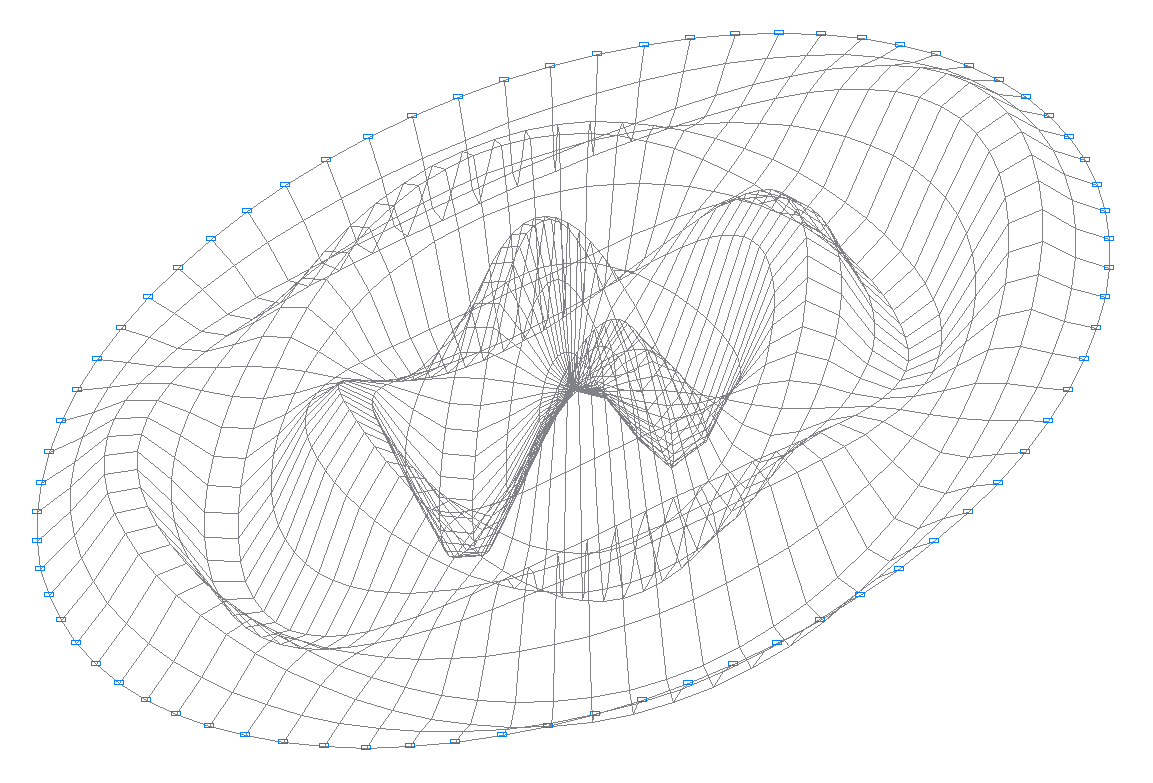
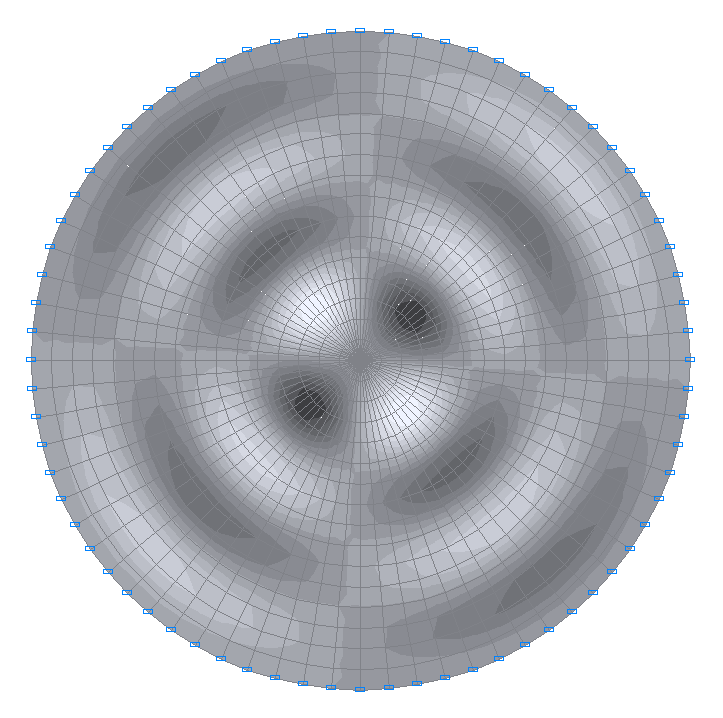

50-th (47-th theoretical) natural oscillation mode
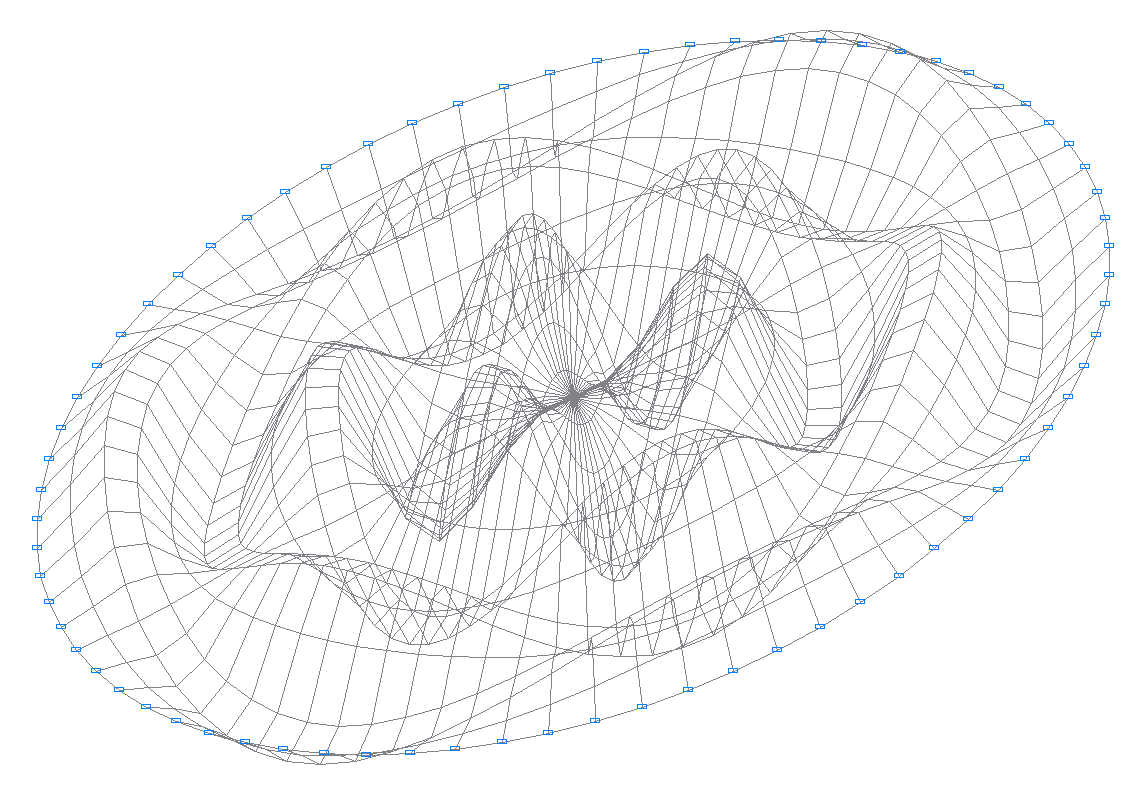
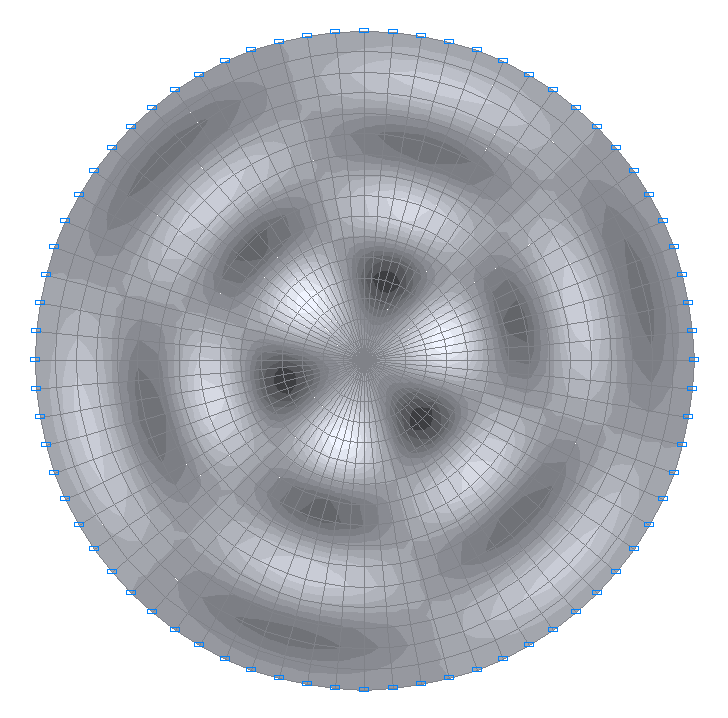

58-th natural oscillation mode
Comparison of solutions:
Natural frequencies ω, rad / s
|
Oscillation mode |
Number of nodal circles m and diameters n |
Theory |
SCAD |
Deviations, % |
|---|---|---|---|---|
|
1 |
0, 0 |
633.5 |
633.8 |
0.05 |
|
2, 3 |
0, 1 |
1318.3 |
1321.7 |
0.26 |
|
4, 5 |
0, 2 |
2162.7 |
2170.6 |
0.37 |
|
6 |
1, 0 |
2466.1 |
2463.8 |
0.09 |
|
7, 8 |
0, 3 |
3164.3 |
3178.9 |
0.46 |
|
9, 10 |
1, 1 |
3771.9 |
3784.3 |
0.33 |
|
11, 12 |
0, 4 |
4319.8 |
4340.1 |
0.47 |
|
13, 14 |
1, 2 |
5244.8 |
5280.1 |
0.67 |
|
15 |
2, 0 |
5525.2 |
5511.5 |
0.25 |
|
16, 17 |
0, 5 |
5626.5 |
5655.5 |
0.52 |
|
18, 19 |
1, 3 |
6884.2 |
6931.9 |
0.69 |
|
20, 21 |
0, 6 |
7082.1 |
7123.6 |
0.59 |
|
22, 23 |
2, 1 |
7445.9 |
7477.9 |
0.43 |
|
24, 25 |
0, 7 |
8684.6 |
8742.7 |
0.67 |
|
26, 27 |
1, 4 |
8687.8 |
8748.6 |
0.70 |
|
28, 29 |
2, 2 |
9537.8 |
9652.0 |
1.20 |
|
30 |
3, 0 |
9808.7 |
9769.4 |
0.40 |
|
31, 32 |
0, 8 |
10432.5 |
10511.5 |
0.76 |
|
33, 34 |
1, 5 |
10653.2 |
10730.3 |
0.72 |
|
35, 36 |
2, 3 |
11800.3 |
11917.6 |
0.99 |
|
37, 38 |
0, 9 |
12324.5 |
12429.0 |
0.85 |
|
39, 40 |
3, 1 |
12342.9 |
12408.9 |
0.53 |
|
41, 42 |
1, 6 |
12778.0 |
12880.4 |
0.80 |
|
43, 44 |
2, 4 |
14232.0 |
14378.8 |
1.03 |
|
45, 46 |
0, 10 |
14359.4 |
14494.1 |
0.94 |
|
47, 48 |
3, 2 |
15050.6 |
15335.5 |
1.89 |
|
49, 50 |
1, 7 |
15060.4 |
15196.7 |
0.91 |
|
51 |
4, 0 |
15316.4 |
15229.9 |
0.56 |
|
52, 53 |
0, 11 |
16536.2 |
16705.2 |
1.02 |
|
54, 55 |
2, 5 |
16830.7 |
17001.7 |
1.02 |
|
56, 57 |
1, 8 |
17498.5 |
17677.4 |
1.02 |
|
58, 59 |
3, 3 |
17931.5 |
18171.2 |
1.34 |
|
60, 61 |
4, 1 |
18463.5 |
18580.3 |
0.63 |
Notes: In the analytical solution the natural frequencies ω of the clamped circular plate with the density of the material ρ can be determined according to the following equation obtained on the basis of the factorization method:
\( \frac{J_{n+1} \left( {\beta \cdot R} \right)}{J_{n} \left( {\beta \cdot R} \right)}+\frac{I_{n+1} \left( {\beta \cdot R} \right)}{I_{n} \left( {\beta \cdot R} \right)}=0,\quad where: \)
\( \beta =\left( {\frac{\rho \cdot h\cdot \omega^{2}}{D}} \right)^{\frac{1}{4}}, \quad D=\frac{E\cdot h^{3}}{12\cdot \left( {1-\nu^{2}} \right)}, \) n=0,1,2,3, ... - number of nodal diameters,
\( J_{n} \left( {\beta \cdot R} \right), \quad J_{n+1} \left( {\beta \cdot R} \right) \) - values of the Bessel function of the first kind of order n,
\( I_{n} \left( {\beta \cdot R} \right), \quad I_{n+1} \left( {\beta \cdot R} \right) \) - values of the modified Bessel function of the first kind of order n.
