Natural Oscillations of a Simply Supported Rectangular Plate
Objective: Modal analysis of a simply supported rectangular plate.
Initial data file: 5.3.spr
Problem formulation: Determine the natural oscillation modes and frequencies ω of the simply supported rectangular plate with the density of the material ρ.
References: I.A. Birger, Ya.G. Panovko, Strength, Stability, Vibrations, Handbook in three volumes, Volume 3, Moscow, Mechanical engineering, 1968, p. 375.
Initial data:
| E = 2.06·108 kPa | - elastic modulus; |
| ν = 0.3 | - Poisson’s ratio; |
| ρ = 7.85 t/m3 | - density of the material; |
| h = 0.01 м | - thickness of the plate; |
| a1 = 1.5 м | - long side of the plate (along the X axis of the global coordinate system); |
| a2 = 1.0 м | - short side of the plate (along the Y axis of the global coordinate system). |
Finite element model: Design model – grade beam / plate, 600 plate elements of type 20. The spacing of the finite element mesh along the sides of the plate (along the X, Y axes of the global coordinate system) is 0.05 m. Boundary conditions are provided by imposing constraints in the direction of the degree of freedom Z for the edges parallel to the X and Y axes of the global coordinate system. The distributed mass is specified by transforming the static load from the self-weight of the plate ow = γ∙h, where γ = ρ∙g = 77.01 kN/m3. Number of nodes in the design model – 651. The determination of the natural oscillation modes and natural frequencies is performed by the method of subspace iteration. The matrix of concentrated masses is used in the calculation.
Results in SCAD
Design model

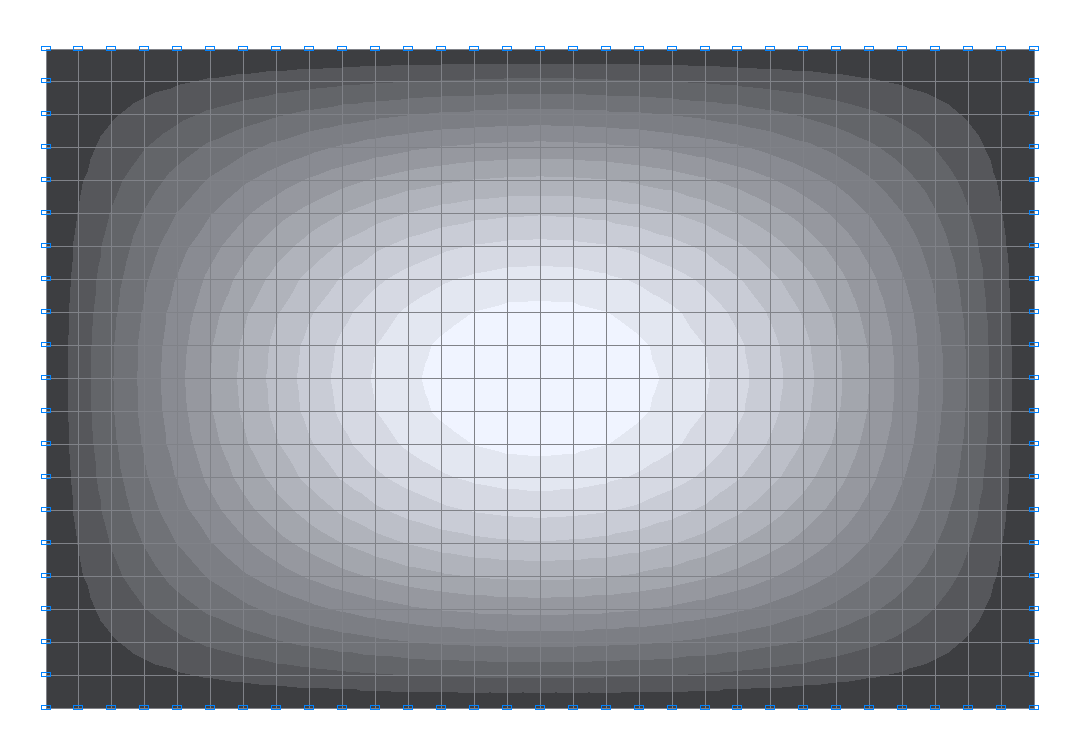

1-st natural oscillation mode



2-nd natural oscillation mode
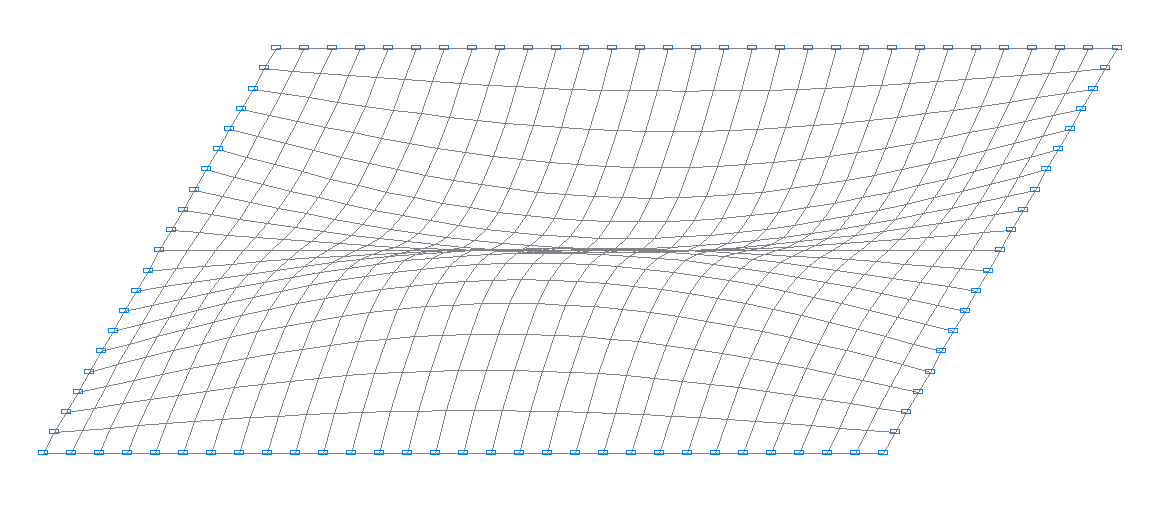
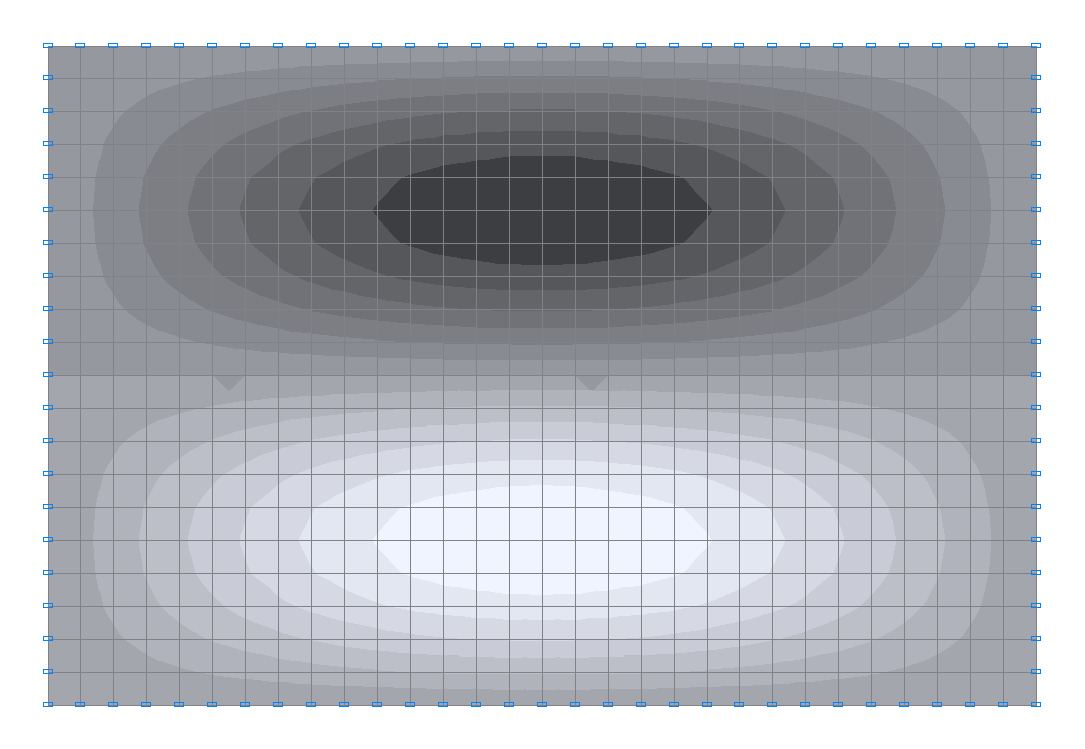
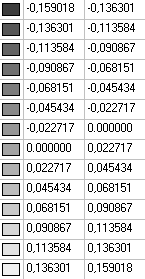
3-rd natural oscillation mode
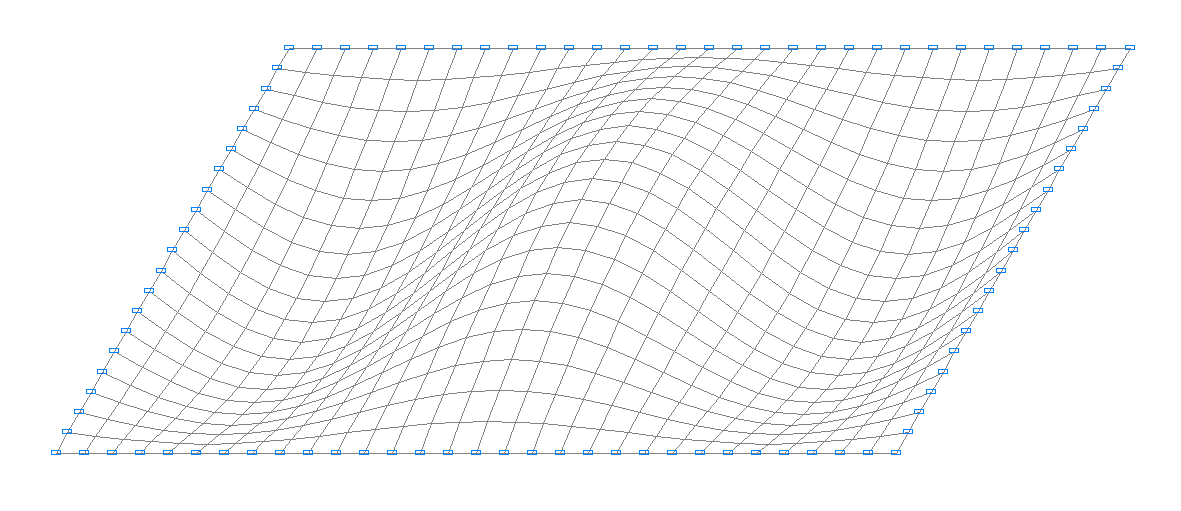
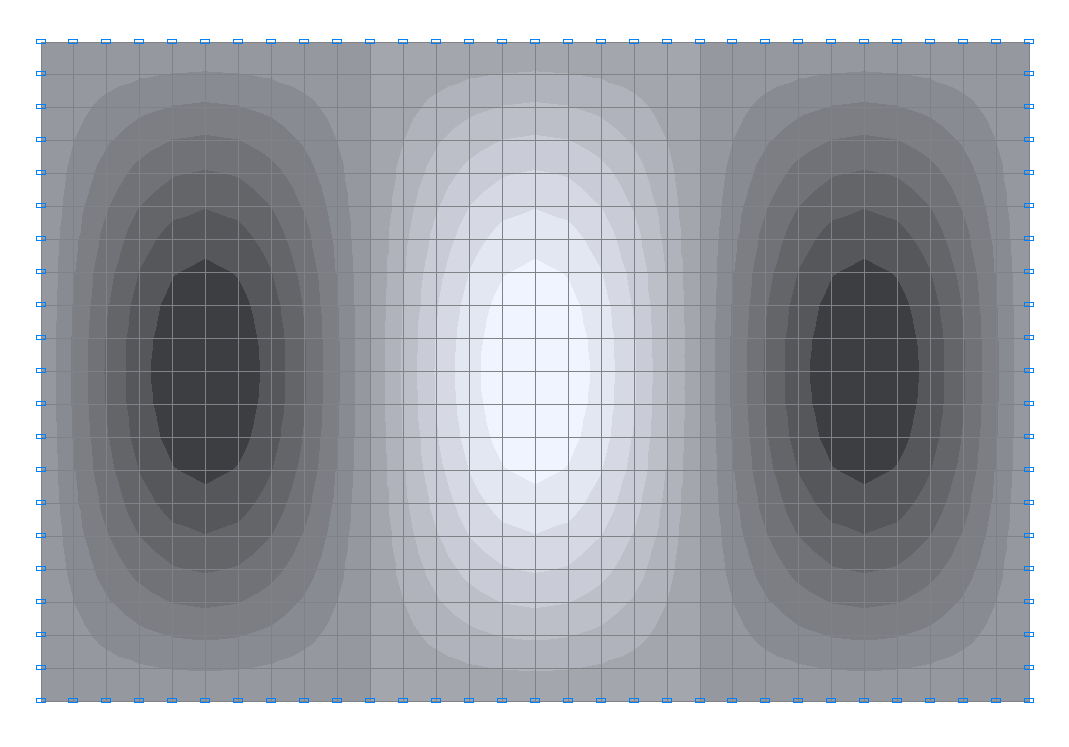

4-th natural oscillation mode
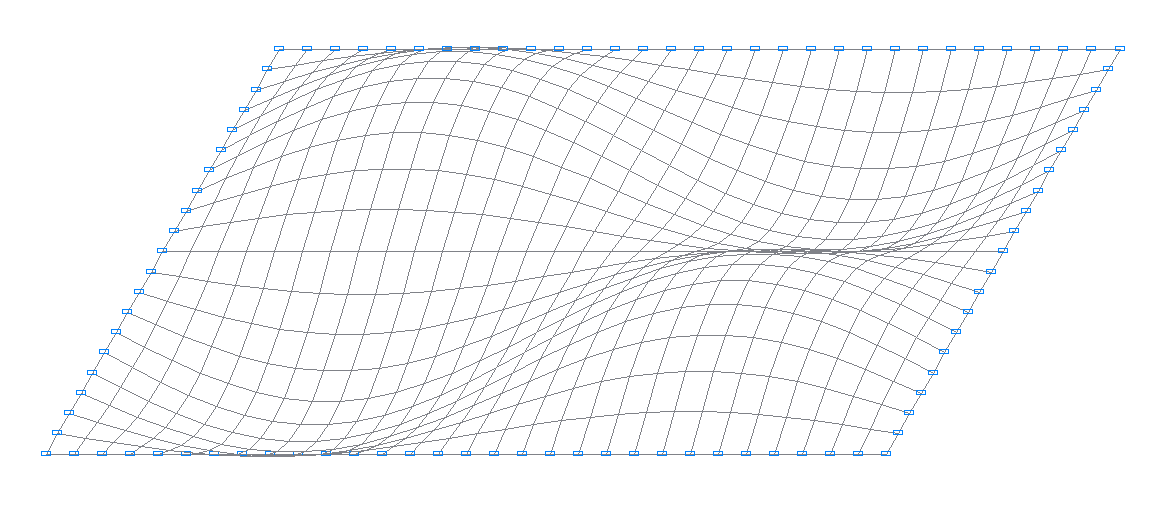
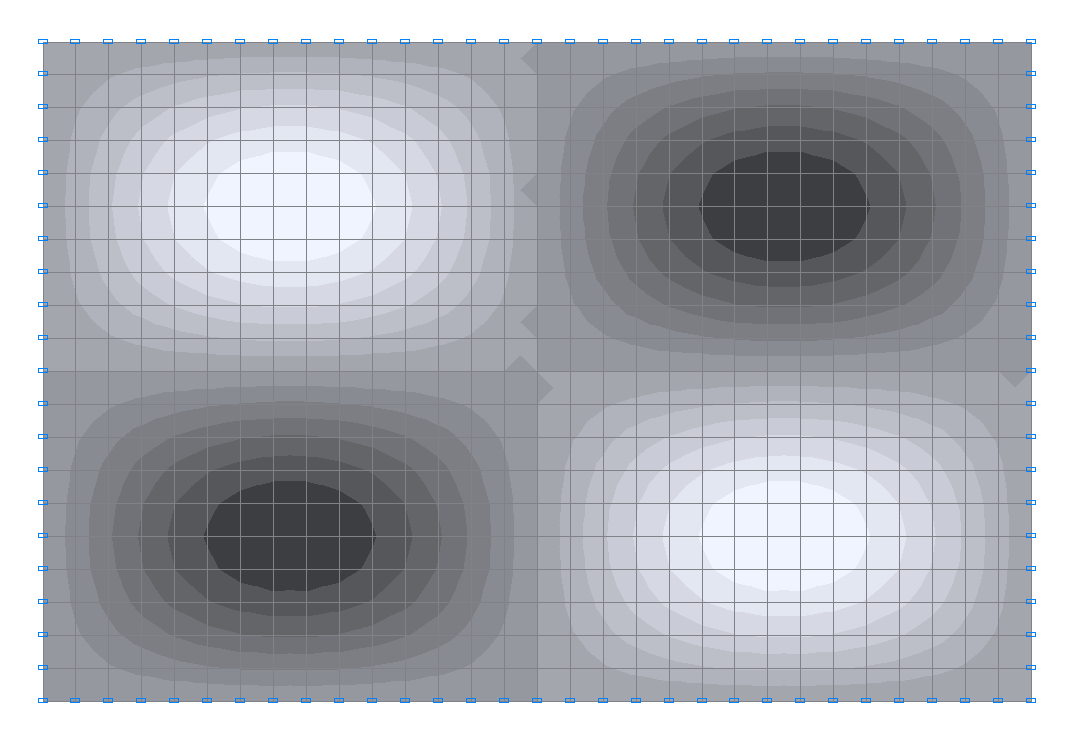

5-th natural oscillation mode
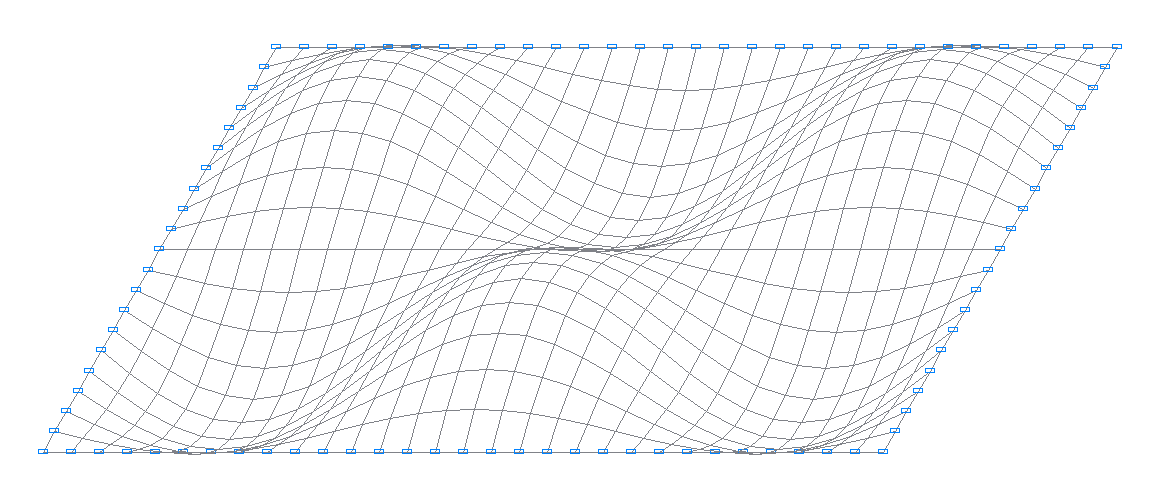
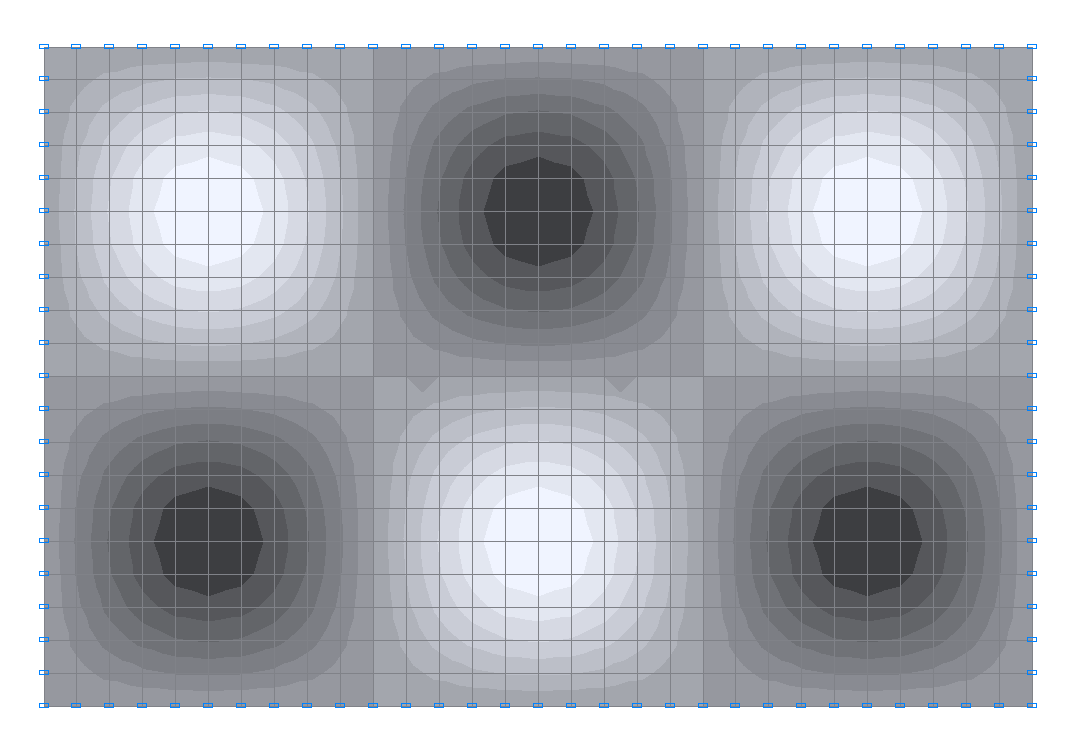

6-th natural oscillation mode
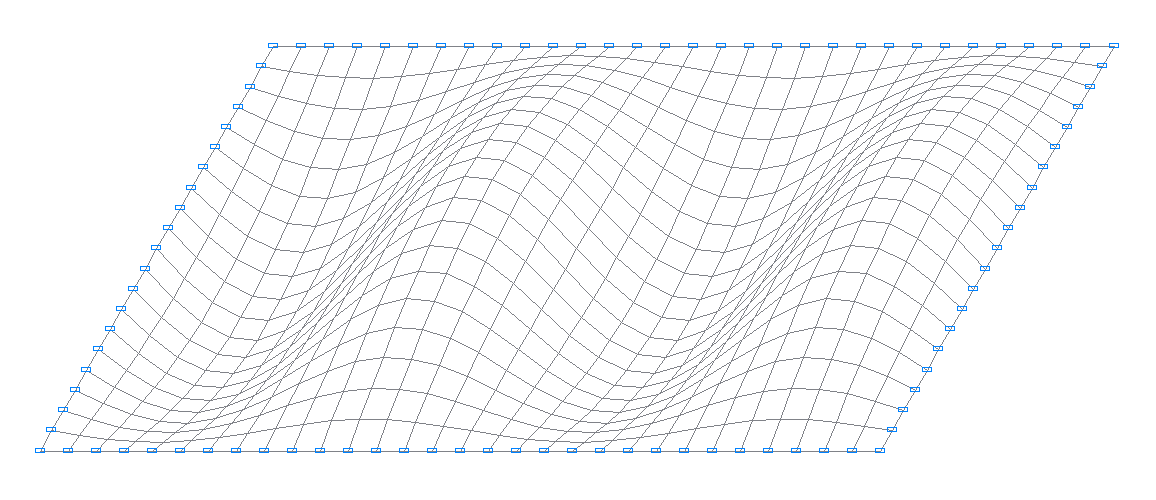
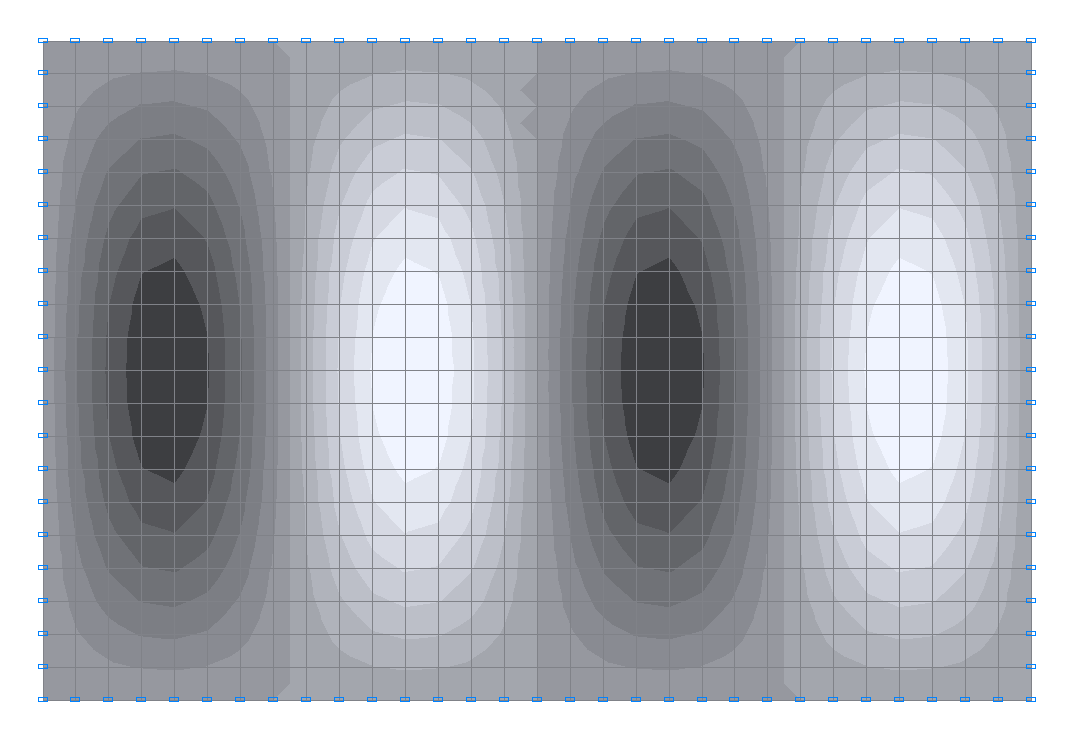

7-th natural oscillation mode

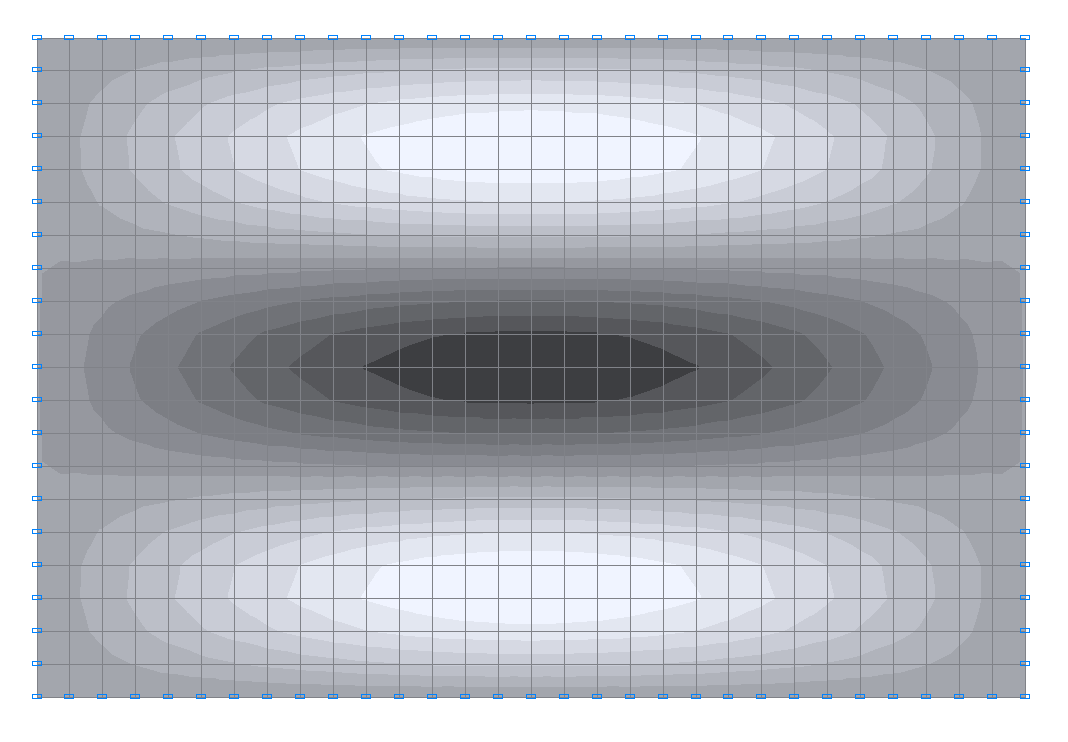

8-th natural oscillation mode
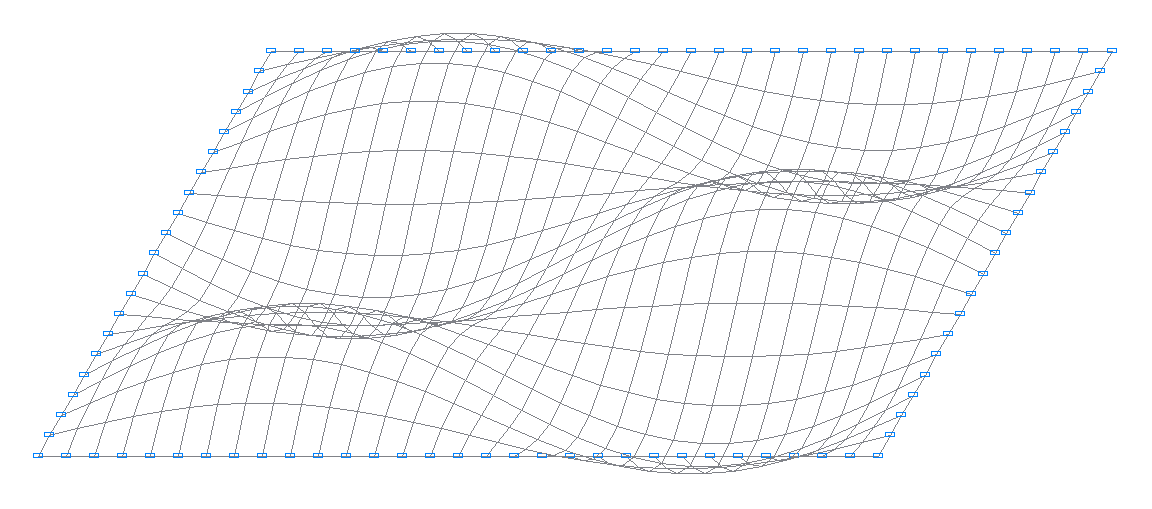


9-th natural oscillation mode
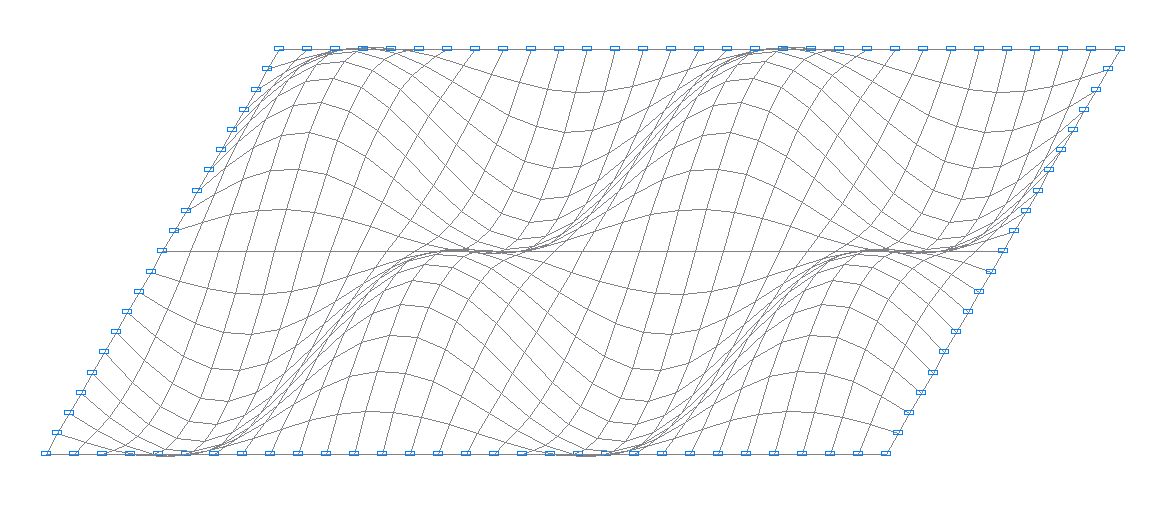
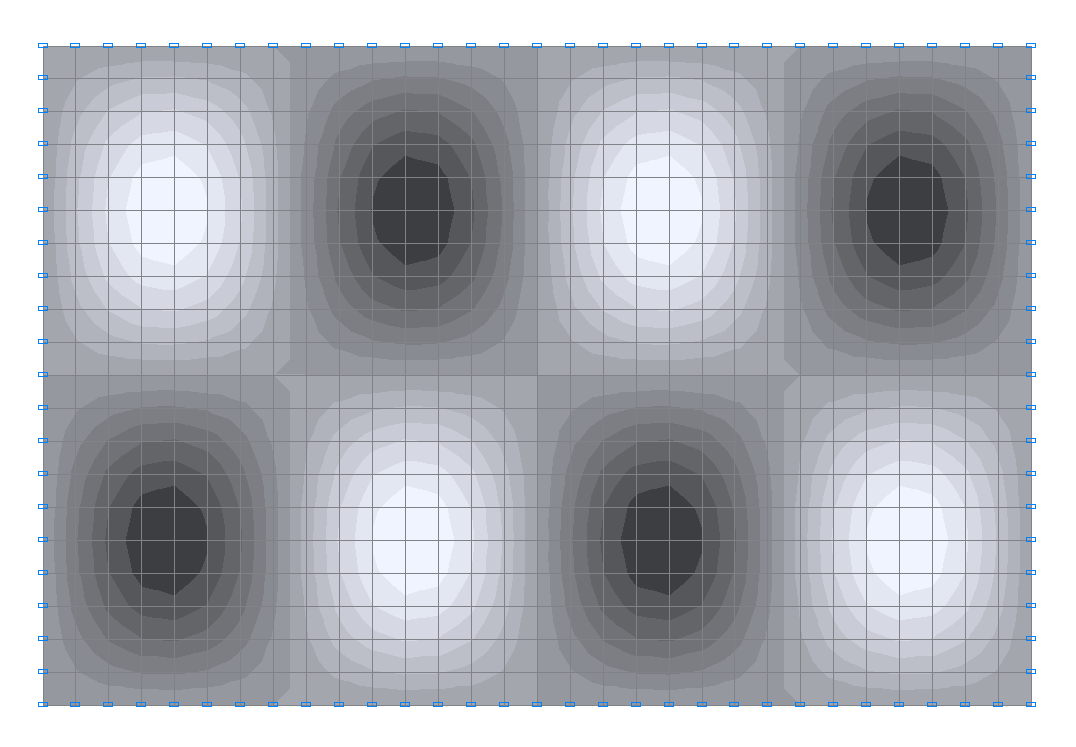

10-th natural oscillation mode
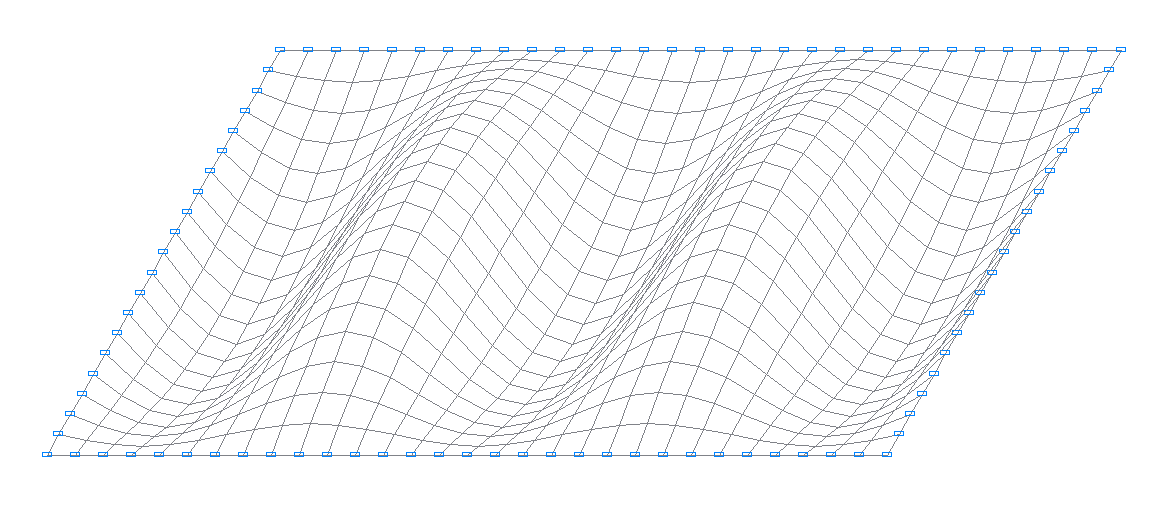
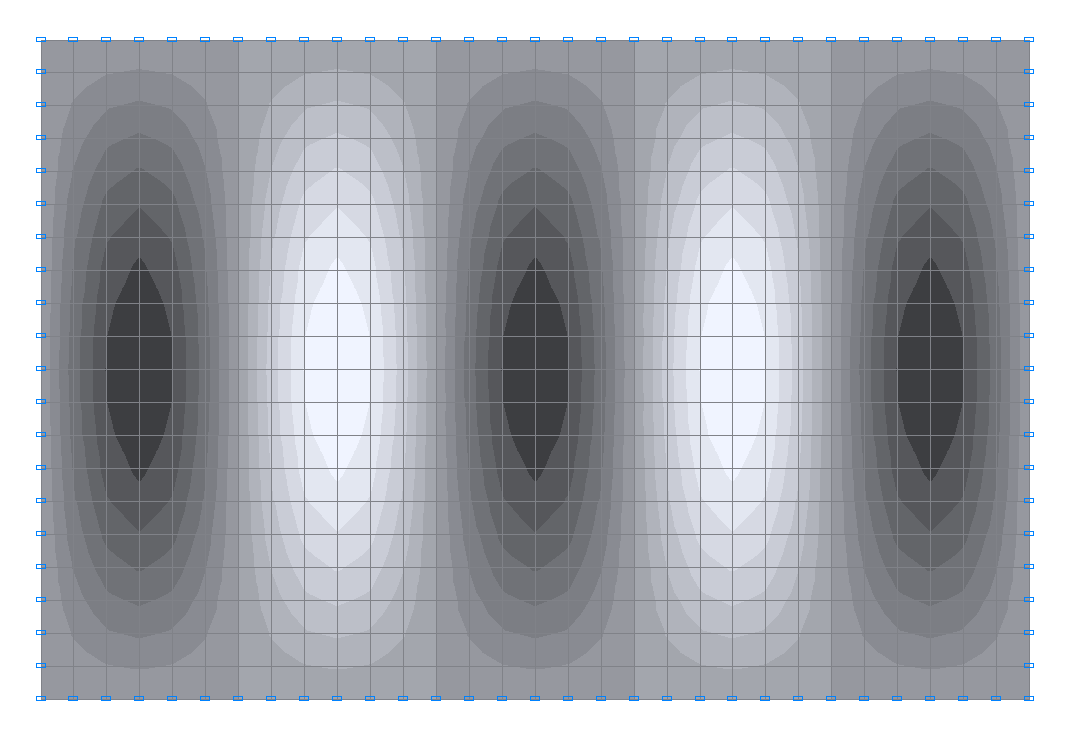

11-th natural oscillation mode
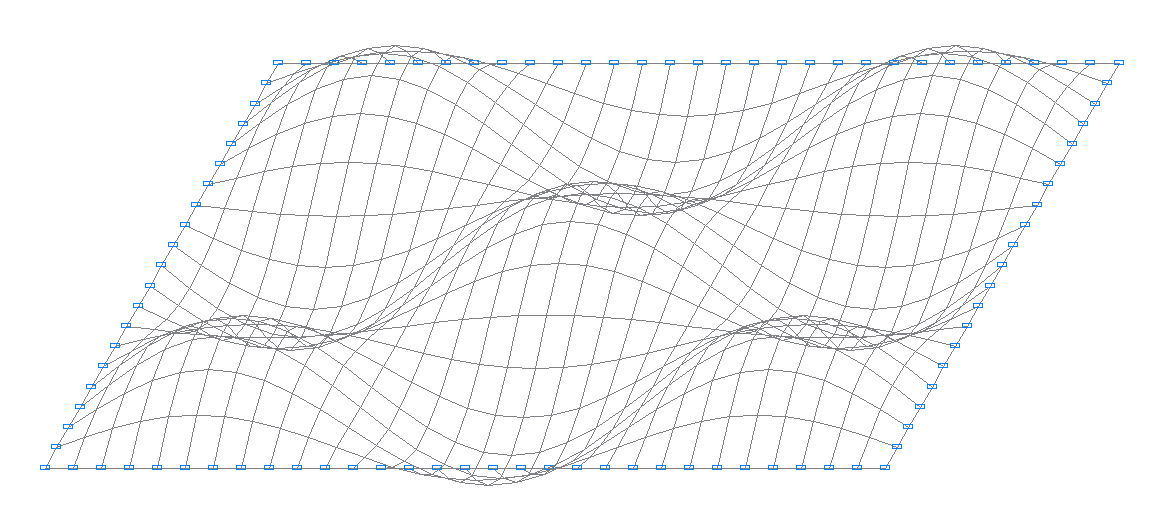
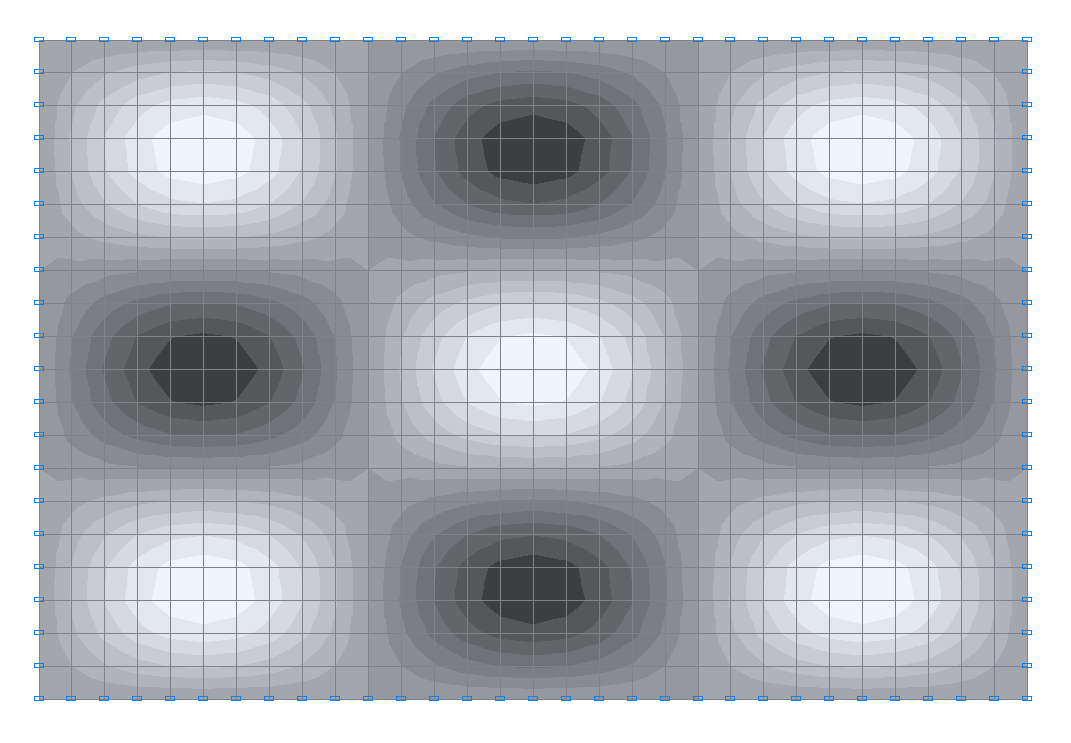

12-th natural oscillation mode
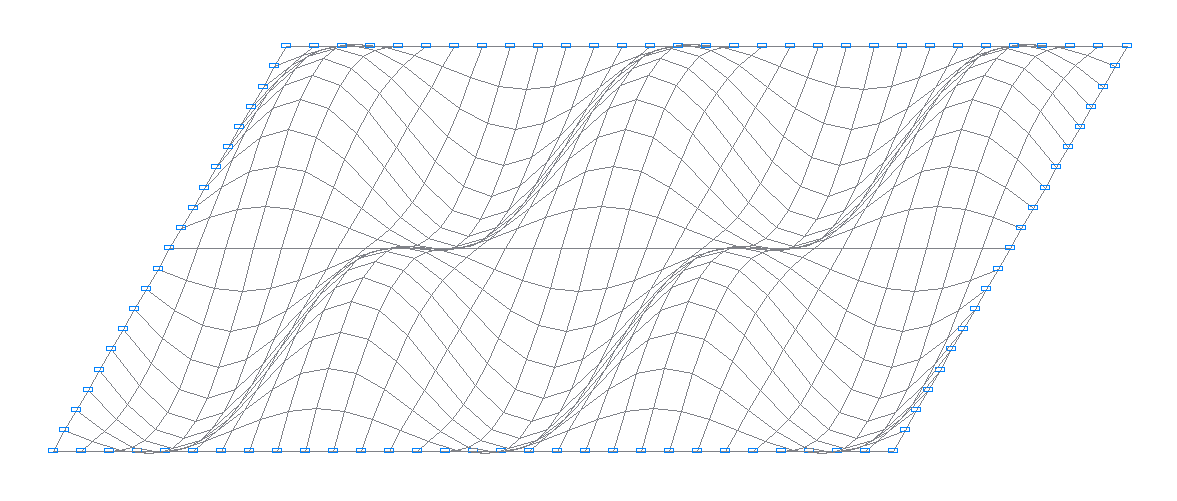
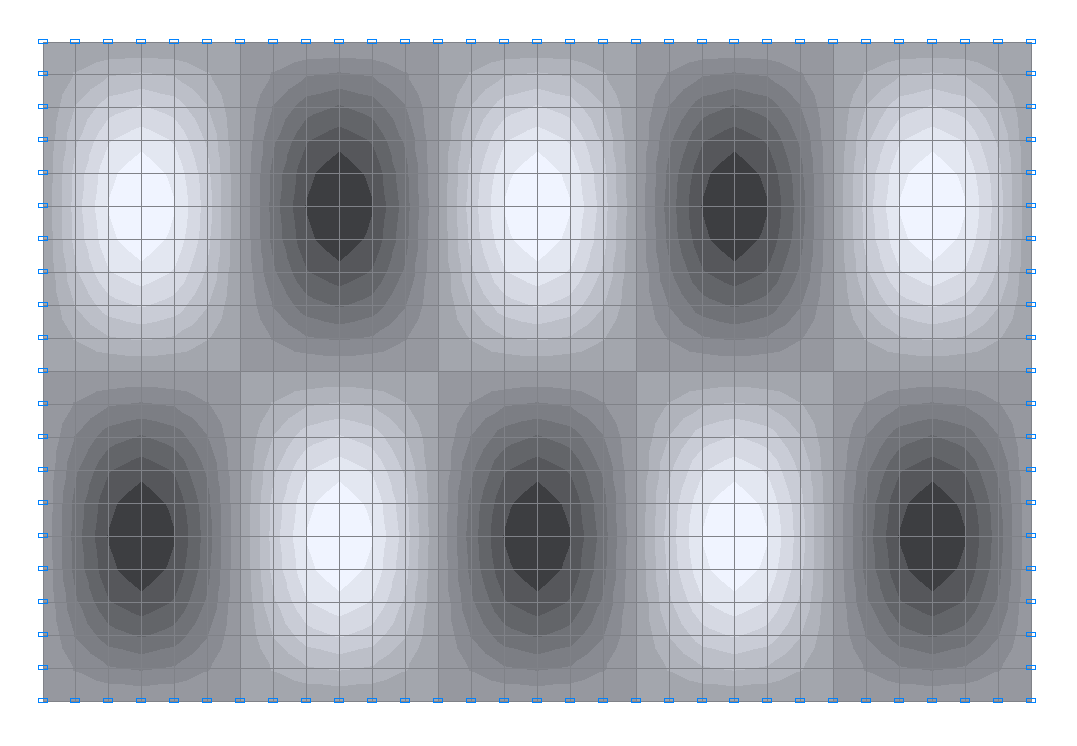

13-th natural oscillation mode
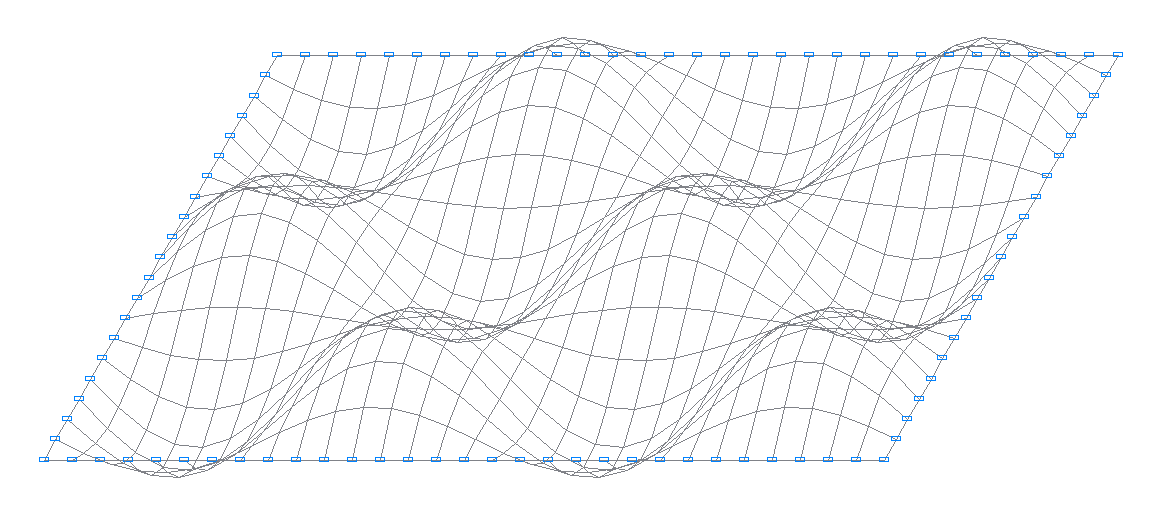
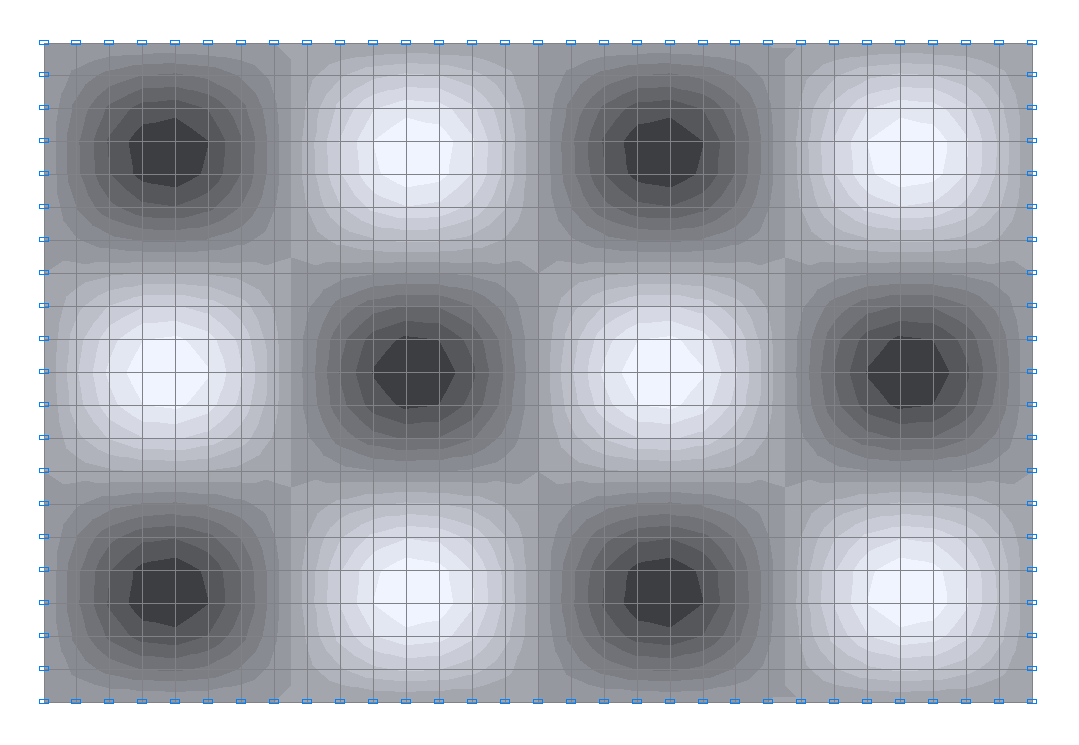

14-th natural oscillation mode

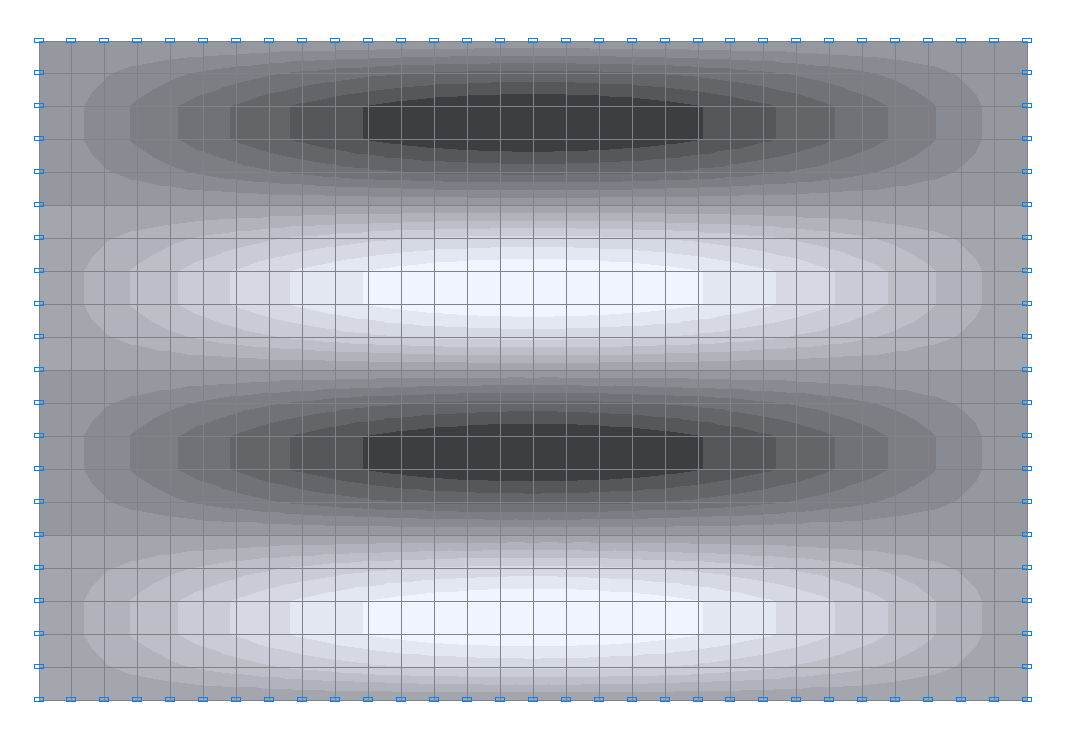

15-th natural oscillation mode
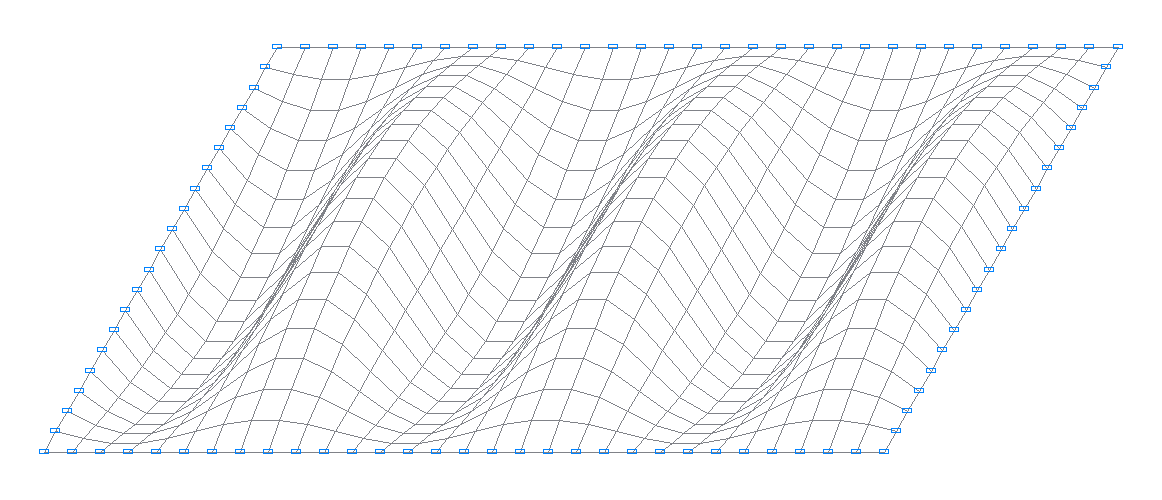
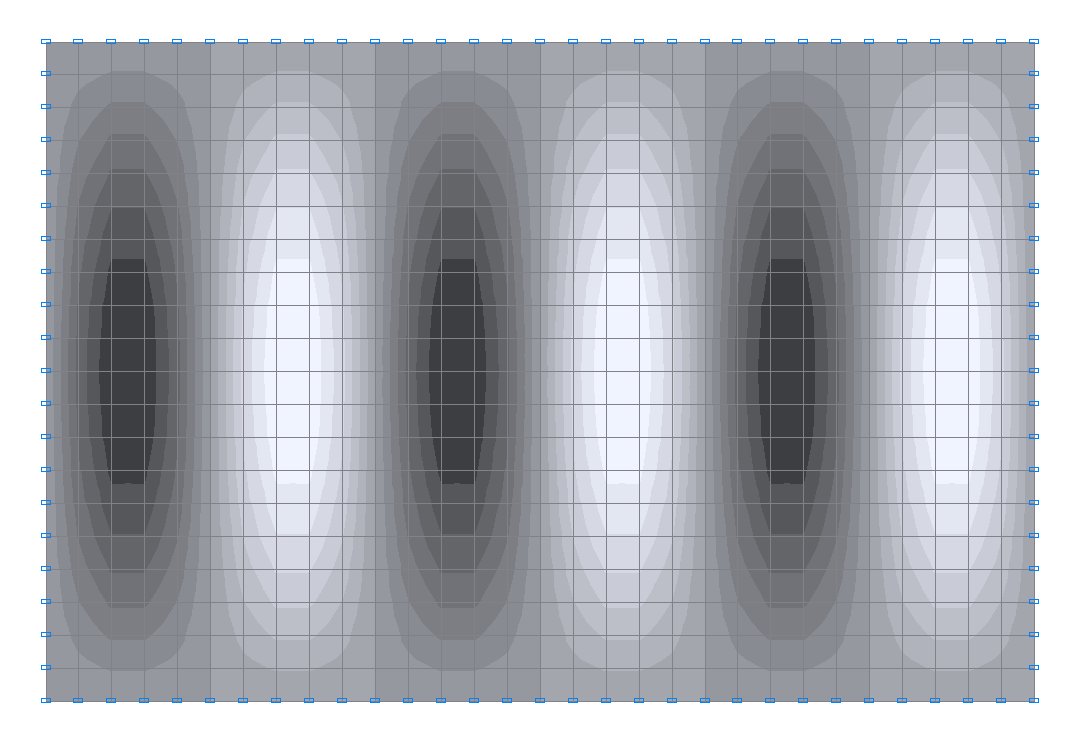

16-th natural oscillation mode
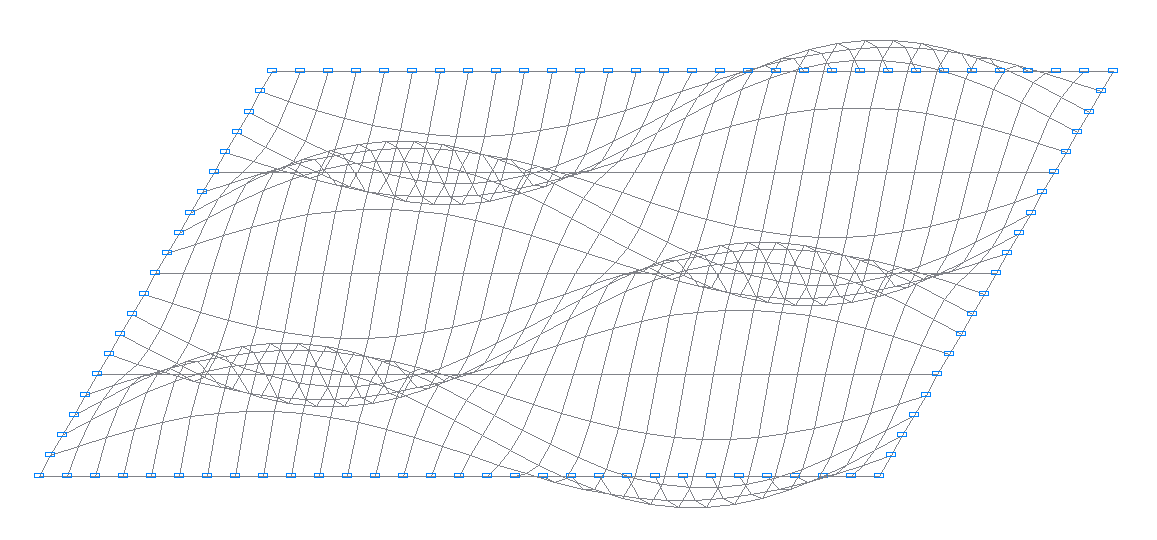
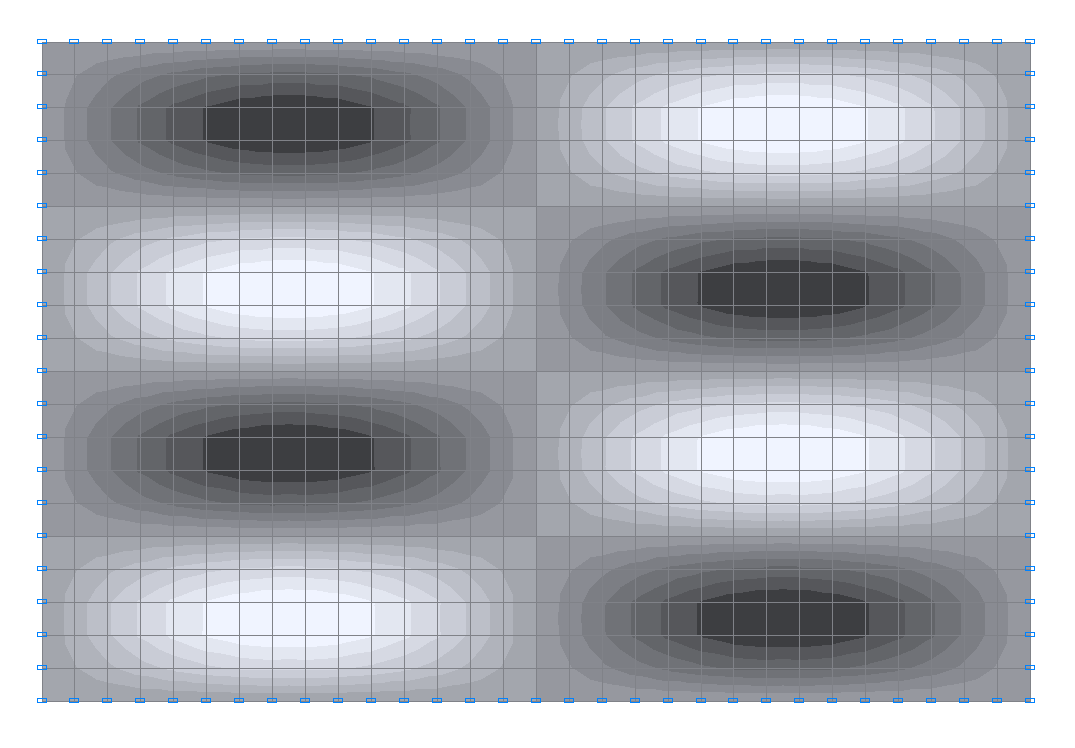

17-th natural oscillation mode
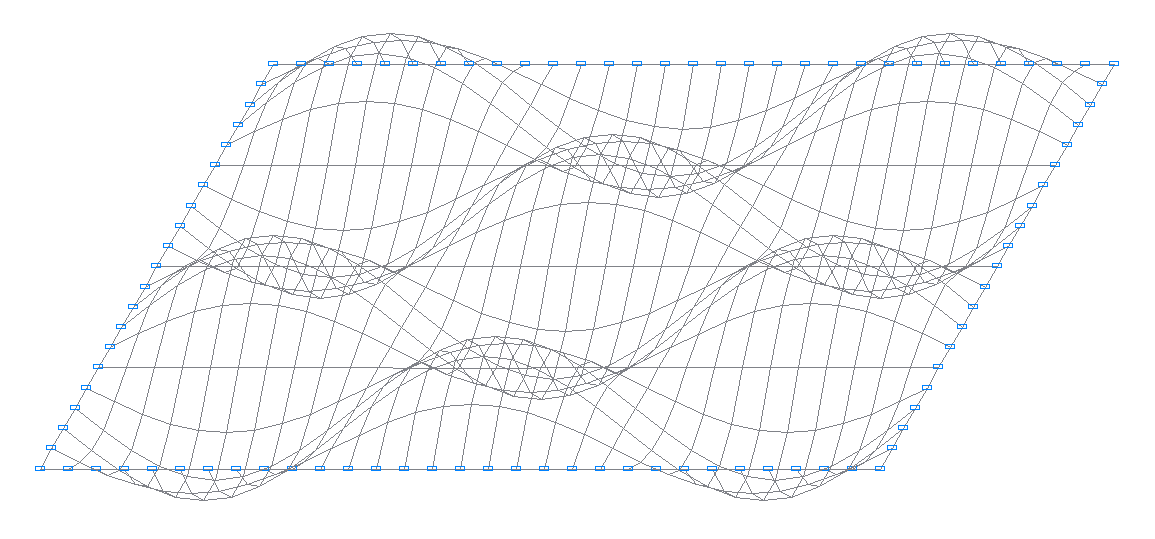
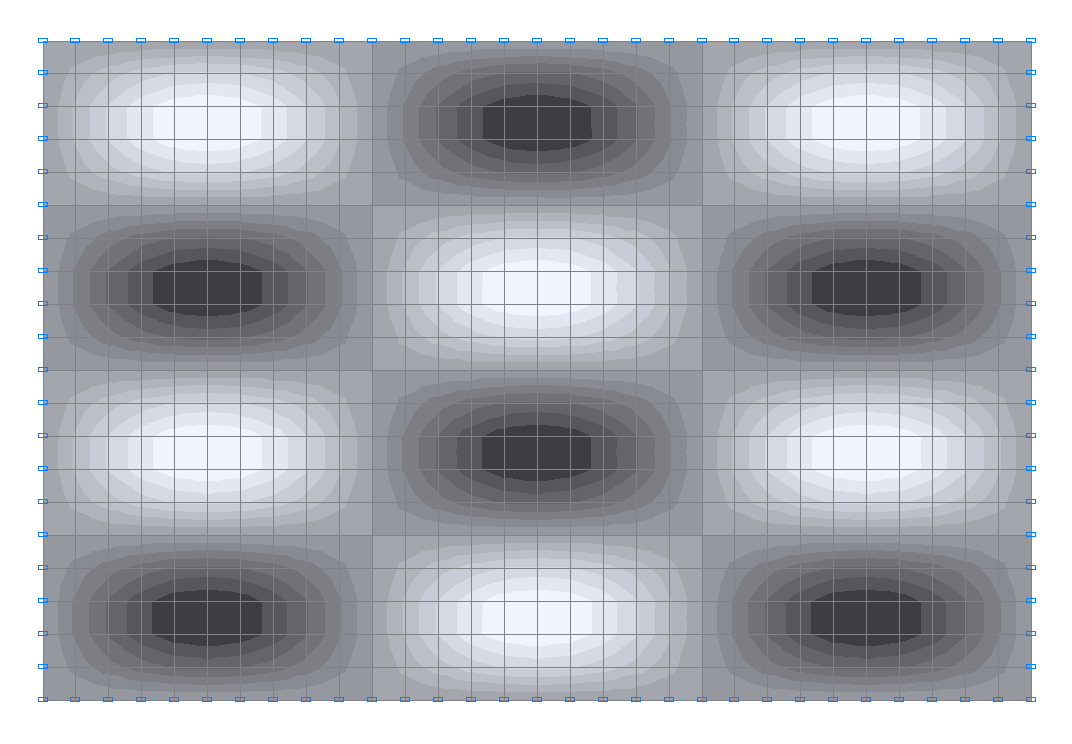
18-th natural oscillation mode
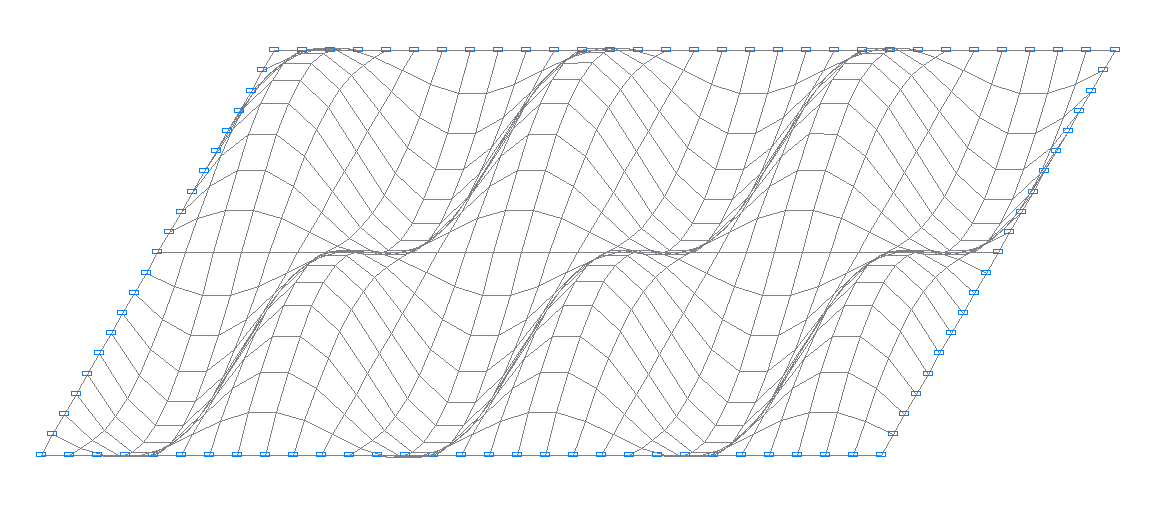
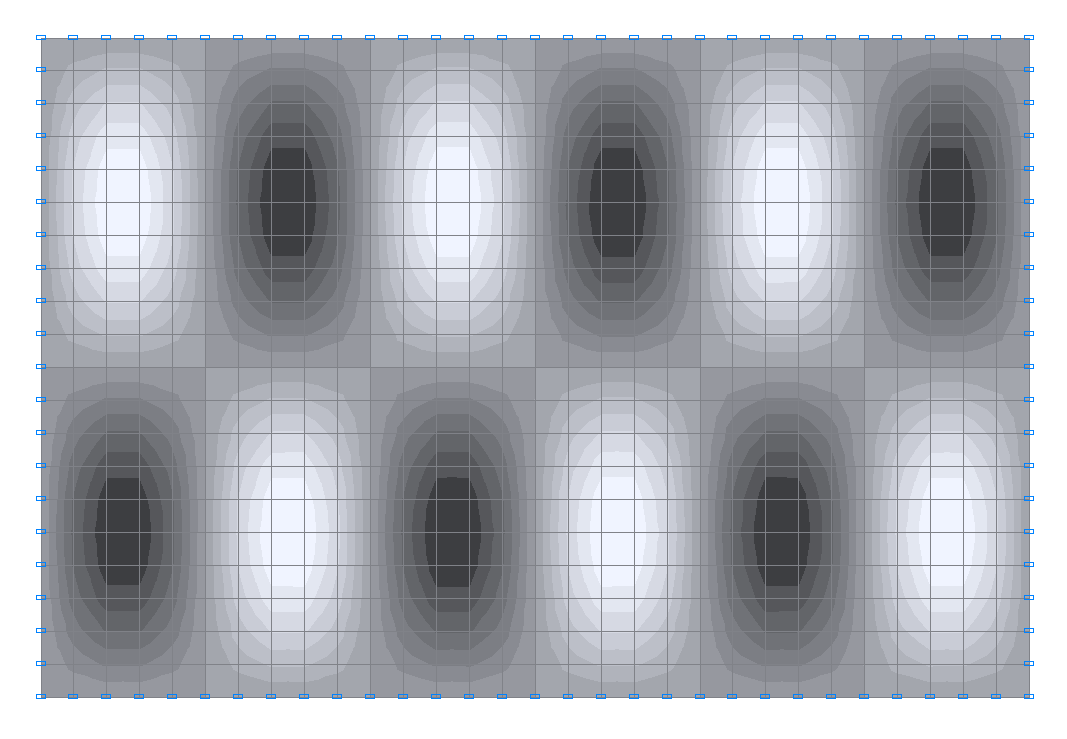

19-th natural oscillation mode
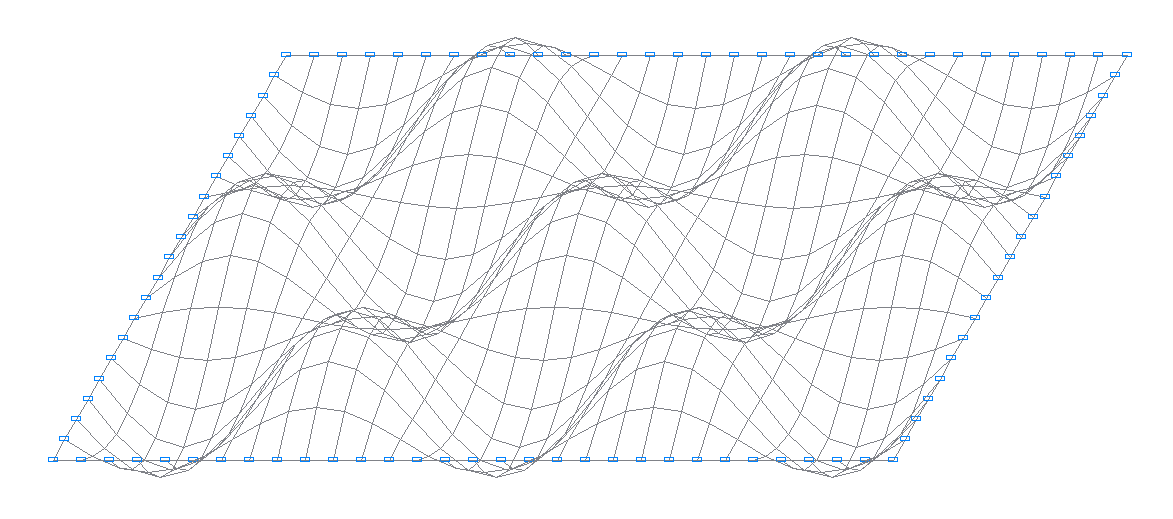
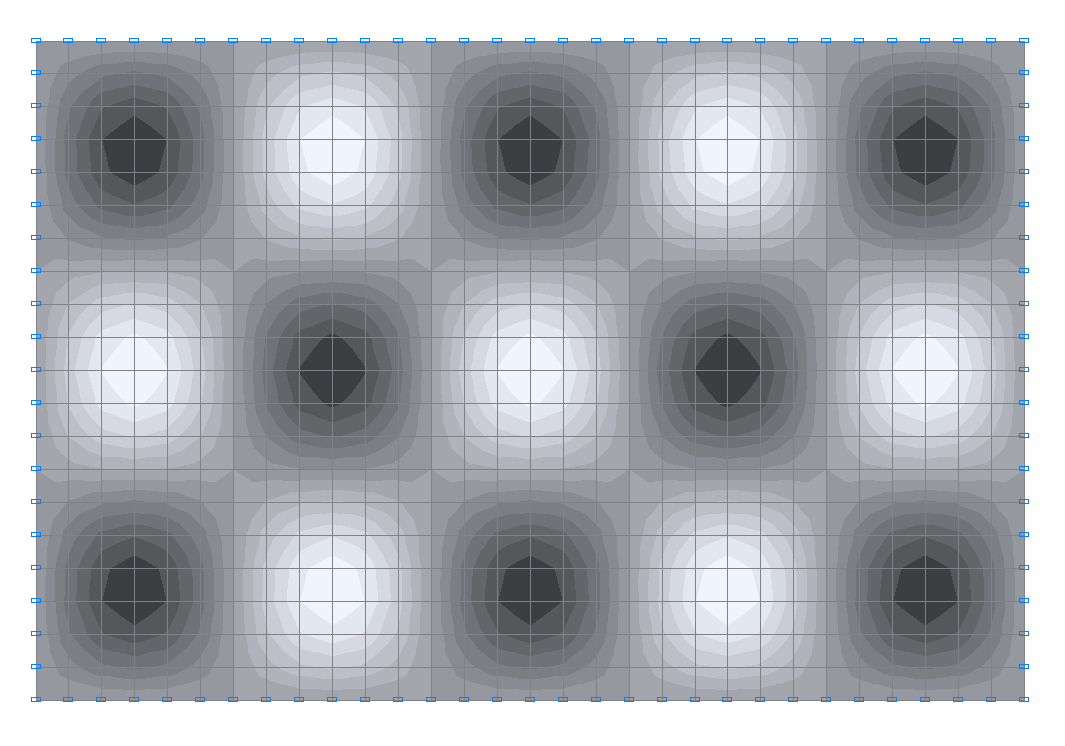

20-th natural oscillation mode
Comparison of solutions:
Natural frequencies ω, rad / s
|
Oscillation mode |
Number of half waves m1, m2 |
Theory |
SCAD |
Deviations, % |
|---|---|---|---|---|
|
1 |
1, 1 |
221.0 |
221.1 |
0.05 |
|
2 |
2, 1 |
425.0 |
425.3 |
0.07 |
|
3 |
1, 2 |
678.0 |
680.3 |
0.34 |
|
4 |
3, 1 |
765.0 |
765.6 |
0.08 |
|
5 |
2, 2 |
884.0 |
885.1 |
0.12 |
|
6 |
3, 2 |
1224.0 |
1226.4 |
0.20 |
|
7 |
4, 1 |
1241.0 |
1242.0 |
0.08 |
|
8 |
1, 3 |
1445.0 |
1445.5 |
0.03 |
|
9 |
2, 3 |
1649.0 |
1651.4 |
0.15 |
|
10 |
4, 2 |
1700.0 |
1704.3 |
0.25 |
|
11 |
5, 1 |
1853.0 |
1854.6 |
0.09 |
|
12 |
3, 3 |
1989.0 |
1994.5 |
0.28 |
|
13 |
5, 2 |
2312.0 |
2318.8 |
0.29 |
|
14 |
4, 3 |
2465.0 |
2474.9 |
0.40 |
|
15 |
1, 4 |
2516.0 |
2516.8 |
0.03 |
|
16 |
6, 1 |
2601.0 |
2603.1 |
0.08 |
|
17 |
2, 4 |
2720.0 |
2724.1 |
0.15 |
|
18 |
3, 4 |
3060.0 |
3069.7 |
0.32 |
|
19 |
6, 2 |
3060.0 |
3069.7 |
0.32 |
|
20 |
5, 3 |
3077.0 |
3092.5 |
0.50 |
Notes: In the analytical solution the natural frequencies ω of the simply supported rectangular plate with the density of the material ρ can be determined according to the following formula:
\[ \omega =\pi^{2}\cdot \left( {\frac{m_{1}^{2}}{a_{2}^{2}}+\frac{m_{2} ^{2}}{a_{2}^{2}}} \right)\cdot \left( {\frac{D}{\rho \cdot h}} \right)^{\frac{1}{2}},\quad where: \quad D=\frac{E\cdot h^{3}}{12\cdot \left( {1-\mu ^{2}} \right)}, \quad m_{1} ,m_{2} =1,2,3, ... \]
