Stability of a Clamped Beam Subjected to a Concentrated Longitudinal Force

Objective: Determination of the critical value of a concentrated longitudinal force acting on a clamped beam corresponding to the moment of its buckling.

Initial data file: CB02_v11.3.spr

Problem formulation: The beam of square cross-section clamped on both ends is subjected to a concentrated longitudinal force P. Determine the critical value of the concentrated longitudinal force P_cr, corresponding to the moment of the buckling of the beam.

References: D. O. Brush and B. O. Almroth, Buckling of Bars, Plates and Shells, New York, McGraw-Hill Co., 1975, p. 22.

Initial data:

E = 3.0·107 Pa	- elastic modulus,
L = 50.0 m	- beam length;
h = 1.0 m	- side of the cross-section of the beam;
P = 1.0·104 N	- initial value of the concentrated longitudinal force.

 

Finite element model: Design  model – plane frame, 10 elements of type 10. The spacing of the finite element mesh along the longitudinal axis (along the X axis of the global coordinate system) is 5.0 m.

Boundary conditions of the clamped (left) end are provided by imposing constraints in the directions of the degrees of freedom X, Z, UY and those of the (right) end with a clamping floating along the beam axis are provided by imposing constraints in the directions of the degrees of freedom Z, UY. The action with the initial value of the concentrated longitudinal force P is specified on the (right) end with a clamping floating along the beam axis. Number of nodes in the design model – 11.

 

Results in SCAD

Design model

 

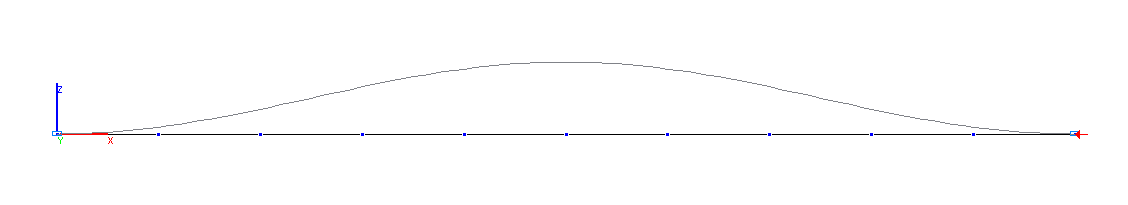
Buckling mode

 

  

Comparison of solutions:

Parameter	Theory	SCAD	Deviation, %
Critical value of the concentrated longitudinal force Pcr, N	39478.4	3.94783∙1000 = =39478.3	0.00

Notes: In the analytical solution the critical value of the concentrated longitudinal force P_cr is determined according to the following formula:

\[ P_{cr} =\frac{4\cdot \pi^{2}\cdot E\cdot I}{L^{2}}, \quad where:\quad I=\frac{h^{4}}{12} \]