Flexible Thread with Supports in One Level Subjected to a Uniformly Distributed Transverse Load

Objective: Determination of the stress-strain state of a flexible thread with supports in one level subjected to a uniformly distributed transverse load q.

Initial data file: NL_CANAT_v21.SPR

Problem formulation: The flexible thread with supports in one level is subjected to the uniformly distributed transverse load q from the self-weight γ. Determine the sag f and the strain σ of the flexible thread.

References: S.P. Fesik, Reference Book on Strength of Materials, 2-nd, Kiev, Budivelnik, 1982, p. 33.

Initial data:

E = 1.0·107 tf/m2	- elastic modulus of the thread;
l = 40.0 м	- length of the span of the flexible thread;
d = 0.04 м	- diameter of the cross-section of the flexible thread;
γ = 8.0 тс/м3	- value of the specific weight of the flexible thread material.

 

Finite element model: Design model – plane frame, 40 elements of type 302. Boundary conditions are provided by imposing constraints in the support nodes of the flexible thread in the directions of the degrees of freedom X, Z. The action of the uniformly distributed transverse load is specified as q = γ·F, where F = π·d2/4. Number of nodes in the design model – 41. The calculation is performed in the geometrically nonlinear formulation by the simple incremental method with the following parameters: loading factor – 0.01, number of steps – 100.

 

Results in SCAD

Design model

 

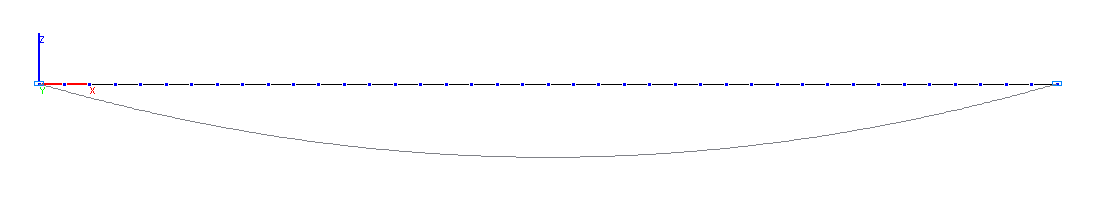
Deformed model

 

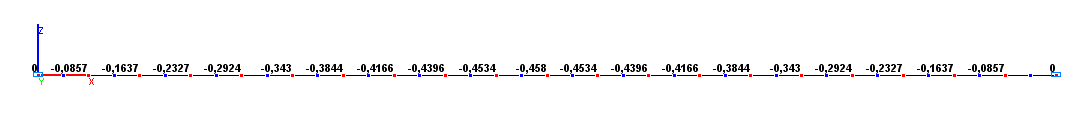
Values of vertical displacements Z (m)

 

Longitudinal force diagram N (tf)

 

 

Comparison of solutions:

Parameter	Theory	SCAD	Deviations, %
Sag f of the flexible thread, m	-0.4579	-0.4580	0.02
Strain σ of the flexible thread, tf/m2	3494.3	4.3761 / (3.1416 · 0.042/4) = 3482.4	0.34

 

Notes: In the analytical solution the sag f and the strain σ of the flexible thread are determined according to the following formulas:

\[ f=\frac{l}{2}\cdot \sqrt[3]{\frac{3\cdot \gamma \cdot l}{8\cdot E}}; \quad \sigma =\frac{\gamma \cdot l^{2}}{8\cdot f}. \]