Sectorial Areas, Static Moments, and Tangential Stresses for an Open-Closed Thin-Walled Section
Aim: To check the accuracy of the determination of sectorial areas ω, static moments with respect to the principal axes of inertia of the section Su, Sv, a sectorial static moment Sω, tangential stresses τu, τv, caused by shear forces, and tangential stresses τω, caused by constrained torsion for an open-closed thin-walled section.
Name of a file with the initial data: Prokic_openclosed.tns
Formulation: Check the accuracy of the calculation of the sectorial areas, static moments, and tangential stresses for an open-closed thin-walled cross-section.
References: Prokić A. Computer program for determination of geometrical properties of thin-walled beams with open-closed section // Computers and Structures, Vol. 74 (2000). – pp. 705 – 715.
Initial data:
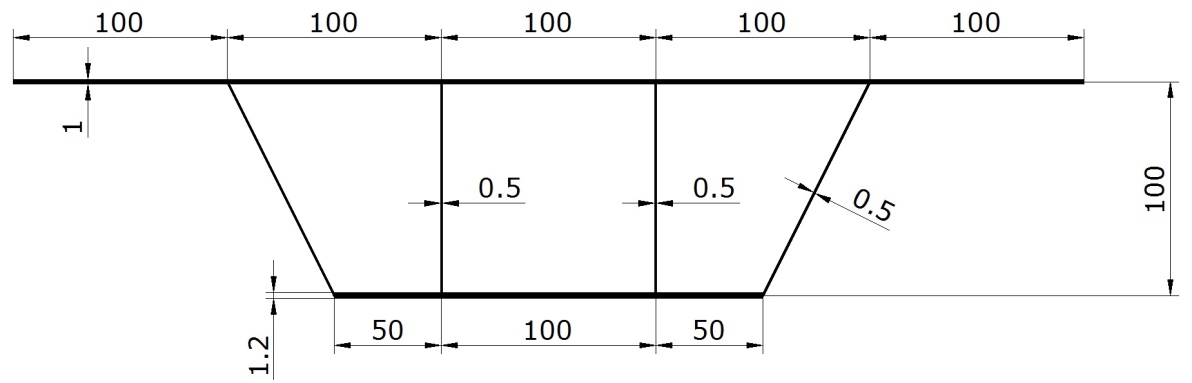
Open-closed thin-walled section with sizes, cm
Results from the source:
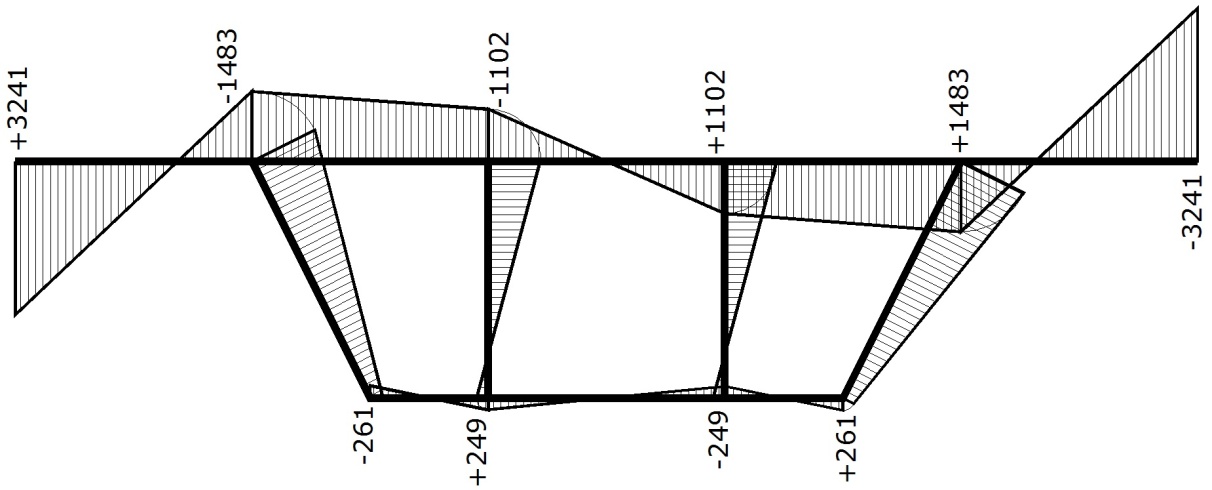
Sectorial area diagram ω, cm2
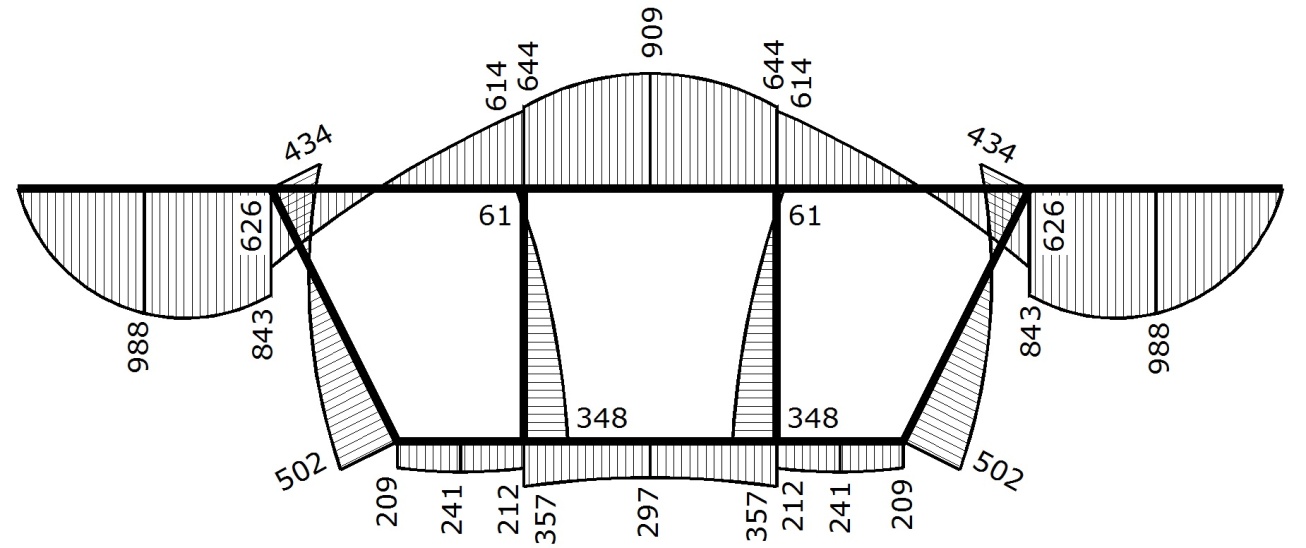
Diagram of tangential stresses related to the constrained torque, τω/Mω×107, 1/cm3
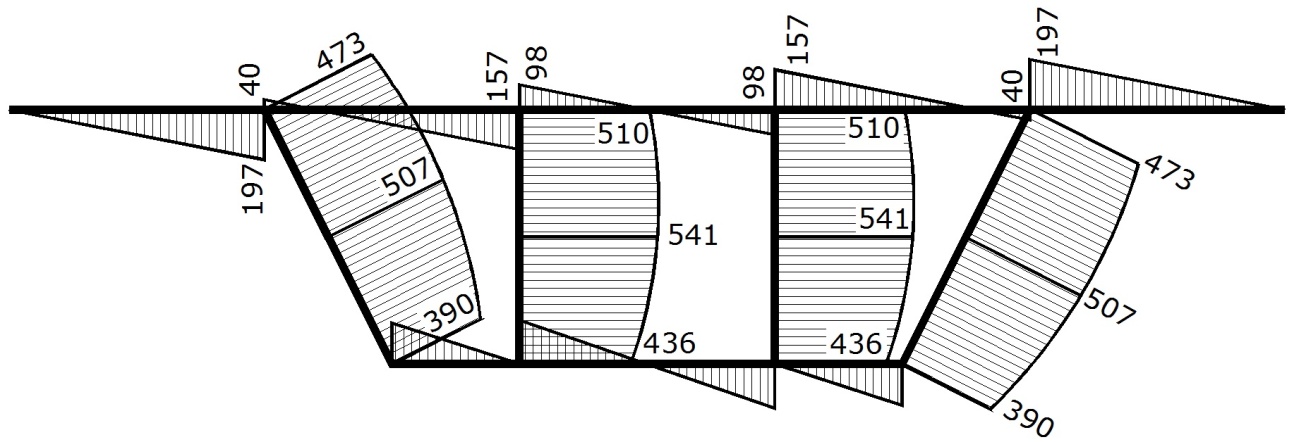
Diagram of tangential stresses related to the shear force, τu/Qu×105, 1/cm2
Results obtained in Tonus:
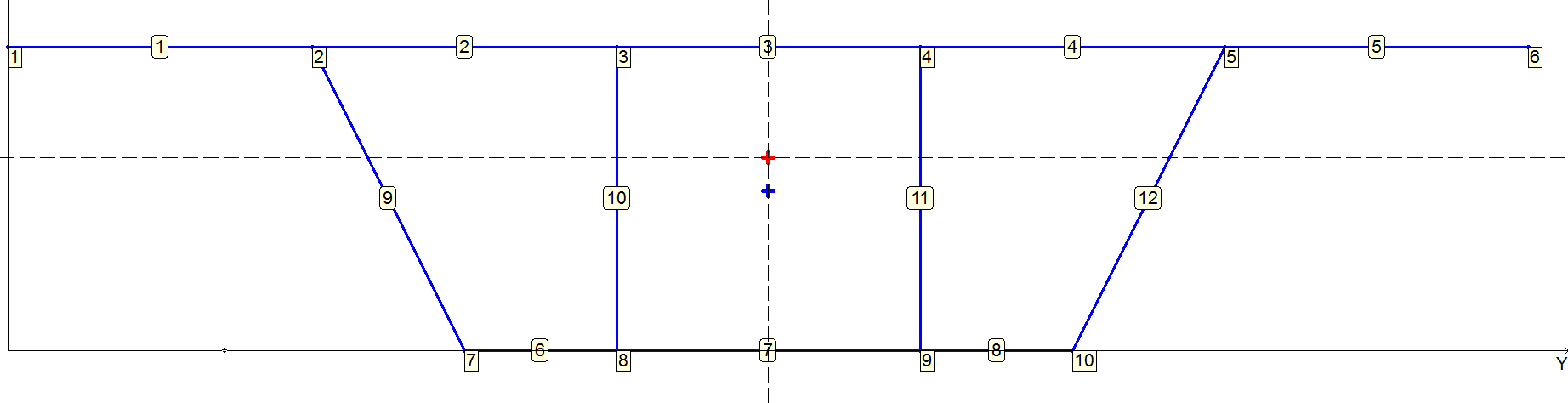
Numbering of vertices and strips, position of the mass center and shear center
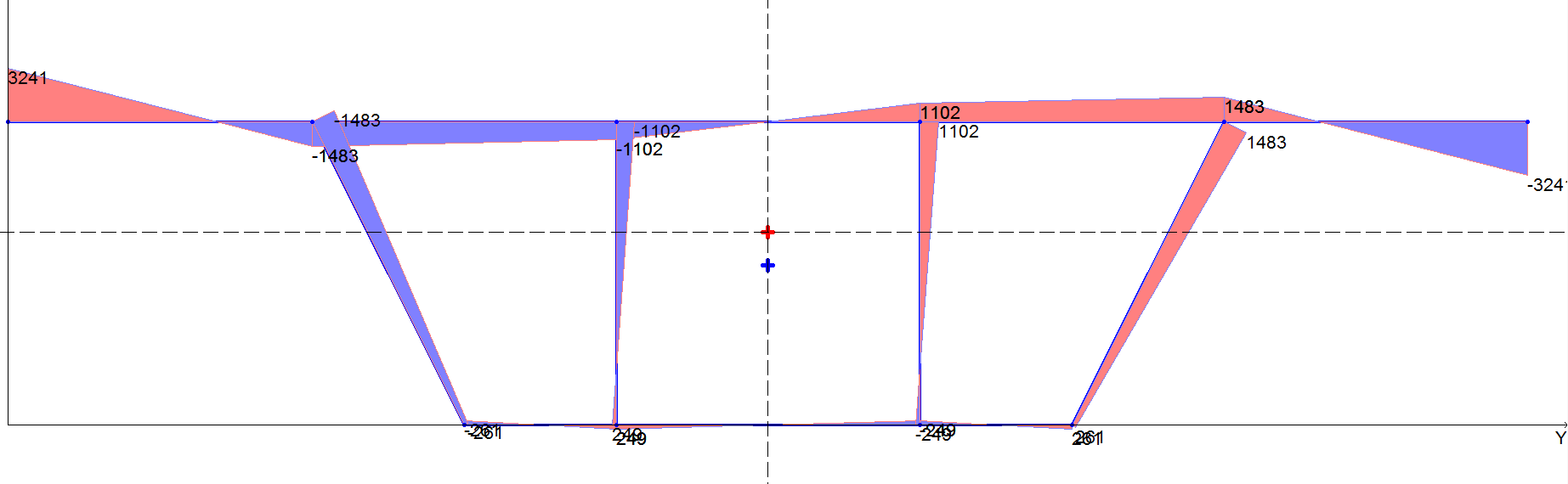
Sectorial area diagram ω, cm2
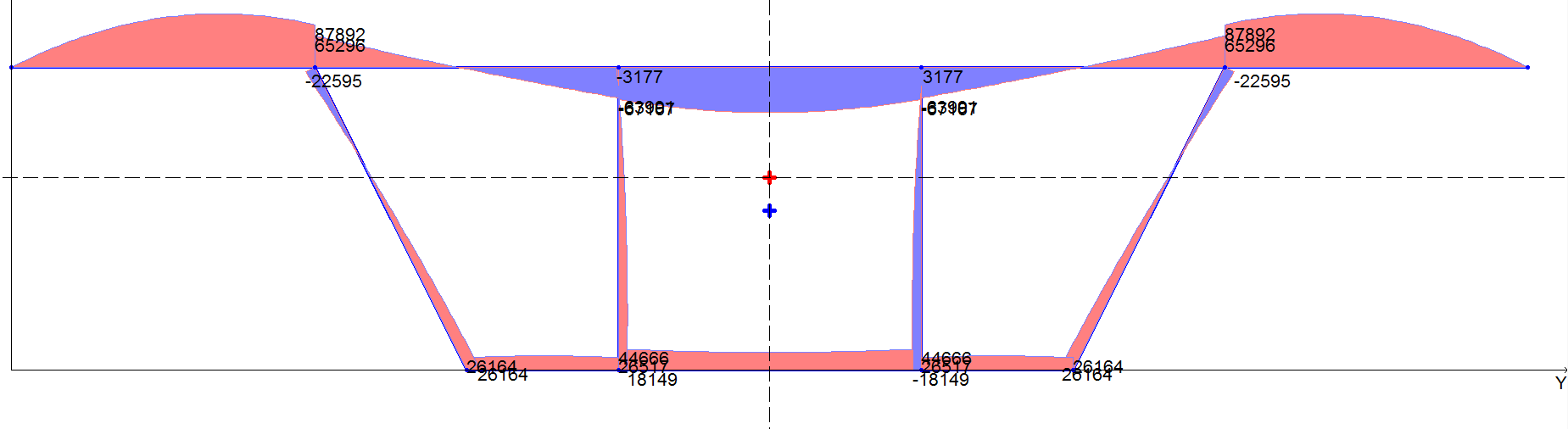
Sectorial static moment diagram Sω, cm4
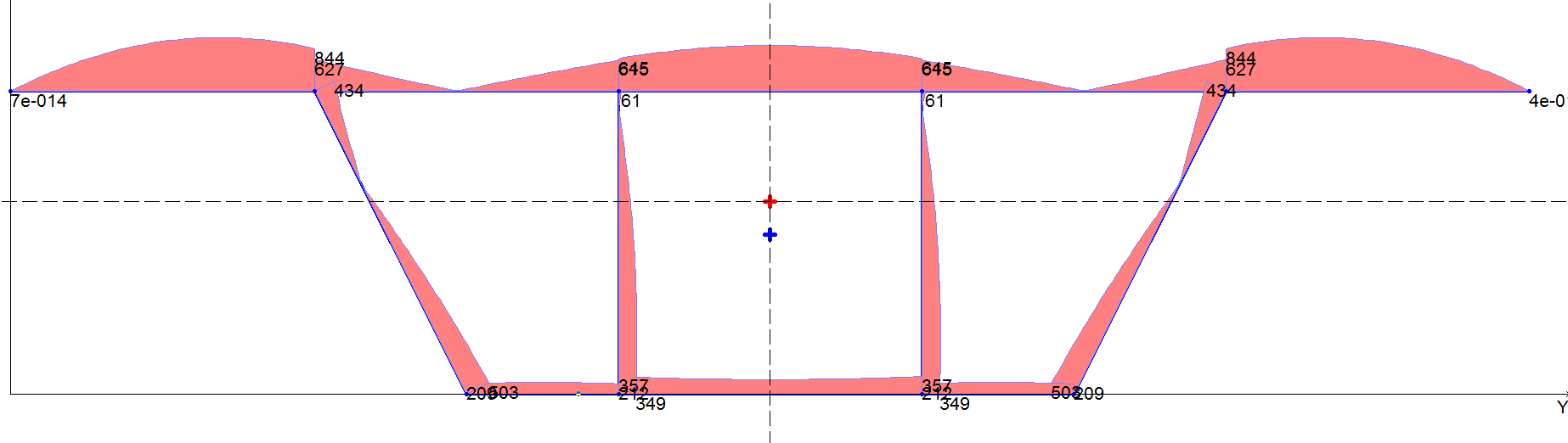
Diagram of the tangential stress module τω for the value of the constrained torque Mω = 107, kNcm
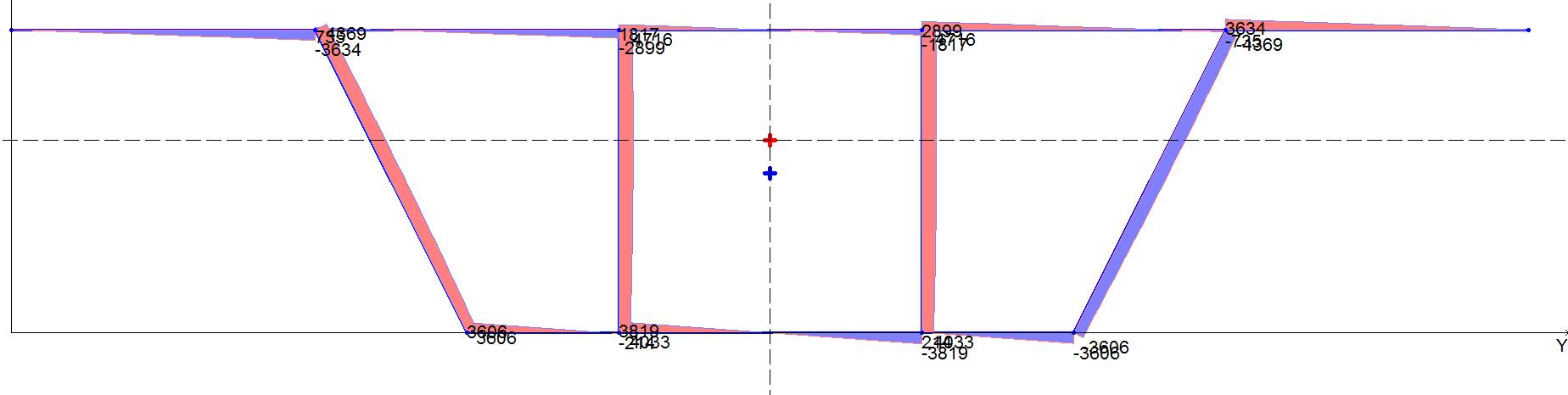
Static moment diagram Sv, cm3
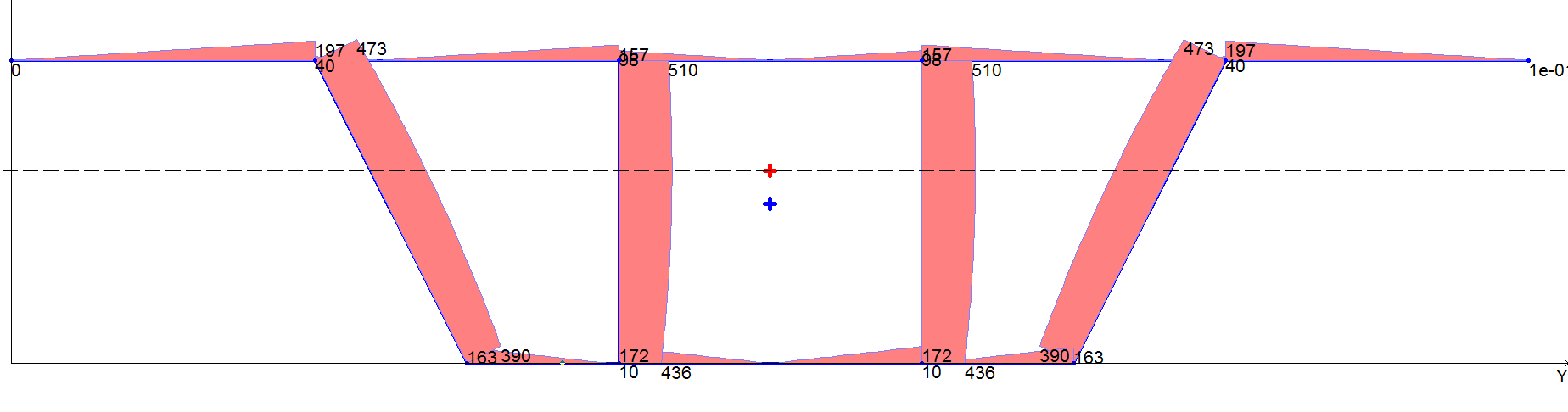
Diagram of the tangential stress module τu for the value of the shear force Qu = 105, kN
Comparison of results:
|
Element number |
Vertex number |
Sectorial static moment Sω, cm4 |
Static moment Sv, cm3 |
||||
|---|---|---|---|---|---|---|---|
|
Source* |
TONUS |
Deviation, % |
Source** |
TONUS |
Deviation, % |
||
|
1 |
1 |
0 |
0 |
0 |
0 |
0 |
0 |
|
1 |
2 |
87776 |
87892 |
0,13 |
3643 |
3634 |
0,25 |
|
2 |
2 |
65181 |
65296 |
0,18 |
740 |
741 |
0,14 |
|
2 |
3 |
63932 |
64036 |
0,16 |
2903 |
2899 |
0,14 |
|
3 |
3 |
67055 |
67159 |
0,16 |
1812 |
1817 |
0,28 |
|
6 |
7 |
26114 |
26164 |
0,19 |
3595 |
3606 |
0,3 |
|
6 |
8 |
26489 |
26517 |
0,11 |
– |
10 |
– |
|
7 |
8 |
44606 |
44666 |
0,13 |
3816 |
3819 |
0,08 |
|
9 |
2 |
22595 |
22595 |
0 |
4373 |
4369 |
0,09 |
|
9 |
7 |
26135 |
26164 |
0,11 |
3606 |
3606 |
0 |
|
10 |
3 |
3176 |
3177 |
0,03 |
4715 |
4716 |
0,02 |
|
10 |
8 |
18117 |
18149 |
0,15 |
4031 |
4033 |
0,05 |
|
Notes: * The value of the static sectorial moment Sω was calculated using the value τω/Mω, obtained from the source as (Iω = 1041229484 cm6): Sω = τωIωt / Mω; ** The value of the static moment Sv was calculated using the value τu/Qu, obtained from the source as (Iv = 1849016 cm4): Sv = τuIvt / Qu. |
|||||||
|
Element number |
Vertex number |
Tangential stress τω, kN/cm2 (at Mω = 107, kNcm) |
Tangential stress τu, kN/cm2 (at Qu = 105, kN) |
||||
|---|---|---|---|---|---|---|---|
|
Source |
TONUS |
Deviation, % |
Source |
TONUS |
Deviation, % |
||
|
1 |
1 |
0 |
0 |
0 |
0 |
0 |
0 |
|
1 |
2 |
843 |
844 |
0,12 |
197 |
197 |
0 |
|
2 |
2 |
626 |
627 |
0,16 |
40 |
40 |
0 |
|
2 |
3 |
614 |
615 |
0,16 |
157 |
157 |
0 |
|
3 |
3 |
644 |
645 |
0,16 |
98 |
98 |
0 |
|
6 |
7 |
209 |
209 |
0 |
162 |
163 |
0,6 |
|
6 |
8 |
212 |
212 |
0 |
– |
10 |
0 |
|
7 |
8 |
357 |
357 |
0 |
172 |
172 |
0 |
|
9 |
2 |
434 |
434 |
0 |
473 |
473 |
0 |
|
9 |
7 |
502 |
503 |
0,20 |
390 |
390 |
0 |
|
10 |
3 |
61 |
61 |
0 |
510 |
510 |
0 |
|
10 |
8 |
348 |
349 |
0,29 |
436 |
436 |
0 |
|
Vertex number |
Sectorial area, cm2 |
||
|---|---|---|---|
|
Source |
TONUS |
Deviation, % |
|
|
1 |
+3241 |
+3241 |
0 |
|
2 |
–1483 |
–1483 |
0 |
|
3 |
–1102 |
–1102 |
0 |
|
7 |
–261 |
–261 |
0 |
|
8 |
+249 |
+249 |
0 |