Sectorial Properties of a Compound Thin-walled Section with a Compound Profile


Aim: To check the accuracy of the geometric properties calculation for a compound thin-walled section.
Name of a file with the initial data: Beilin.tns
Formulation: Check the accuracy of the geometric properties calculation for a thin-walled compound cross-section.
References: E.A.Beilin, Version of the Unified Torsion Theory for Bars with Open, Closed, and Partially Closed Profiles. In: Researches on Mechanics of Structures and Materials. Topical collected works of universities, Leningrad Civil Engineering Institute, 1991, pp. 57-74.
Initial data:
| d = 1 cm | – thickness of strips; |
| b = 10 cm | – height of the section. |
Results Obtained in Tonus:
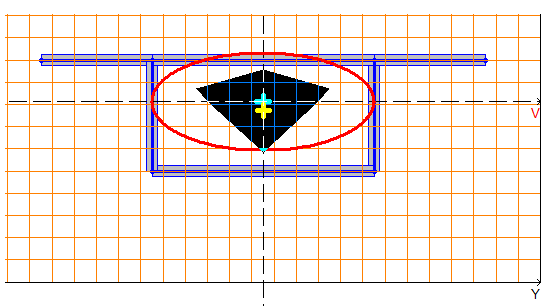
Design model, coordinate and principal axes, center of mass, ellipse of inertia, core of the section
Comparison of results:
|
Parameter |
Theory |
TONUS |
Deviation, % |
|
Cross-sectional area, A cm2 |
80 |
80 |
0 |
|
Conventional shear area along the principal U-axis, Av,y cm2 |
20 |
20 |
0 |
|
Conventional shear area along the principal V-axis, Av,z cm2 |
60 |
60 |
0 |
|
Torsional moment of inertia, It cm4 |
2693,333 |
2693,333 |
0 |
|
Sectorial moment of inertia, Iw cm6 |
9722,222 |
9722,222 |
0 |
|
Y-coordinate of the shear center, yb cm |
25 |
25 |
0 |
|
Z-coordinate of the shear center, zb cm |
15,417 |
15,417 |
0 |
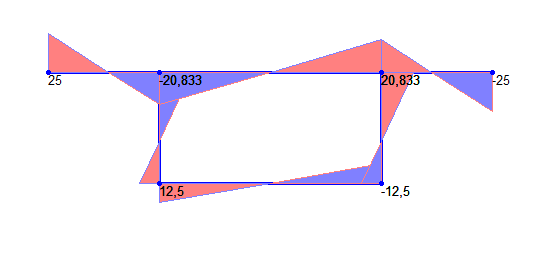
Comparison of the sectorial coordinates:
|
|
|
|
Numbers of Vertices |
Sectorial Coordinate Diagrams |
|
Number of a point |
Sectorial coordinate |
||
|---|---|---|---|
|
|
Theory |
TONUS |
Deviation, % |
|
1 |
25 |
25 |
0 |
|
2 |
-25 |
-25 |
0 |
|
3 |
20.833 |
20.833 |
0 |
|
4 |
-12.5 |
-12.5 |
0 |
|
5 |
12.5 |
12.5 |
0 |
|
6 |
-20.833 |
-20.833 |
0 |